Motion in a Plane - Result Question 49
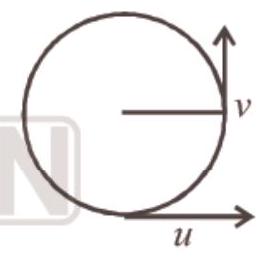
52. A stone is tied to a string of length
[2004]
(a)
(b)
(c)
(d)
Show Answer
Answer:
Correct Answer: 52. (b)
Solution:
- (b)
or,
or,