SATHEE: Gravitation - Result Question 3

Gravitation - Result Question 3

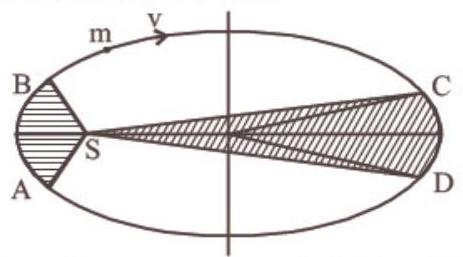
3. The figure shows elliptical orbit of a planet $m$ about the sun $S$ . The shaded area SCD is twice the shaded area $S A B$ . If $t_{1}$ is the time for the planet to move from $C$ to $D$ and $t_{2}$ is the time to move from $A$ to $B$ then :

[2009]

(a) $t_{1} = 4 t_{2}$

(b) $t_{1} = 2 t_{2}$

(d) $t_{1} > t_{2}$

Show Answer

Answer:

Correct Answer: 3. (b)

Solution:

(b) According to Kepler’s law, the areal velocity of a planet around the sun always remains constant.

$S C D : A_{1} - t_{1}$ (areal velocity constant)

$S A B : A_{2} - t_{2}$

$\frac{A_{1}}{t_{1}} = \frac{A_{2}}{t_{2}}$ ,

$t_{1} = t_{2} \cdot \frac{A_{1}}{A_{2}} (.$ given $. A_{1} = 2 A_{2})$

$\begin{aligned} = t_{2} \cdot \frac{2 A_{2}}{A_{2}} \\ ∴ t_{1} = 2 t_{2} \end{aligned}$

The area covered by radius vector in $d t$ seconds $=$

$\frac{1}{2} r^{2} d θ$

$\begin{matrix} Area velocity = \frac{1}{2} r^{2} \frac{d θ}{d t} = \frac{1}{2} r^{2} ω (∵ ω \frac{d θ}{d t}) \\ = \frac{1}{2} r v (∵ v = ω r) \end{matrix}$

It follows that speed of the planet is maximum when it is closest to the sun and is minimum when the planet is farthest from the sun.

Gravitation - Result Question 3

3. The figure shows elliptical orbit of a planet $m$ about the sun $S$ . The shaded area SCD is twice the shaded area $S A B$ . If $t_{1}$ is the time for the planet to move from $C$ to $D$ and $t_{2}$ is the time to move from $A$ to $B$ then :

Answer:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Gravitation - Result Question 3

3. The figure shows elliptical orbit of a planet m about the sun S. The shaded area SCD is twice the shaded area SAB. If t1 is the time for the planet to move from C to D and t2 is the time to move from A to B then :

Answer:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu

3. The figure shows elliptical orbit of a planet $m$ about the sun $S$ . The shaded area SCD is twice the shaded area $S A B$ . If $t_{1}$ is the time for the planet to move from $C$ to $D$ and $t_{2}$ is the time to move from $A$ to $B$ then :