Electric Charges and Fields - Result Question 5
5. Two pith balls carrying equal charges are suspended from a common point by strings of equal length. The equilibrium separation between them is
clamped at half the height. The equilibrium separation between the balls now become
[2013]
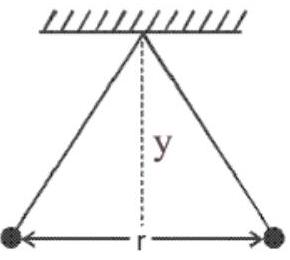
(a)
(c)
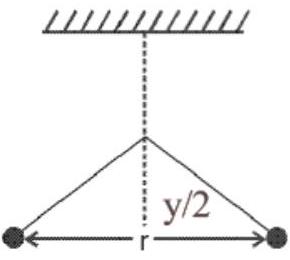
(d)
Show Answer
Answer:
Correct Answer: 5. (a)
Solution:
- (a)
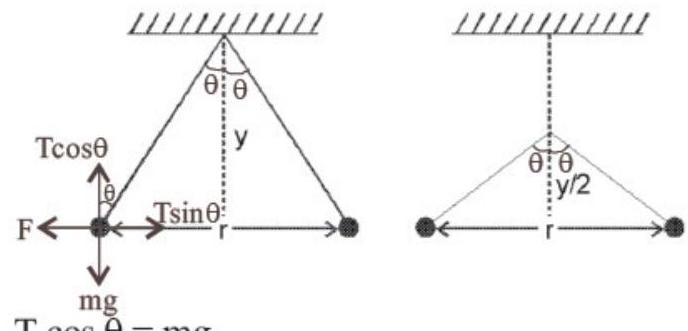
From figure,
When string is clamped at half the height
Dividing the above two equations, we have






