Alternating Current - Result Question 27
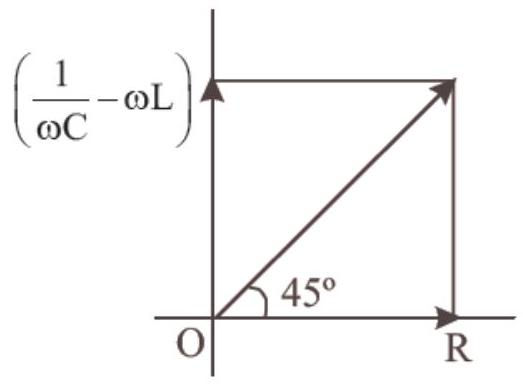
27. In a circuit,
[2005]
(a)
(b)
(c)
(d)
Show Answer
Answer:
Correct Answer: 27. (d)
Solution:
- (d) From figure,