Chapter 09 Ray Optics and Optical Instruments
Multiple Choice Questions (MCQs)
1. A ray of light incident at an angle $\theta$ on a refracting face of a prism emerges from the other face normally. If the angle of the prism is $5^{\circ}$ and the prism is made of a material of refractive index 1.5, the angle of incidence is
(a) $7.5^{\circ}$
(b) $5^{\circ}$
(c) $15^{\circ}$
(d) $2.5^{\circ}$
Show Answer
Thinking Process
The ray refractive by first surface falls normally on second surface, in order to emerges from the other face normally.
Answer
(a) Since, deviation $\delta=(\mu-1) A=(1.5-1) \times 5^{\circ}=2.5^{\circ}$
By geometry, angle of refraction by first surface is $5^{\circ}$.
But $\delta=\theta-r$, so, we have, $2.5^{\circ}=\theta-5^{\circ}$ on solving $\theta=7.5^{\circ}$.
2. A short pulse of white light is incident from air to a glass slab at normal incidence. After travelling through the slab, the first colour to emerge is $\newline$
(a) blue $\newline$
(b) green $\newline$
(c) violet $\newline$
(d) red $\newline$
Show Answer
Thinking Process
When light ray goes from one medium to other medium, the frequency of light remains unchanged.
Answer
(d) Since $v \propto \lambda$, the light of red colour is of highest wavelength and therefore of highest speed. Therefore, after travelling through the slab, the red colour emerge first.
3. An object approaches a convergent lens from the left of the lens with a uniform speed $5 m / s$ and stops at the focus. The image
(a) moves away from the lens with an uniform speed $5 m / s$
(b) moves away from the lens with an uniform acceleration
(c) moves away from the lens with a non-uniform acceleration
(d) moves towards the lens with a non-uniform acceleration
Show Answer
Thinking Process
This problem has link with the formation of image when object is at different positions.
Answer
(c) When an object approaches a convergent lens from the left of the lens with a uniform speed of $5 m / s$, the image away from the lens with a non-uniform acceleration.
4. A passenger in an aeroplane shall
(a) never see a rainbow
(b) may see a primary and a secondary rainbow as concentric circles
(c) may see a primary and a secondary rainbow as concentric arcs
(d) shall never see a secondary rainbow
Show Answer
Answer
(b) A passenger in an aeroplane may see a primary and a secondary rainbow like concentric circles.
5. You are given four sources of light each one providing a light of a single colour - red, blue, green and yellow. Suppose the angle of refraction for a beam of yellow light corresponding to a particular angle of incidence at the interface of two media is $90^{\circ}$. Which of the following statements is correct if the source of yellow light is replaced with that of other lights without changing the angle of incidence?
(a) The beam of red light would undergo total internal reflection
(b) The beam of red light would bend towards normal while it gets refracted through the second medium
(c) The beam of blue light would undergo total internal reflection
(d) The beam of green light would bend away from the normal as it gets refracted through the second medium
Show Answer
Thinking Process
This problem is based on the critical angle of total internal reflection.
Answer
(c) According to VIBGYOR, among all given sources of light, the blue light have smallest wavelength.According to Cauchy relationship, smaller the wavelength higher the refractive index and consequently smaller the critical angle.
So, corresponding to blue colour, the critical angle is least which facilitates total internal reflection for the beam of blue light. The beam of green light would also undergo total internal reflection.
6. The radius of curvature of the curved surface of a plano-convex lens is $20 cm$. If the refractive index of the material of the lens be 1.5, it will
(a) act as a convex lens only for the objects that lie on its curved side
(b) act as a concave lens for the objects that lie on its curved side
(c) act as a convex lens irrespective of the side on which the object lies
(d) act as a concave lens irrespective of side on which the object lies
Show Answer
Thinking Process
By lens maker’s formula for plano-convex lens, focal length is given by $f=\frac{R}{\mu-1}$. This is always positive for $\mu>1$ or optically denser medium of material of lens placed in air.
Answer
(c) Here, $R=20 cm, \mu=1.5$, on substituting the values in $f=\frac{R}{\mu-1}=\frac{20}{1.5-1}=40 cm$ of converging nature as $f>0$. Therefore, lens act as a convex lens irrespective of the side on which the object lies.
7. The phenomena involved in the reflection of radiowaves by ionosphere is similar to
(a) reflection of light by a plane mirror
(b) total internal reflection of light in air during a mirage
(c) dispersion of light by water molecules during the formation of a rainbow
(d) scattering of light by the particles of air
Show Answer
Answer
(b) The phenomenon involved in the reflection of radiowaves by ionosphere is similar to total internal reflection of light in air during a mirage i.e., angle of incidence is greater than critical angle.
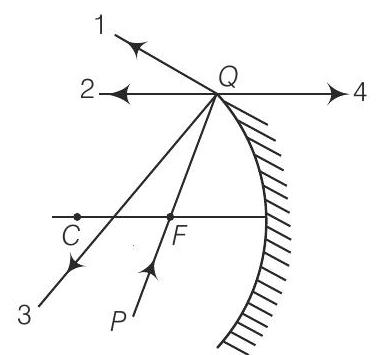
8. The direction of ray of light incident on a concave mirror is shown by $P Q$ while directions in which the ray would travel after reflection is shown by four rays marked 1, 2, 3 and 4 (figure). Which of the four rays correctly shows the direction of reflected ray?
(a) 1 $\newline$
(b) 2 $\newline$
(c) 3 $\newline$
(d) 4 $\newline$
Show Answer
Answer
(b) The $P Q$ ray of light passes through focus $F$ and incident on the concave mirror, after reflection, should become parallel to the principal axis and shown by ray-2 in the figure.
9. The optical density of turpentine is higher than that of water while its mass density is lower. Figure shows a layer of turpentine floating over water in a container. For which one of the four rays incident on turpentine in figure, the path shown is correct?
(a) 1 $\newline$
(b) 2 $\newline$
(c) 3 $\newline$
(d) 4 $\newline$

Show Answer
Thinking Process
When a light ray goes from (optically) rarer medium to (optically) denser medium, then it bends towards the normal i.e., i>r and vice-versa.
Answer
(b) Here, light ray goes from (optically) rarer medium air to optically denser terpentine, then it bends towards the normal i.e., $i>r$ whereas when it goes from to optically denser medium terpentine to rarer medium water. then it bends away the normal i.e., $i<r$.
10. $A$ car is moving with at a constant speed of $60 km h^{-1}$ on a straight road. Looking at the rear view mirror, the driver finds that the car following him is at a distance of $100 m$ and is approaching with a speed of $5 kmh^{-1}$.
In order to keep track of the car in the rear, the driver begins to glance alternatively at the rear and side mirror of his car after every $2 s$ till the other car overtakes. If the two cars were maintaining their speeds, which of the following statement (s) is/are correct?
(a) The speed of the car in the rear is $65 km h^{-1}$
(b) In the side mirror, the car in the rear would appear to approach with a speed of $5 kmh^{-1}$ to the driver of the leading car
(c) In the rear view mirror, the speed of the approaching car would appear to decrease as the distance between the cars decreases
(d) In the side mirror, the speed of the approaching car would appear to increase as the distance between the cars decreases
Show Answer
Thinking Process
The image formed by convex mirror does not depend on the relative position of object w.r.t. mirror.
Answer
(d) The speed of the image of the car would appear to increase as the distance between the cars decreases.
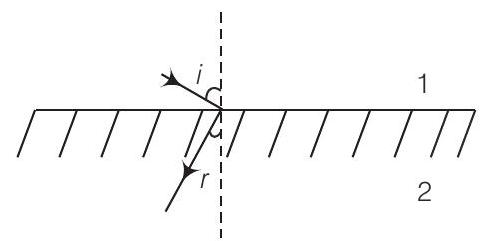
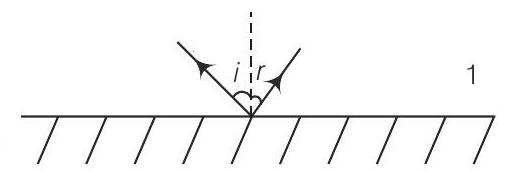
11. There are certain material developed in laboratories which have a negative refractive index figure. A ray incident from air (Medium 1) into such a medium (Medium 2) shall follow a path given by
(a)
(c)
2 (b)
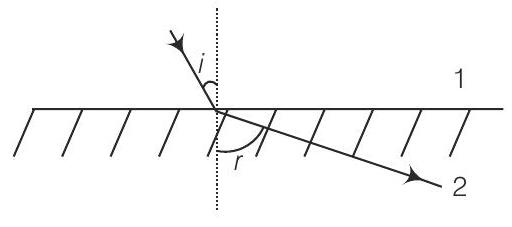
(d)
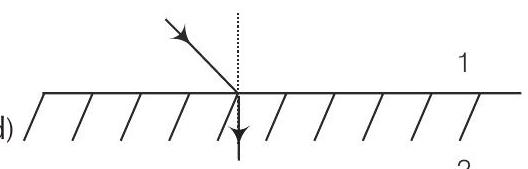
Show Answer
Answer
(a) The negative refractive index metamaterials are those in which incident ray from air (Medium 1) to them refract or bend differently to that of positive refractive index medium.
Multiple Choice Questions (More Than One Options)
12. Consider an extended object immersed in water contained in a plane trough. When seen from close to the edge of the trough the object looks distorted because
(a) the apparent depth of the points close to the edge are nearer the surface of the water compared to the points away from the edge
(b) the angle subtended by the image of the object at the eye is smaller than the actual angle subtended by the object in air
(c) some of the points of the object far away from the edge may not be visible because of total internal reflection
(d) water in a trough acts as a lens and magnifies the object
Show Answer
Thinking Process
This problem is based on the phenomenon of reflection when it goes from denser to rare medium
Answer
$(a, b, c)$
When immersed object is seen from close to the edge of the trough the object looks distorted because the apparent depth of the points close to the edge are nearer the surface of the water compared to the points away from the edge.
The angle subtended by the image of the object at the eye is smaller than the actual angle subtended by the object in air and some of the points of the object far away from the edge may not be visible because of total internal reflection.
13. A rectangular block of glass $A B C D$ has a refractive index 1.6. A pin is placed midway on the face $A B$ figure. When observed from the face $A D$, the pin shall
(a) appear to be near $A$
(b) appear to be near $D$
(c) appear to be at the centre of $A D$
(d) not be seen at all
Show Answer
Thinking Process
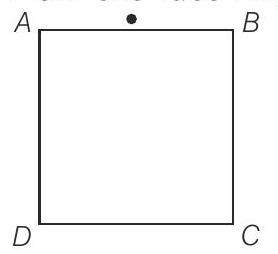
This problem is associated with the phenomenon of total internal reflection.
Answer
(d) For $\mu=1.6$, the critical angle, $\mu=1 / \sin C$, we have $C=38.7^{\circ}$, when viewed from $A D$, as long as angle of incidence on $A D$ of the ray emanating from pin is greater than the critical angle, the light suffers from total internal reflection and cannot be seen through $A D$.
14. Between the primary and secondary rainbows, there is a dark band known as Alexandar’s dark band. This is because
(a) light scattered into this region interfere destructively
(b) there is no light scattered into this region
(c) light is absorbed in this region
(d) angle made at the eye by the scattered rays with respect to the incident light of the sun lies between approximately $42^{\circ}$ and $50^{\circ}$
Show Answer
Answer
$(a, d)$
Alexandar’s dark band lies between the primary and secondary rainbows, forms due to light scattered into this region interfere destructively.
Since, primary rainbows subtends an angle nearly $41^{\circ}$ to $42^{\circ}$ at observer’s eye, whereas, secondary rainbows subtends an angle nearly $51^{\circ}$ to $54^{\circ}$ at observer’s eye w.r.t. incident light ray.
So, the scattered rays with respect to the incident light of the sun lies between approximately $42^{\circ}$ and $50^{\circ}$.
15. A magnifying glass is used, as the object to be viewed can be brought closer to the eye than the normal near point. This results in
(a) a larger angle to be subtended by the object at the eye and hence, viewed in greater detail
(b) the formation of a virtual erect image
(c) increase in the field of view
(d) infinite magnification at the near point
Thinking Process
The problems is about the case when an object is placed between optical centre and focus of the convergent (magnifying) lens.
Show Answer
Answer
$(a, b)$
A magnifying glass is used, as the object to be viewed can be brought closer to the eye than the normal near point. This results in a larger angle to be subtended by the object at the eye and hence, viewed in greater detail. Morever, the formation of a virtual erect and enlarged image, takes place.
16. An astronomical refractive telescope has an objective of focal length $20 m$ and an eyepiece of focal length $2 cm$.
(a) The length of the telescope tube is $20.02 m$
(b) The magnification is 1000
(c) The image formed is inverted
(d) An objective of a larger aperture will increase the brightness and reduce chromatic aberration of the image
Show Answer
Thinking Process
The magnifying power $m$ is the ratio of the angle $\beta$ subtended at the eye by the final image to the angle $\boldsymbol{\alpha}$ which the object subtends at the lens or the eye. Hence, in normal adjustment
$$ m \approx \frac{\beta}{\alpha} \approx \frac{h}{f_{e}} \frac{f_{o}}{h}=\frac{f_{o}}{f_{e}} $$
In this case, the length of the telescope tube is $f_{o}+f_{e}$.
Answer
$(a, b, c)$
The length of the telescope tube is $f_{o}+f_{e}=20+(0.02)=20.02 m$
Also,
$m=20 / 0.02=1000$
Also, the image formed is inverted.
Very Short Answer Type Questions
17. Will the focal length of a lens for red light be more, same or less than that for blue light?
Show Answer
Answer
As the refractive index for red is less than that for blue, parallel beams of light incident on a lens will be bent more towards the axis for blue light compared to red.
In other words, $\mu_{b}>\mu_{r}$
By lens maker’s formula
$$ \frac{1}{f}=(n_{21}-1) \frac{1}{R_1}-\frac{1}{R_2} $$
Therefore, $f_{b}<f_{r}$.
Thus, the focal length for blue light will be smaller than that for red.
18. $ \text{The near vision of an average person is 25 cm}$. To view an object with an angular magnification of 10 , what should be the power of the microscope?
Show Answer
Answer
The least distance of distinct vision of an average person (i.e., $D$ ) is $25 cm$, in order to view an object with magnification 10 ,
Here, $v=D=25 cm$ and $u=f$
But the magnification $m=v / u=D / f$
$$ \begin{aligned} & m=\frac{D}{f} \\ & f=\frac{D}{m}=\frac{25}{10}=2.5=0.025 m \\ & P=\frac{1}{0.025}=40 D \end{aligned} $$
This is the required power of lens
19. An unsymmetrical double convex thin lens forms the image of a point object on its axis. Will the position of the image change if the lens is reversed?
Show Answer
Thinking Process
One lens have unique focal length irrespective of its face or geometry taken for use.
Answer
No, the reversibility of the lens maker’s equation.
20. Three immiscible liquids of densities $d_1>d_2>d_3$ and refractive indices $\mu_1>\mu_2>\mu_3$ are put in a beaker. The height of each liquid column is $\frac{h}{3}$. A dot is made at the bottom of the beaker. For near normal vision, find the apparent depth of the dot.
Show Answer
Thinking Process
The image formed by first medium act as an object for second medium .
Answer
Let the apparent depth be $O_1$ for the object seen from $m_2$, then
$$ O_1=\frac{\mu_2}{\mu_1} \frac{h}{3} $$
Ray Optics and Optical Instruments
Since, apparent depth $=$ real depth $/$ refractive index $\mu$.
Since, the image formed by Medium $1, O_2$ act as an object for Medium 2.
If seen from $\mu_3$, the apparent depth is $O_2$.
Similarly, the image formed by Medium 2, $O_2$ act as an object for Medium 3
$$ \begin{aligned} O_2 & =\frac{\mu_3}{\mu_2} \frac{h}{3}+O_1 \\ & =\frac{\mu_3}{\mu_2} \frac{h}{3}+\frac{\mu_2 h}{\mu_1 3}=\frac{h}{3} \frac{\mu_3}{\mu_2}+\frac{\mu_2}{\mu_1} \end{aligned} $$
Seen from outside, the apparent height is
$$ \begin{aligned} O_3 & =\frac{1}{\mu_3} \frac{h}{3}+O_2=\frac{1}{\mu_3} \frac{h}{3}+\frac{h}{3} \frac{\mu_3}{\mu_2}+\frac{\mu_3}{\mu_1} \\ & =\frac{h}{3} \frac{1}{\mu_1}+\frac{1}{\mu_2}+\frac{1}{\mu_3} \end{aligned} $$
This is the required expression of apparent depth.
21. For a glass prism $(\mu=\sqrt{3})$, the angle of minimum deviation is equal to the angle of the prism. Find the angle of the prism.
Show Answer
Answer
The relationship between refractive index, prism angle $A$ and angle of minimum deviation is given by
$$ \mu=\frac{\sin \frac{(A+D_{m})}{2}}{\sin \frac{A}{2}} $$
Here,
$\therefore$ Given,
$$ D_{m}=A $$
Substituting the value, we have
$\therefore \quad \mu=\frac{\sin A}{\sin \frac{A}{2}}$
On solving, we have
$$ \begin{aligned} & =\frac{2 \sin \frac{A}{2} \cos \frac{A}{2}}{\sin \frac{A}{2}}=2 \cos \frac{A}{2} \\ \mu & =\frac{\sin A}{\sin \frac{A}{2}}=\frac{2 \sin \frac{A}{2} \cos \frac{A}{2}}{\sin \frac{A}{2}}=2 \cos \frac{A}{2} \end{aligned} $$
For the given value of refractive index,
we have
$$ \therefore \cos \frac{A}{2} =\frac{\sqrt{3}}{2} $$ $$ \text{or} \quad \quad s\frac{A}{2} =30^{\circ}$$ $$\therefore A =60^{\circ}$$
This is the required value of prism angle.
22. A short object of length $L$ is placed along the principal axis of a concave mirror away from focus. The object distance is $u$. If the mirror has a focal length $f$, what will be the length of the image? You may take $L«|v-f|$.
Show Answer
Thinking Process
The length of image is the separation between the images formed by mirror of the extremities of object.
Answer
Since, the object distance is $u$. Let us consider the two ends of the object be at distance $u_1=u-L / 2$ and $u_2=u+L / 2$, respectively so that $|u_1-u_2|=L$. Let the image of the two ends be formed at $v_1$ and $v_2$, respectively so that the image length would be
$$ L^{\prime}=|v_1-v_2| $$
Applying mirror formula, we have
$$ \frac{1}{u}+\frac{1}{v}=\frac{1}{f} \text { or } v=\frac{f u}{u-f} $$
On solving, the positions of two images are given by
$$ v_1=\frac{f(u-L / 2)}{u-f-L / 2}, v_2=\frac{f(u+L / 2)}{u-f+L / 2} $$
For length, substituting the value in (i), we have
$$ L^{\prime}=|v_1-v_2|=\frac{f^{2} L}{(u-f)^{2} \times L^{2} / 4} $$
Since, the object is short and kept away from focus, we have
$$ L^{2} / 4«(u-f)^2 \\ \text {Hence, finally} L^{\prime}=\frac{f^2}{(u-f)^2} $$
This is the required expression of length of image.
23. A circular disc of radius $R$ is placed co-axially and horizontally inside an opaque hemispherical bowl of radius a figure. The far edge of the disc is just visible when viewed from the edge of the bowl. The bowl is filled with transparent liquid of refractive index $\mu$ and the near edge of the disc becomes just visible. How far below the top of the bowl is the disc placed?
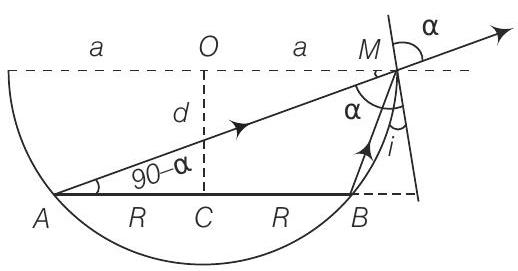
Show Answer
Thinking Process
This problem involves the wide application of geometry and Snell’s law.
Answer
Refering to the figure, $A M$ is the direction of incidence ray before liquid is filled. After liquid is filled in , $B M$ is the direction of the incident ray. Refracted ray in both cases is same as that along $A M$.
Let the disc is separated by $O$ at a distance $d$ as shown in figure. Also, considering angle
Here, in figure
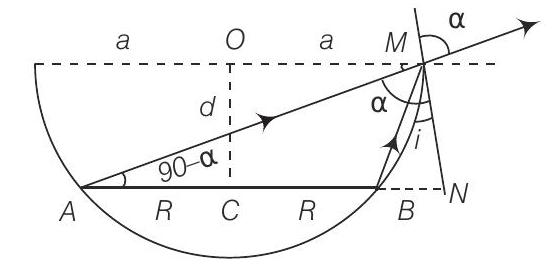
$$ N=90^{\circ}, O M=a, C B=N B=a-R, A N=a+R $$
and
$$ \begin{aligned} \sin t & =\frac{a-R}{\sqrt{d^{2}+(a-R)^{2}}} \\ \sin \alpha & =\cos (90-\alpha)=\frac{a+R}{\sqrt{d^{2}+(a+R)^{2}}} \end{aligned} $$
But on applying Snell’s law,
$$ \frac{1}{\mu}=\frac{\sin t}{\sin r}=\frac{\sin t}{\sin \alpha} $$
On substituting the values, we have the separation
$$ d=\frac{\mu(a^{2}-b^{2})}{\sqrt{(a+r)^{2}-\mu(a-r)^{2}}} $$
This is the required expression.
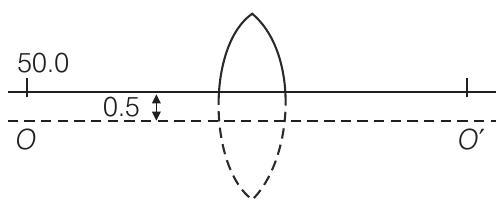
24. A thin convex lens of focal length $25 cm$ is cut into two pieces $0.5 cm$ above the principal axis. The top part is placed at $(0,0)$ and an object placed at $(-50 cm, 0)$. Find the coordinates of the image.
Show Answer
Thinking Process
There is no effect on the focal length of the lens if it is cut as given in the question.
Answer
If there was no cut, then the object would have been at a height of $0.5 cm$ from the principal axis $OO^{\prime}$.
Applying lens formula, we have
$$ \begin{aligned} & & \frac{1}{v}-\frac{1}{u} & =\frac{1}{f} \\ & \therefore & \frac{1}{v} & =\frac{1}{u}+\frac{1}{f}=\frac{1}{-50}+\frac{1}{25}=\frac{1}{50} \\ & & \text { Mangnification is } m & =\frac{v}{u}=-\frac{50}{50}=-1 \end{aligned} $$
Thus, the image would have been formed at $50 cm$ from the pole and $0.5 cm$ below the principal axis. Hence, with respect to the $X$-axis passing through the edge of the cut lens, the coordinates of the image are $(50 cm,-1 cm)$.
25. In many experimental set-ups, the source and screen are fixed at a distance say $D$ and the lens is movable. Show that there are two positions for the lens for which an image is formed on the screen. Find the distance between these points and the ratio of the image sizes for these two points.
Show Answer
Thinking Process
This is also one of the methods for finding the focal length of the lens in laboratory and known as displacement method.
Answer
Principal of reversibility is states that the position of object and image are interchangeable. So, by the versibility of $u$ and $v$, as seen from the formula for lens.
$$ \frac{1}{f}=\frac{1}{v}-\frac{1}{u} $$
It is clear that there are two positions for which there shall be an image.
On the screen, let the first position be when the lens is at $O$. Finding $u$ and $v$ and substituting in lens formula.
Given,
$\Rightarrow$
Placing it in the lens formula
$$ \frac{1}{D-v}+\frac{1}{v}=\frac{1}{f} $$
On solving, we have
$$ \begin{aligned} \Rightarrow \frac{v+D-v}{(D-v) v} =\frac{1}{f} \newline \newline\\ \Rightarrow v^{2}-D v+D f =0 \newline \newline\\ \Rightarrow v =\frac{D}{2} \pm \frac{\sqrt{D^{2}-4 D f}}{2} \end{aligned} $$
Hence, finding $u$
$$ u=-(D-v)=-\frac{D}{2} \pm \frac{\sqrt{D^{2}-4 D f}}{2} $$
When, the object distance is $\quad \frac{D}{2}+\frac{\sqrt{D^{2}-4 D f}}{2}$
the image forms at
$$ \frac{D}{2}-\frac{\sqrt{D^{2}-4 D f}}{2} $$
Similarly, when the object distance is
The image forms at
$$ \begin{aligned} & \frac{D}{2}-\frac{\sqrt{D^{2}-4 D f}}{2} \\ & \frac{D}{2}+\frac{\sqrt{D^{2}-4 D f}}{2} \end{aligned} $$
The distance between the poles for these two object distance is
$$ \frac{D}{2}+\frac{\sqrt{D^{2}-4 D f}}{2}-\frac{D}{2}-\frac{\sqrt{D^{2}-4 D f}}{2}=\sqrt{D^{2}-4 D f} $$
$$ \begin{aligned} & \text { Let } \\ & \text { If } u=\frac{D}{2}+\frac{d}{2} \text {, then the image is at } v=\frac{\sqrt{D^{2}-4 D f}}{2}-\frac{d}{2} . \\ & \therefore \quad \text { The magnification } m_1=\frac{D-d}{D+d} \\ & \text { If } u=\frac{D-d}{2} \text {, then } v=\frac{D+d}{2} \\ & \therefore \text { The magnification } m_2=\frac{D+d}{D-d} \\ & \text { Thus, } \quad \frac{m_2}{m_1}=\frac{D+d}{D-d} . \end{aligned} $$
This is the required expression of magnification.
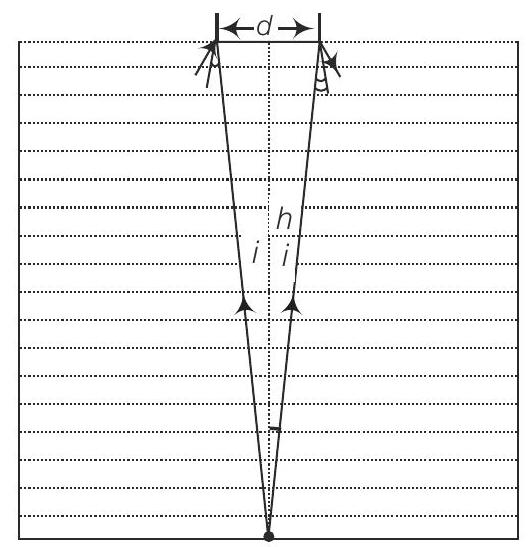
26. A jar of height $h$ is filled with a transparent liquid of refractive index $\mu$ figure. At the centre of the jar on the bottom surface is a dot. Find the minimum diameter of a disc, such that when placed on the top surface symmetrically about the centre, the dot is invisible.
Show Answer
Thinking Process
The problem is based on the principle of total internal reflection and area of visibility.
Answer
Let $d$ be the diameter of the disc. The spot shall be invisible if the incident rays from the dot at $O$ to the surface at $d / 2$ at the critical angle.
Let $i$ be the angle of incidence.
Using relationship between refractive index and critical angle,
then,
$$ \sin t=\frac{1}{\mu} $$
Using geometry and trigonometry.
Now,
$$ \begin{aligned} \frac{d / 2}{h} & =\tan i \\ \frac{d}{2} & =h \tan i=h[\sqrt{\mu^{2}-1}]^{-1} \\ d & =\frac{2 h}{\sqrt{\mu^{2}-1}} \end{aligned} $$
$$ \Rightarrow $$
This is the required expression of $d$.
27. myopic adult has a far point at $0.1 m$. His power of accomodation is $4 D$.
(i) What power lenses are required to see distant objects?
(ii) What is his near point without glasses?
(iii) What is his near point with glasses? (Take the image distance from the lens of the eye to the retina to be $2 cm$.)
Show Answer
Thinking Process
If two thin lenses of focal length $f_1$ and $f_2$ are in contact, the effective focal length of the combination is given by,
in terms of power $P=P_1+P_2$
$$ \frac{1}{f}=\frac{1}{f_1}+\frac{1}{f_2} $$
Answer
(i) Let the power at the far point be $P_{f}$ for the normal relaxed eye of an average person. The required power
$$ P_{f}=\frac{1}{f}=\frac{1}{0.1}+\frac{1}{0.02}=60 D $$
By the corrective lens the object distance at the far point is $\infty$.
The power required is
$$ P_f^{\prime}=\frac{1}{f^{\prime}}=\frac{1}{\infty}+\frac{1}{0.02}=50 D $$
So for eye + lens system,
we have the sum of the eye and that of the glasses $P_{g}$
$$ \begin{matrix} \therefore & P_f^{\prime}=P_{f}+P_{g} \\ \therefore & P_{g}=-10 D \end{matrix} $$
(ii) His power of accomodatlon is $4 D$ for the normal eye. Let the power of the normal eye for near vision be $P_{n}$.
$$ \text { Then, } \quad 4=P_{n}-P_{f} \text { or } P_{n}=64 D $$
Let his near point be $x_{n}$, then
$$ \begin{aligned} \frac{1}{x_{n}}+\frac{1}{0.02} & =64 \text { or } \frac{1}{x_{n}}+50=64 \\ \frac{1}{x_{n}} & =14, \\ \therefore \quad x_{n} & =\frac{1}{14} ; 0.07 m \end{aligned} $$
(iii) With glasses $P_n^{\prime}=P_f^{\prime}+4=54$
$$ \begin{aligned} 54 & =\frac{1}{x_n^{\prime}}+\frac{1}{0.02}=\frac{1}{x_n^{\prime}}+50 \\ \frac{1}{x_n^{\prime}} & =4 \\ \therefore \quad x_n^{\prime} & =\frac{1}{4}=0.25 m \end{aligned} $$
28. Show that for a material with refractive index $\mu \geq \sqrt{2}$, light incident at any angle shall be guided along a length perpendicular to the incident face.
Show Answer
Answer
Any ray entering at an angle $i$ shall be guided along $A C$ if the angle ray makes with the face $A C(\varphi)$ is greater than the critical angle as per the principle of total internal reflection $\varphi+r=90^{\circ}$, therefore $\sin \varphi=\cos r$
$\begin{aligned} \Rightarrow \qquad \sin \varphi & \geq \frac{1}{\mu} \\ \Rightarrow \qquad \cos r & \geq \frac{1}{\mu} \\ \text{or} \qquad 1-\cos ^2 r & \leq 1-\frac{1}{\mu^2} \\ \text{i.e.,} \qquad \sin ^2 r & \leq \frac{1}{\mu^2} \end{aligned}$
$\begin{aligned} & \text { i.e., } \qquad \sin ^2 r \leq 1-\frac{1}{\mu^2} \\ \qquad & \text { since, } \qquad \sin i=\mu \sin r \\ & \frac{1}{\mu_2} \sin ^2 i \leq 1-\frac{1}{\mu^2} \\ & \text { when } \qquad i=\frac{\pi}{2} \\ & \end{aligned}$
Then,we have smallest angle $\varphi$.
If that is greater than the critical angle,then all other the critical angle.
$\begin{array}{ll} \text { Thus, }\qquad & 1 \leq \mu^2-1 \\ \text { or } & \mu^2 \geq 2 \\ \Rightarrow & \mu \geq \sqrt{2} \end{array}$
This is the required result.
29. The mixture a pure liquid and a solution in a long vertical column (i.e., horizontal dimensions $\ll$ vertical dimensions) produces diffusion of solute particles and hence a refractive index gradient along the vertical dimension. A ray of light entering the column at right angles to the vertical is deviated from its original path. Find the deviation in travelling a horizontal distance $d \ll<$, the height of the column.
Show Answer
Answer
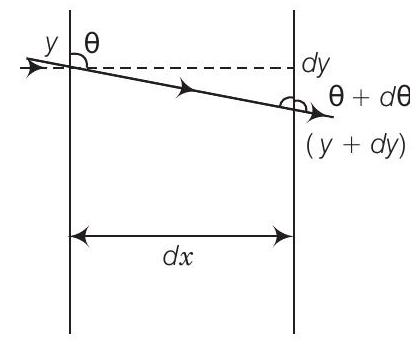
Let us consider a portion of a ray between $x$ and $x+d x$ inside the liquid. Let the angle of incidence at $x$ be $\theta$ and let it enter the thin column at height $y$. Because of the bending it shall emerge at $x+d x$ with an angle $\theta+d \theta$ and at a height $y+d y$. From Snell’s law,
$\mu(y) \sin \theta=\mu(y+d y) \sin (\theta+d \theta)$
$\mu(y) \sin \theta ; \mu(y)+\frac{d \mu}{d y} d y \quad(\sin \theta \cos d \theta+\cos \theta \sin d \theta) \newline$
$\mu(y) \sin \theta+\mu(y) \cos \theta d \theta+\frac{d \mu}{d y} d y \sin \theta \newline$
$\mu(y) \cos \theta d \theta ; \frac{-d \mu}{d y} d y \sin \theta$ $d \theta ; \frac{-d \mu}{\mu d y} d y \tan \theta$
$$ \tan \theta=\frac{d x}{d y} $$
(from the figure)
On solving, we have
$$ \therefore \quad d \theta=\frac{-1 d \mu}{\mu d y} d x $$
Solving this variable separable form of differential equation.
$$ \therefore \quad \theta=\frac{-1 d \mu}{\mu d y} \int_0^{d} d x=\frac{-1 d \mu}{\mu d y} d $$
30. If light passes near a massive object, the gravitational interaction causes a bending of the ray. This can be thought of as happening due to a change in the effective refractive index of the medium given by
$$ n(r)=1+2 G M / r c^{2} $$
where $r$ is the distance of the point of consideration from the centre of the mass of the massive body, $G$ is the universal gravitational constant, $M$ the mass of the body and $c$ the speed of light in vacuum. Considering a spherical object find the deviation of the ray from the original path as it grazes the object.
Show Answer
Answer
Let us consider two planes at $r$ and $r+d r$. Let the light be incident at an angle $\theta$ at the plane at $r$ and leave $r+d r$ at an angle $\theta+d \theta$. Then from Snell’s law,
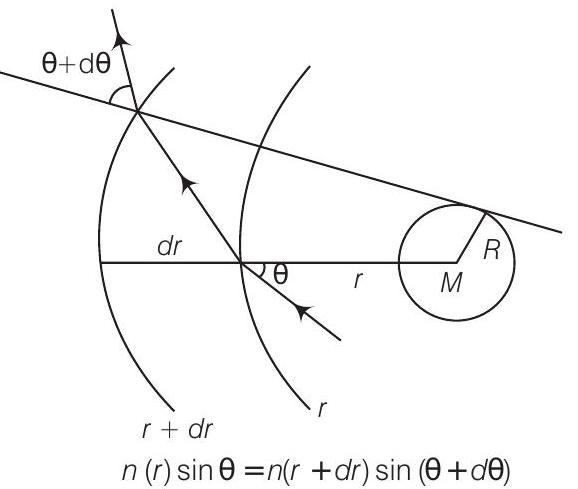
$\Rightarrow \quad n(r) \sin \theta ; n(r)+\frac{d n}{d r} d r \quad(\sin \theta \cos d \theta+\cos \theta \sin d \theta)$
$$ n(r)+\frac{d n}{d r} d r \quad(\sin \theta+\cos \theta d \theta) $$
Ignoring the product of differentials
or we have,
$$ \begin{aligned} n(r) \sin \theta ; n(r) \sin \theta & +\frac{d n}{d r} d r \sin \theta+n(r) \cos \theta d \theta \\ -\frac{d n}{d r} \tan \theta & =n(r) \frac{d \theta}{d r} \\ \frac{2 G M}{r^{2} c^{2}} \tan \theta & =1+\frac{2 G M}{r^{2}} \frac{d \theta}{d r} \approx \frac{d \theta}{d r} \\ \int_0^{\theta_0} d \theta & =\frac{2 G M}{c^{2}} \int_{-\infty}^{\infty} \frac{\tan \theta d r}{r^{2}} \end{aligned} $$
Now substitution for integrals, we have
$$ \text { Now, } \quad \begin{aligned} r^{2} & =x^{2}+R^{2} \text { and } \tan \theta=\frac{R}{x} \\ 2 r d r & =2 x d x \\ \text { Put } \quad \int_0^{\theta_0} d \theta & =\frac{2 G M}{c^{2}} \int_{-\infty}^{\infty} \frac{R}{x} \frac{x d x}{(x^{2}+R^{2})^{\frac{3}{2}}} \\ x & =R \tan \varphi \\ d x & =R \sec ^{2} \varphi d \varphi \\ \theta_0 & =\frac{2 G M R}{c^{2}} \int_{-\pi / 2}^{\pi / 2} \frac{R \sec ^{2} \varphi d \varphi}{R^{3} \sec ^{3} \varphi} \\ & =\frac{2 G M}{R c^{2}} \int_{-\pi / 2}^{\pi / 2} \cos \varphi d \varphi=\frac{4 G M}{R c^{2}} \end{aligned} $$
This is the required proof.
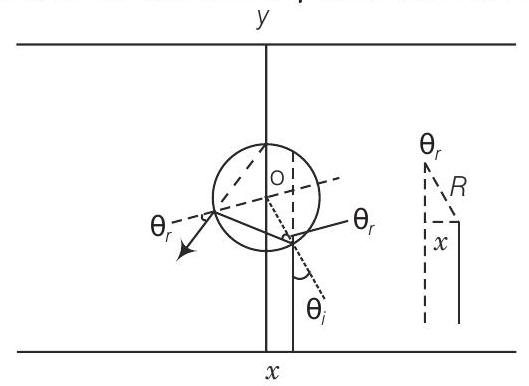
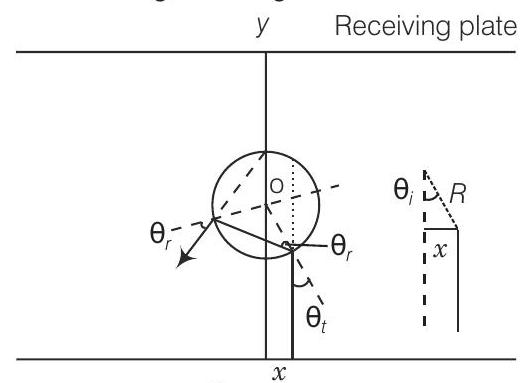
31. An infinitely long cylinder of radius $R$ is made of an unusual exotic material with refractive index-1 (figure). The cylinder is placed between two planes whose normals are along the $y$-direction. The centre of the cylinder 0 lies along the $y$-axis. A narrow laser beam is directed along the $y$-direction from the lower plate. The laser source is at a horizontal distance $x$ from the diameter in the $y$ direction. Find the range of $x$ such that light emitted from the lower plane does not reach the upper plane.
Show Answer
Answer
Since, the material is of refractive index $-1, \theta_{r}$, is negative and $\theta_r^{\prime}$ positive.
Now,
$$ |\theta_{t}|=|\theta_{r}|=|\theta_r^{\prime}| $$
The total deviation of the outcoming ray from the incoming ray is $4 \theta$. Rays shall not reach the recieving plate if
$$ .\frac{\pi}{2} \leq 4 \theta_{t} \leq \frac{3 \pi}{2} \quad \text { [angles measured clockwise from the } y \text {-axis }] $$
On solving,
$$ \frac{\pi}{8} \leq \theta_{t} \leq \frac{3 \pi}{8} $$
Now,
or
$$ \begin{aligned} \sin \theta_{t} & =\frac{x}{R} \\ \frac{\pi}{8} & \leq \sin ^{-1} \frac{x}{R} \leq \frac{3 \pi}{8} \\ \frac{\pi}{8} & \leq \frac{x}{R} \leq \frac{3 \pi}{8} \end{aligned} $$
Thus, for light emitted from the source shall not reach the receiving plate. If $\frac{R \pi}{8} \leq x \leq \frac{R 3 \pi}{8}$.
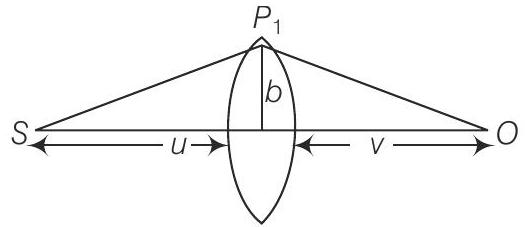
32. (i) Consider a thin lens placed between a source $(S)$ and an observer ( 0 ) (Figure). Let the thickness of the lens vary as $w(b)=w_0-\frac{b^{2}}{\alpha}$, where $b$ is the verticle distance from the pole, $w_0$ is a constant. Using Fermat’s principle i.e., the time of transit for a ray between the source and observer is an extremum find the condition that all paraxial rays starting from the source will converge at a point 0 on the axis. Find the focal length.
(ii) A gravitational lens may be assumed to have a varying width of the form
$$ \begin{aligned} w(b) & =k_1 \ln \frac{k_2}{b} \quad b_{\text {min }}<b<b_{\max } \\ & =k_1 \ln \frac{k_2}{b_{\text {min }}} \quad b<b_{\text {min }} \end{aligned} $$
Show that an observer will see an image of a point object as a ring about the centre of the lens with an angular radius
$$ \beta=\sqrt{\frac{(n-1) k_1 \frac{u}{v}}{u+v}} $$
Show Answer
Answer
(i) The time elapsed to travel from $S$ to $P_1$ is
$$ t_1=\frac{S P_1}{c}=\frac{\sqrt{u^{2}+b^{2}}}{c} $$
or
$$ \frac{u}{c} 1+\frac{1}{2} \frac{b^{2}}{u^{2}} \text { assuming } b«u_0 \text {. } $$
The time required to travel from $P_1$ to $O$ is
$$ t_2=\frac{P_1 O}{c}=\frac{\sqrt{v^{2}+b^{2}}}{c} ; \frac{v}{c} 1+\frac{1}{2} \frac{b^{2}}{v^{2}} $$
The time required to travel through the lens is
$$ t_1=\frac{(n-1) w(b)}{c} $$
where $n$ is the refractive index.
Thus, the total time is
$$ t=\frac{1}{c} u+v+\frac{1}{2} b^{2} \frac{1}{u}+\frac{1}{v}+(n-1) w(b) $$
Put
$$ \frac{1}{D}=\frac{1}{u}+\frac{1}{v} $$
Then,
$$ t=\frac{1}{c} u+v+\frac{1}{2} \frac{b^{2}}{D}+(n-1) w_0+\frac{b^{2}}{\alpha} $$
Fermet’s principle gives the time taken should be minimum.
For that first derivative should be zero.
$$ \begin{aligned} \frac{d t}{d b} & =0=\frac{b}{C D}-\frac{2(n-1) b}{c \alpha} \\ \alpha & =2(n-1) D \end{aligned} $$
Thus, a convergent lens is formed if $\alpha=2(n-1) D$. This is independant of and hence, all paraxial rays from $S$ will converge at $O$ i.e., for rays
and
$$ (b«v .) $$
Since, $\frac{1}{D}=\frac{1}{u}+\frac{1}{V}$, the focal length is $D$.
(ii) In this case, differentiating expression of time taken $t$ w.r.t. $b$
$$ t =\frac{1}{c} u+v+ \frac{1}{2} \frac{b^2}{D}+(n-1) k_1 \ln \frac{k_2}{b} \newline $$ $$ \Rightarrow \frac{d t}{d b} =0=\frac{b}{D}-(n-1) \frac{k_1}{b} \newline \therefore b^{2} =(n-1) k_1 D \newline \therefore b =\sqrt{(n-1) k_1 D} \newline $$
Thus, all rays passing at a height $b$ shall contribute to the image. The ray paths make an angle.
$$ \beta ; \frac{b}{v}=\frac{\sqrt{(n-1) k_1 D}}{v^{2}}=\sqrt{\frac{(n-1) k_1 u v}{v^{2}(u+v)}}=\sqrt{\frac{(n-1) k_1 u}{(u+v) v}} $$
This is the required expression.










