Chapter 05 Magnetism and Matter
Multiple Choice Questions (MCQs)
1. A toroid of $n$ turns, mean radius $R$ and cross-sectional radius a carries current $I$. It is placed on a horizontal table taken as $x y$-plane. Its magnetic moment $\mathbf{m}$
(a) is non-zero and points in the $z$-direction by symmetry
(b) points along the axis of the toroid $(m=m \varphi)$
(c) is zero, otherwise there would be a field falling as $\frac{1}{r^{3}}$ at large distances outside the toroid
(d) is pointing radially outwards
Show Answer
Thinking Process
Toroid is a hollow circular ring on which a large number of turns of a wire are closely wound. Thus, in such a case magnetic field is only confined inside the body of toroid.
Answer
(c) In case of toroid, the magnetic field is only confined inside the body of toroid in the form of concentric magnetic lines of force and there is no magnetic field outside the body of toroid. This is because the loop encloses no current. Thus, the magnetic moment of toroid is zero.
In general, if we take $r$ as a large distance outside the toroid, then $m \propto \frac{1}{r^{3}}$. But this case is not possible here.
2. The magnetic field of the earth can be modelled by that of a point dipole placed at the centre of the earth. The dipole axis makes an angle of $11.3^{\circ}$ with the axis of the earth. At Mumbai, declination is nearly zero. Then,
(a) the declination varies between $11.3^{\circ} \mathrm{W}$ to $11.3^{\circ} \mathrm{E}$
(b) the least declination is $0^{\circ}$
(c) the plane defined by dipole axis and the earth axis passes through Greenwich
(d) declination averaged over the earth must be always negative
Show Answer
Answer
(a) For the earth’s magnetism, the magnetic field lines of the earth resemble that of a hypothetical magnetic dipole located at the centre of the earth.
The axis of the dipole does not coincide with the axis of rotation of the earth but is presently tilted by approxmately $11.3^{\circ}$ with respect to the later. This results into two situations as given in the figure ahead.
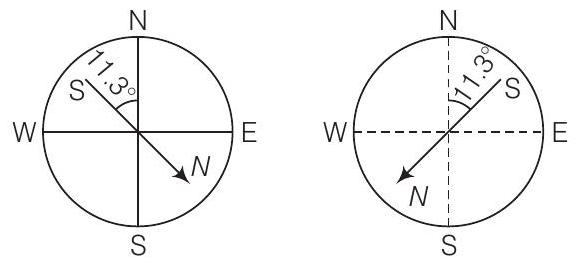
Hence, the declination varies between $11.3^{\circ} \mathrm{W}$ to $11.3^{\circ} \mathrm{E}$.
3. In a permanent magnet at room temperature,
(a) magnetic moment of each molecule is zero $\newline$
(b) the individual molecules have non-zero magnetic moment which are all perfectly aligned $\newline$
(c) domains are partially aligned $\newline$
(d) domains are all perfectly aligned $\newline$
Show Answer
Thinking Process
Permanent magnet at room temperature behave as a ferromagnetic substance for a long period of time.
Answer
(d) As we know a permanent magnet is a substance which at room temperature retain ferromagnetic property for a long period of time.
The individual atoms in a ferromagnetic material possess a dipole moment as in a paramagnetic material.
However, they interact with one another in such a way that they spontaneously align themselves in a common direction over a macroscopic volume called domain. Thus, we can say that in a permanent magnet at room temperature, domains are all perfectly aligned.
4. Consider the two idealised systems (i) a parallel plate capacitor with large plates and small separation and (ii) a long solenoid of length $L \gg R$, radius of cross-section. In (i) $\mathbf{E}$ is ideally treated as a constant between plates and zero outside. In (ii) magnetic field is constant inside the solenoid and zero outside. These idealised assumptions, however, contradict fundamental laws as below
(a) case (i) contradicts Gauss’ law for electrostatic fields
(b) case (ii) contradicts Gauss’ law for magnetic fields
(c) case (i) agrees with $\oint \mathrm{E}$. dl $=0$.
(d) case (ii) contradicts $\oint \mathrm{H} \cdot \mathrm{dl}=I_{\mathrm{e}}$
Show Answer
Thinking Process
The electric field lines, do not form a continuous closed path while the magnetic field lines form the closed paths.
Answer
(b) As Gauss’ law states, $\int_{S} E \cdot d s=\frac{q}{\varepsilon_{0}}$ for electrostatic field. It does not contradict for electrostatic fields as the electric field lines do not form continuous closed path.
According to Gauss’ law in magnetic field,
$$ \int_{S} \mathrm{E} \cdot \mathrm{ds}=0 $$
It contradicts for magnetic field, because there is a magnetic field inside the solenoid and no field outside the solenoid carrying current but the magnetic field lines form the closed path.
5. paramagnetic sample shows a net magnetisation of $8 \mathrm{Am}^{-1}$ when placed in an external magnetic field of $0.6 \mathrm{~T}$ at a temperature of $4 \mathrm{~K}$. When the same sample is placed in an external magnetic field of $0.2 \mathrm{~T}$ at a temperature of $16 \mathrm{~K}$, the magnetisation will be $\newline$
(a) $\frac{32}{3} \mathrm{Am}^{-1}$ $\newline$
(b) $\frac{2}{3} \mathrm{Am}^{-1}$ $\newline$
(c) $6 \mathrm{Am}^{-1}$ $\newline$
(d) $2.4 \mathrm{Am}^{-1}$ $\newline$
Show Answer
Thinking Process
From Curie law, we know that magnetisation is directly proportional to the magnetic field induction and inversely proportional to the temperature in kelvin.
Answer
(b) As Curie law explains, we can deduce a formula for the relation between magnetic field induction, temperature and magnetisation.
i.e., $\quad I$ (magnetisation $) \propto \frac{B \text { (magnetic field induction })}{t \text { (temperature in kelvin })}$
$\begin{array}{rlrl} \Rightarrow & \frac{I_2}{I_1} =\frac{B_2}{B_1} \times \frac{t_1}{t_2} \\ \text { Let us suppose, here } & I_1 =8 \mathrm{Am}^{-1} \\ B_1 & =0.6 \mathrm{~T}, t_1=4 \mathrm{~K} \\ B_2 & =0.2 \mathrm{~T}, t_2=16 \mathrm{~K} \\ I_2=? \\ \Rightarrow \quad & \frac{0.2}{0.6} \times \frac{4}{16} =\frac{I_2}{8} \\ \Rightarrow \quad & I_2 =8 \times \frac{1}{12}=\frac{2}{3} \mathrm{Am}^{-1} \end{array}$
Multiple Choice Questions (More Than One Options)
6. $S$ is the surface of a lump of magnetic material.
(a) Lines of $\mathbf{B}$ are necessarily continuous across $S$
(b) Some lines of $\mathbf{B}$ must be discontinuous across $S$
(c) Lines of $\mathbf{H}$ are necessarily continuous across $S$
(d) Lines of $\mathbf{H}$ cannot all be continuous across $S$
Show Answer
Thinking Process
According to the properties of magnetic field lines (B), for any magnet, it forms continuous closed loops. This is unlike the electric dipole where these field lines begin from a positive charge and end on the negative charge or escape to infinity.
Also, magnetic intensity $(H)$ outside any magnet is $H=B / \mu_{0}$ and for inside the magnet $H=B / \mu_{0} \mu_{r}$, where $\mu_{r}$ is the relative permeability of material (magnetic).
Answer
$(a, d)$
Magnetic field lines for magnetic induction (B) form continuous lines. So, lines of B are necessarily continuous across $S$.
Also, magnetic intensity $\mathbf{( H )}$ varies for inside and outside the lump. So, lines of $\mathbf{H}$ cannot all be continuous across $S$.
7. The primary origin (s) of magnetism lies in
(a) atomic currents
(b) Pauli exclusion principle
(c) polar nature of molecules
(d) intrinsic spin of electron
Show Answer
Answer
$(a, d)$
The primary origin of magnetism lies in the fact that the electrons are revolving and spinning about nucleus of an atom, which gives rise to current called atomic current.
This atomic currents gives rise to magnetism. The revolving and spinning about nucleus of an atom is called intrinsic spin of electron.
8. A long solenoid has 1000 turns per metre and carries a current of $1 \mathrm{~A}$. It has a soft iron core of $\mu_{r}=1000$. The core is heated beyond the Curie temperature, $T_{c}$.
(a) The $\mathbf{H}$ field in the solenoid is (nearly) unchanged but the $\mathbf{B}$ field decreases drastically
(b) The $\mathbf{H}$ and $\mathbf{B}$ fields in the solenoid are nearly unchanged
(c) The magnetisation in the core reverses direction
(d) The magnetisation in the core diminishes by a factor of about $10^{8}$
Show Answer
Thinking Process
The magnetic intensity $\mathbf{H}$ field $=n \boldsymbol{I}$, where $n=$ number of turns per metre of a solenoid
and $I=$ current and $B=\mu_{0} \mu_{r} n I$.
Also, at normal temperature, a solenoid behave as a ferromagnetic substand and at the temperature beyond the Curie temperature, it behaves as a paramagnetic substance.
Answer
(a, $d)$
Here, for solenoid $\mathbf{H}=n I$.
$\Rightarrow \quad \mathrm{H}=1000 \times 1=1000 \mathrm{Am}$
Thus, $\mathbf{H}$ is a constant, so it is nearly unchanged.
But
$$ \begin{aligned} B & =\mu_{0} \mu_{r} n I \\ & =\mu_{0} n I \mu_{r} \\ & =k \text { (constant) } \mu_{r} . \end{aligned} $$
Thus, from above equation, we find that $\mathbf{B}$ varies with the variation in $\mu_{r}$.
Now, for magnetisation in the core, when temperature of the iron core of solenoid is raised beyond Curie temperature, then it behave as paramagnetic material, where
$\begin{aligned} \text{and} \quad (\chi_m) _{\text {Fero }} & \approx 10^3 \\ \text{and} \quad (\chi_m) _{\text {Para }} & \approx 10^{-5} \\ \Rightarrow \quad\frac{(\chi_mt) _{\text {Fero }}}{(\chi_m)} & =\frac{10^3}{10^{-5}}=10^8 \end{aligned}$
9. Essential difference between electrostatic shielding by a conducting shell and magnetostatic shielding is due to
(a) electrostatic field lines can end on charges and conductors have free charges
(b) lines of $\mathbf{B}$ can also end but conductors cannot end them
(c) lines of $\mathbf{B}$ cannot end on any material and perfect shielding is not possible
(d) shells of high permeability materials can be used to divert lines of $\mathbf{B}$ from the interior region
Show Answer
Answer
$(a, c, d)$
Electrostatic shielding is the phenomenon to block the effects of an electric field. The conducting shell can block the effects of an external field on its internal content or the effect of an internal field on the outside environment.
Magnetostatic shielding is done by using an enclosure made of a high permeability magnetic material to prevent a static magnetic field outside the enclosure from reaching objects inside it or to confine a magnetic field within the enclosure.
10. Let the magnetic field on the earth be modelled by that of a point magnetic dipole at the centre of the earth. The angle of dip at a point on the geographical equator
(a) is always zero
(b) can be zero at specific points
(c) can be positive or negative
(d) is bounded
Show Answer
Thinking Process
Angle of inclination or dip is the angle that the total magnetic field of the earth makes with the surface of the earth.
Answer
$(b, c, d)$
If the total magnetic field of the earth is modelled by a point magnetic dipole at the centre, then it is in the same plane of geographical equator, thus the angle of dip at a point on the geographical equator is bounded in a range from positive to negative value.
Very Short Answer Type Questions
11. A proton has spin and magnetic moment just like an electron. Why then its effect is neglected in magnetism of materials
Show Answer
Thinking Process
Mass of a proton is very larger than the mass of an electron, so its spinning is negligible as compared to that of electron spin.
Answer
The comparison between the spinning of a proton and an electron can be done by comparing their magnetic dipole moment which can be given by
$$ \begin{aligned} & M & =\frac{e h}{4 \pi m} \text { or } M \propto \frac{1}{m} & \newline \ \therefore & & \frac{M_{p}}{M_{e}} & =\frac{m_{e}}{m_{p}} \ & =\frac{M_{e}}{1837 M_{e}} & & \left(\because \frac{e h}{4 \pi}=\text { constant }\right) \ \Rightarrow & & \frac{M_{p}}{M_{e}} & =\frac{1}{1837} \ll 1 \ \Rightarrow & M_{p} & \ll M_{e} & \left(\because M_{p}=1837 m_{e}\right) \end{aligned} $$
Thus, effect of magnetic moment of proton is neglected as compared to that of electron.
12. A permanent magnet in the shape of a thin cylinder of length $10 \mathrm{~cm}$ has $M=10^{6} \mathrm{~A} / \mathrm{m}$. Calculate the magnetisation current $I_{M}$.
Show Answer
Answer
Given, $M$ (intensity of magnetisation) $=10^{6} \mathrm{~A} / \mathrm{m}$.
$$ \begin{aligned} l \text { (length) } & =10 \mathrm{~cm}=10 \times 10^{-2} \mathrm{~m}=0.1 \mathrm{~m} \\ I_{M} & =\text { magnetisation current } \\ M & =\frac{I_{M}}{l} \\ I_{M} & =M \times l \\ & =10^{6} \times 0.1=10^{5} \mathrm{~A} \end{aligned} $$
$$ \text { and } \quad I_{M}=\text { magnetisation current } $$
We know that
Note Here, $M=$ intensity of magnetisation as its unit is given as $A / m$.
13. Explain quantitatively the order of magnitude difference between the diamagnetic susceptibility of $\mathrm{N}_{2}\left(\sim 5 \times 10^{-9}\right)$ (at STP) and $\mathrm{Cu}\left(\sim 10^{-5}\right)$.
Show Answer
Thinking Process
Magnetic susceptibility is a measure of how a magnetic material responds to an external field.
Answer
We know that
Also,
$$ \begin{array}{r} \text { Density of nitrogen } \rho_{N}{2}=\frac{28 ~g}{22.4 ~L}=\frac{28 ~g}{22400 cc} \\ \text { density of copper } \rho_{Cu}=\frac{8 ~g}{22.4 L}=\frac{8 ~g}{22400 cc} \end{array} $$
Now, comparing both densities
Also given
$$ \begin{aligned} \frac{\rho_{N_2}}{\rho_{{Cu}}} & =\frac{28}{22400} \times \frac{1}{8}=1.6 \times 10^{-4} \newline \ \frac{\chi_{N_{2}}}{\chi_{{Cu}}} & =\frac{5 \times 10^{-9}}{10^{-5}}=5 \times 10^{-4} \newline \ \chi & =\frac{\text { Magnetisation }(M)}{\text { Magnetic intensity }(H)} \newline \ & =\frac{\text { Magnetic moment }(M) / \text { Volume }(V)}{H} \newline \ & =\frac{M}{H V}=\frac{M}{H \text { (mass / density) }}=\frac{M \rho}{H m} \end{aligned} $$
We know that, $\quad \chi=\frac{\text { Magnetisation }(M)}{\text { Magnetic intensity }(H)}$
$\therefore \quad \chi \propto \rho \quad\left(\because \frac{M}{H m}=\right.$ constant $)$
Hence,
$$ \frac{{N_2}}{{{Cu}}}=\frac{\rho_{N_2}}{{Cu}}=1.6 \times 10^{-4} $$
Thus, we can say that magnitude difference or major difference between the diamagnetic susceptibility of ${N}_{2}$ and ${Cu}$.
14. From molecular view point, discuss the temperature dependence of susceptibility for diamagnetism, paramagnetism and ferromagnetism.
Show Answer
Answer
Susceptibility of magnetic material $\chi=\frac{I}{H}$, where $I$ is the intensity of magnetisation induced in the material and $H$ is the magnetising force.
Diamagnetism is due to orbital motion of electrons in an atom developing magnetic moments opposite to applied field. Thus, the resultant magnetic moment of the diamagnetic material is zero and hence, the susceptibility $\chi$ of diamagnetic material is not much affected by temperature.
Paramagnetism and ferromagnetism is due to alignments of atomic magnetic moments in the direction of the applied field. As temperature is raised, the alignment is disturbed, resulting decrease in susceptibility of both with increase in temperature.
15. $A$ ball of superconducting material is dipped in liquid nitrogen and placed near a bar magnet.
(i) In which direction will it move?
(ii) What will be the direction of its magnetic moment?
Show Answer
Thinking Process
A superconducting material and nitrogen are diamagnetic in nature.
Answer
When a diamagnetic material is dipped in liquid nitrogen, it again behaves as a diamagnetic material. Thus, superconducting material will again behave as a diamagnetic material. When this diamagnetic material is placed near a bar magnet, it will be feebly magnetised opposite to the direction of magnetising field.
(i) Thus, it will be repelled.
(ii) Also its direction of magnetic moment will be opposite to the direction of magnetic field of magnet.
Short Answer Type Questions
16. Verify the Gauss’s law for magnetic field of a point dipole of dipole moment $\mathbf{m}$ at the origin for the surface which is a sphere of radius $R$.
Show Answer
Answer
Let us draw the figure for given situation,
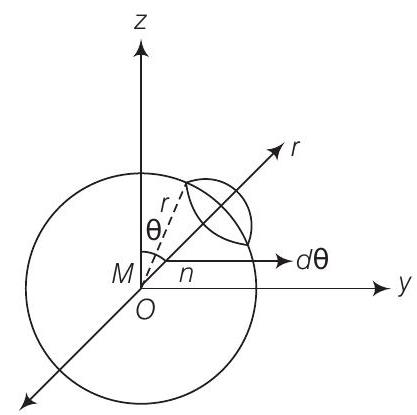
We have to prove that $g \mathbf{B} \cdot d \mathbf{S}=0$. This is called Gauss’s law in magnetisation.
According to question,
Magnetic moment of dipole at origin $O$ is
$$ \mathbf{M}=M \hat{k} $$
Let $P$ be a point at distance $r$ from $O$ and $O P$ makes an angle $\theta$ with $z$-axis. Component of $\mathbf{M}$ along $O P=M \cos \theta$.
Now, the magnetic field induction at $P$ due to dipole of moment $\mathbf{M} \cos \theta$ is
$$ \mathbf{B}=\frac{\mu_{0}}{4 \pi} \frac{2 M \cos \theta}{r^{3}} \hat{r} $$
From the diagram, $r$ is the radius of sphere with centre at $O$ lying in yz-plane. Take an elementary area $\mathbf{S}$ of the surface at $P$. Then,
$$ \begin{aligned} d \mathbf{S} & =r(r \sin \theta d \theta) \hat{\mathbf{r}}=r^{2} \sin \theta d \theta \hat{\mathbf{r}} \\ g \mathbf{B} \cdot d \mathbf{S} & =\oint \frac{\mu_{0}}{4 \pi} \frac{2 M \cos \theta}{r^{3}} \hat{\mathbf{r}}\left(r^{2} \sin \theta d \theta \hat{\mathbf{r}}\right) \\ & =\frac{\mu_{0}}{4 \pi} \frac{M}{r} \int_{0}^{2 \pi} 2 \sin \theta \cdot \cos \theta d \theta \\ & =\frac{\mu_{0}}{4 \pi} \frac{M}{r} \int_{0}^{2 \pi} \sin 2 \theta d \theta \\ & =\frac{\mu_{0}}{4 \pi} \frac{M}{r} \frac{-\cos 2 \theta}{2} 0 \\ & =-\frac{\mu_{0}}{4 \pi} \frac{M}{2 r}[\cos 4 \pi-\cos 0] \\ & =\frac{\mu_{0}}{4 \pi} \frac{M}{2 r}[1-1]=0 \end{aligned} $$
17. Three identical bar magnets are rivetted together at centre in the same plane as shown in figure. This system is placed at rest in a slowly varying magnetic field. It is found that the system of magnets does not show any motion. The north-south poles of one magnet is shown in the figure. Determine the poles of the remaining two.
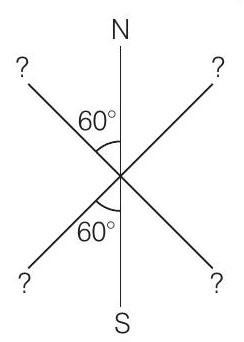
Show Answer
Answer
The system will be in stable equilibrium if the net force on the system is zero and net torque on the system is also zero. This is possible only when the poles of the remaining two magnets are as given in the figure.
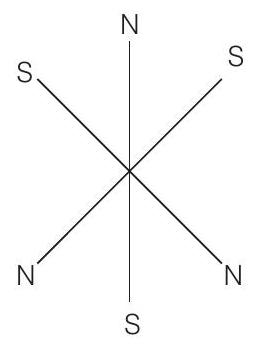
18. Suppose we want to verify the analogy between electrostatic and magnetostatic by an explicit experiment. Consider the motion of (i) electric dipole $\mathbf{p}$ in an electrostatic field $\mathbf{E}$ and (ii) magnetic dipole $\mathbf{M}$ in a magnetic field $\mathbf{B}$. Write down a set of conditions on $\mathbf{E}, \mathbf{B}, \mathbf{p}, \mathbf{M}$ so that the two motions are verified to be identical. (Assume identical initial conditions).
Show Answer
Thinking Process
$E(r)=c B(r)$, suppose the angle between $\mathbf{p}$ and $\mathbf{E}$ is $\boldsymbol{\theta}$. Torque on electric dipole of moment
$\mathbf{p}$ in electric field $\mathbf{E}, \tau=p E \sin \theta$.
Answer
Now, suppose that the angle between $\mathbf{M}$ and $B$ is $\theta$.
Torque on magnetic dipole moment $\mathbf{M}$ in magnetic field $\mathbf{B}$,
$$ \tau^{\prime}=M B \sin \theta $$
Two motions will be identical, if
$$ \Rightarrow $$
But,
$$ \begin{aligned} p E \sin \theta & =M B \sin \theta \ p E & =M B \ E & =c B \end{aligned} $$
$\therefore$ Putting this value in E(i),
$$ \begin{gathered} p c B=M B \ p=\frac{M}{c} \end{gathered} $$
19. A bar magnet of magnetic moment $M$ and moment of inertia $I$ (about centre, perpendicular to length) is cut into two equal pieces, perpendicular to length. Let $T$ be the period of oscillations of the original magnet about an axis through the mid-point, perpendicular to length, in a magnetic field $B$. What would be the similar period $T^{\prime}$ for each pieceK Thinking Process
$$ T=2 \pi \sqrt{\frac{I}{M B}} $$
$$ \text { where, } \begin{aligned} T & =\text { time period } \\ I & =\text { moment of inertia } \\ m & =\text { mass of magnet } \\ B & =\text { magnetic field } \end{aligned} $$
Show Answer
Answer
Given, $I=$ moment of inertia of the bar magnet
$m=$ mass of bar magnet
$l=$ length of magnet about an any passing through its centre and perpendicular to its length
$M=$ magnetic moment of the magnet
$B=$ uniform magnetic field in which magnet is oscillating, we get time period of
oscillation is,
$$ T=2 \pi \sqrt{\frac{I}{M B}} $$
Here,
$$ I=\frac{m l^{2}}{12} $$
When magnet is cut into two equal pieces, perpendicular to length, then moment of inertia of each piece of magnet about an axis perpendicular to length passing through its centre is
Magnetic dipole moment
$$ I^{\prime}=\frac{m}{2} \frac{(l / 2)^{2}}{12}=\frac{m l^{2}}{12} \times \frac{1}{8}=\frac{I}{8} $$
Its time period of oscillation is
$$ M^{\prime}=M / 2 $$
$$ \begin{aligned} & T^{\prime}=2 \pi \sqrt{\frac{I^{\prime}}{M^{\prime} B}}=2 \pi \sqrt{\frac{I / 8}{(M / 2) B}}=\frac{2 \pi}{2} \sqrt{\frac{I}{M B}} \\ & T^{\prime}=\frac{T}{2} \end{aligned} $$
20. Use (i) the Ampere’s law for $H$ and (ii) continuity of lines of $\mathbf{B}$, to conclude that inside a bar magnet,
(a) lines of $\mathbf{H}$ run from the $N$-pole to $S$ - pole, while
(b) lines of $\mathbf{B}$ must run from the $S$-pole to $N$-pole.
Show Answer
Answer
Consider a magnetic field line of $\mathbf{B}$ through the bar magnet as given in the figure below.
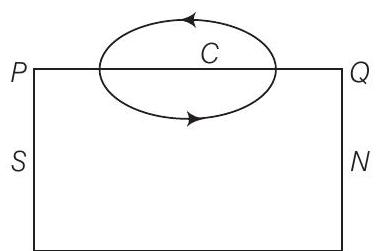
The magnetic field line of $B$ through the bar magnet must be a closed loop.
Let $C$ be the amperian loop. Then,
$$ \int_{Q}^{P} H \cdot d l=\int_{Q}^{P} \frac{B}{m_{0}} \cdot d l $$
We know that the angle between $\mathbf{B}$ and $\mathbf{d I}$ is less than $90^{\circ}$ inside the bar magnet. So, it is positive.
i.e.,
$$ \int_{Q}^{P} \mathrm{H} \cdot \mathrm{dl}=\int_{Q}^{P} \frac{\mathrm{B}}{\mu_{0}} \cdot \mathrm{dl}>0 $$
Hence, the lines of $\mathrm{B}$ must run from south pole $(S)$ to north pole $(N)$ inside the bar magnet.
According to Ampere’s law,
$$ \begin{array}{ll} \therefore & \oint_{P Q P} \mathrm{H} \cdot \mathrm{dl}=0 \\ \therefore & \oint_{P Q P} \mathrm{H} \cdot \mathrm{dl}=\int_{P}^{Q} \mathrm{H} \cdot \mathrm{dl}+\int_{Q}^{P} \mathrm{H} \cdot \mathrm{dl}=0 \\ \text { As } & \int_{Q}^{P} \mathrm{H} . \mathrm{dl}>0, \mathrm{so}, \int_{P}^{Q} \mathrm{H} \cdot \mathrm{dl}<0 \end{array} $$
It will be so if angle between $\mathbf{H}$ and $\mathbf{~ d l}$ is more than $90^{\circ}$, so that $\cos \theta$ is negative. It means the line of $\mathrm{H}$ must run from $\mathrm{N}$-pole to $\mathrm{S}$-pole inside the bar magnet.
Long Answer Type Questions
21. Verify the Ampere’s law for magnetic field of a point dipole of dipole
moment $\mathbf{M}=M \hat{\mathbf{k}}$. Take $C$ as the closed curve running clockwise along
(i) the $z$-axis from $z=a>0$ to $z=R$,
(ii) along the quarter circle of radius $R$ and centre at the origin in the first quadrant of $x z$-plane,
(iii) along the $x$-axis from $x=R$ to $x=a$, and
(iv) along the quarter circle of radius $a$ and centre at the origin in the first quadrant of $x z$-plane
Show Answer
Thinking Process
Let us consider the figure below

Answer
From $P$ to $Q$, every point on the $z$-axis lies at the axial line of magnetic dipole of moment $\mathbf{M}$. Magnetic field induction at a point distance $z$ from the magnetic dipole of moment is
$$ |\mathbf{B}|=\frac{\mu_{0}}{4 \pi} \frac{2|\mathbf{M}|}{z^{3}}=\frac{\mu_{0} M}{2 \pi z^{3}} $$
(i) Along z-axis from $P$ to $Q$.
$$ \begin{aligned} \int_{\rho}^{Q} B \cdot d l & =\int_{\rho}^{Q} B \cdot d l \cos 0^{\circ}=\int_{a}^{B} B d z \\ & =\int_{a}^{R} \frac{\mu_{0}}{2 \pi} \frac{M}{z^{3}} d z=\frac{\mu_{0} M}{2 \pi} \frac{-1}{2} \frac{1}{R^{2}}-\frac{1}{a^{2}} \\ & =\frac{\mu_{0} M}{4 \pi} \frac{1}{a^{2}}-\frac{1}{R^{2}} \end{aligned} $$
(ii) Along the quarter circle $Q S$ of radius $R$ as given in the figure below

The point $A$ lies on the equatorial line of the magnetic dipole of moment $M \sin \theta$. Magnetic field at point $A$ on the circular arc is
$$ \begin{aligned} B & =\frac{\mu_{0}}{4 \pi} \frac{M \sin \theta}{R^{3}} ; \mathrm{dl}=R d \theta \\ \therefore \quad & \int \mathrm{B} \cdot \mathrm{dl}=\int \mathrm{B} \cdot \mathrm{dl} \cos \theta=\int_{0}^{\frac{\pi}{2}} \frac{\mu_{0}}{4 \pi} \frac{M \sin \theta}{R^{3}} R d \theta \end{aligned} $$
Circular arc $=\frac{\mu_0}{4 \pi} \frac{M}{R}(-\cos \theta)_{0}^{\pi / 2}=\frac{\mu_0}{4 \pi} \frac{M}{R^{2}}$
(iii) Along $x$-axis over the path $S T$, consider the figure given ahead
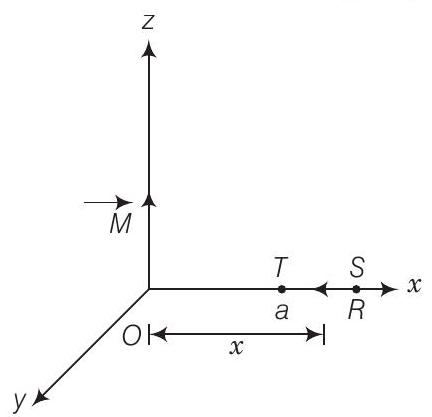
From figure, every point lies on the equatorial line of magnetic dipole. Magnetic field induction at a point distance $x$ from the dipole is
$$ \begin{aligned} & B=\frac{\mu_{0}}{4 \pi} \frac{M}{x^{3}} \\ & \therefore \quad \int_{\beta}^{T} \cdot \mathbf{d l}=\int_{R}^{a}-\frac{\mu_{0} \mathbf{M}}{4 \pi x^{3}} \cdot d \mathbf{d l}=0\left[\because \text { angle between }(-\mathbf{M}) \text { and } \mathbf{d l} \text { is } 90^{\circ}\right] \end{aligned} $$
(iv) Along the quarter circle TP of radius a. Consider the figure given below
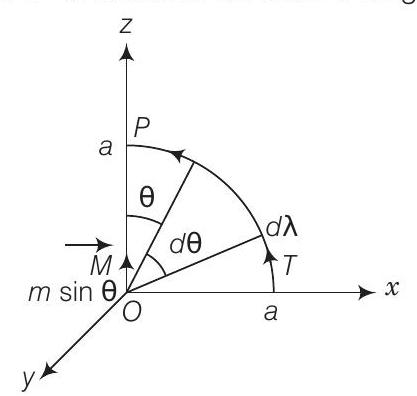
From case (ii), we get line integral of $\mathbf{B}$ along the quarter circle $T P$ of radius $a$ is circular arc TP
$$ \begin{aligned} & \int B \cdot dl \int_{\pi / 2}^0 \frac{\mu_0}{4 \pi} \frac{M \sin \theta}{a^{3}} ad \theta \\ & =\frac{\mu_0}{4 \pi} \frac{M}{a^{2}} \int_{\pi / 2}^0 \sin \theta d \theta=\frac{\mu_0}{4 \pi} \frac{M}{a^{2}}[-\cos \theta]_{\pi / 2}^0 \\ & =\frac{-\mu_0}{4 \pi} \frac{M}{a^{2}} \\ & =\frac{\mu_0 M}{4} \frac{1}{a^{2}}-\frac{1}{R^{2}}+\frac{\mu_0}{4 \pi} \frac{M}{R^{2}}+0+-\frac{\mu_0}{4 \pi} \frac{M}{a^{2}}=0 \end{aligned} $$
$$ \therefore \quad \oint_{P Q S T} B \cdot d l=\int_{P}^{Q} B \cdot d l+\int_{Q}^{S} B \cdot d l+\int_{S}^{T} B \cdot d l+\int_{T}^{P} B \cdot d l $$
22. What are the dimensions of $\chi$, the magnetic susceptibility? Consider an $\mathrm{H}$-atom. Gives an expression for $\chi$, upto a constant by constructing a quantity of dimensions of $\chi$, out of parameters of the atom $e, m, v, R$ and $\mu_{0}$. Here, $m$ is the electronic mass, $v$ is electronic velocity, $R$ is Bohr radius. Estimate the number so obtained and compare with the value of $|X| \sim 10^{-5}$ for many solid materials.
Show Answer
Thinking Process
Magnetic susceptibility is a measure of how a magnetic material responds to an external field. i.e., magnetic susceptibility
$$ \chi_{m}=\frac{I}{H}=\frac{(\text { Intensity of magnetisation) }}{\text { (Magnetising force) }} $$
Answer
As $I$ and $H$ both have same units and dimensions, hence, $\chi$ has no dimensions. Here, in this question, $\chi$ is to be related with $e, m, v, R$ and $\mu_{0}$. We know that dimensions of $\mu_{0}=\left[\mathrm{ML} \theta^{-2}\right]$
From Biot-Savart’s law,
$$ \begin{aligned} d B & =\frac{\mu_{0}}{4 \pi} \frac{I d l \sin \theta}{r^{2}} \\ \Rightarrow \quad \mu_{0} & =\frac{4 \pi r^{2} d B}{I d l \sin \theta}=\frac{4 \pi r^{2}}{I d l \sin \theta} \times \frac{f}{q v \sin \theta} \quad \because d B=\frac{F}{q v \sin \theta} \\ \therefore \quad \text { Dimensions of } \mu_{0} & =\frac{L^{2} \times\left(\mathrm{MLT}^{-2}\right)}{\left(\mathrm{QT}^{-1}\right)(\mathrm{L}) \times 1 \times(\mathrm{Q})\left(\mathrm{LT}^{-1}\right) \times(1)} \newline=\left[\mathrm{MLQ}^{-2}\right] \end{aligned} $$
where $Q$ is the dimension of charge.
As $\chi$ is dimensionless, it should have no involvement of charge $Q$ in its dimensional formula. It will be so if $\mu_{0}$ and $e$ together should have the value $\mu_{0} e^{2}$, as $e$ has the dimensions of charge.
Let
$$ \chi=\mu_{0} e^{2} m^{a} v^{b} R^{c} $$
where $a, b, c$ are the power of $m, v$ and $R$ respectively, such that relation (i) is satisfied.
Dimensional equation of (i) is
$$ \begin{aligned} {\left[\mathrm{M}^{0} \mathrm{~L}^{0} \mathrm{~T}^{0} \mathrm{Q}^{0}\right] } & =\left[\mathrm{ML} \mathrm{Q}^{-2}\right] \times\left[\mathrm{Q}^{2}\right]\left[\mathrm{M}^{a}\right] \times\left(\mathrm{LT}^{-1}\right)^{b} \times[\mathrm{L}]^{c} \\ & =\left[\mathrm{M}^{1+a}+\mathrm{L}^{1+b+c} \mathrm{~T}^{-b} \mathrm{Q}^{0}\right] \end{aligned} $$
Equating the powers of $M, L$ and $T$, we get
$$ \begin{aligned} & 0=1+a \Rightarrow a=-1,0=1+b+c \\ & 0=-b \Rightarrow b=0,0=1+0+c \text { or } c=-1 \end{aligned} $$
Putting values in Eq. (i), we get
$$ \begin{aligned} \chi & =\mu_{0} e^{2} m^{-1} v^{2} R^{-1}=\frac{\mu_{0} e^{2}}{m R} \\ \mu_{0} & =4 \pi \times 10^{-7} \mathrm{Tm} \mathrm{A}{ }^{-1} \\ e & =1.6 \times 10^{-19} \mathrm{C} \\ m & =9.1 \times 10^{-31} \mathrm{~kg}, R=10^{-10} \mathrm{~m} \\ \chi & =\frac{\left(4 \pi \times 10^{-7}\right) \times\left(1.6 \times 10^{-19}\right)^{2}}{\left(9.1 \times 10^{-31}\right) \times 10^{-10}} \approx 10^{-4} \end{aligned} $$
$$ \therefore \quad \frac{\chi}{\chi_{\text {(given solid) }}}=\frac{10^{-4}}{10^{-5}}=10 $$
23. Assume the dipole model for the earth’s magnetic field $B$ which is given by $B_{V}=$ vertical component of magnetic field $=\frac{\mu_{0}}{4 \pi} \frac{2 m \cos \theta}{r^{3}}$
$B_{H}=$ horizontal component of magnetic field $=\frac{\mu_{0}}{4 \pi} \frac{\sin \theta m}{r^{3}}$
$\theta=90^{\circ}$-lattitude as measured from magnetic equator.
Find loci of points for which (a) $|B|$ is minimum (b) dip angle is zero and (c) dip angle is $45^{\circ}$.
Show Answer
Answer
(a)
$$ \begin{aligned} B_{V} & =\frac{\mu_{0}}{4 \pi} \frac{2 m \cos \theta}{r^{3}} \\ B_{H} & =\frac{\mu_{0}}{4 \pi} \frac{\sin \theta m}{r^{3}} \end{aligned} $$
Squaring both the equations and adding, we get
$$ \begin{aligned} B_{V}^{2}+B_{H}^{2} & =\frac{\mu_{0}}{4 \pi} \frac{m^{2}}{r^{6}}\left[4 \cos ^{2} \theta+\sin ^{2} \theta\right] \\ B & =\sqrt{B_{V}^{2}+B_{H}^{2}}=\frac{\mu_{0}}{4 \pi} \frac{m}{r^{3}}\left[3 \cos ^{2} \theta+1\right]^{1 / 2} \end{aligned} $$
From Eq. (iii), the value of $B$ is minimum, if $\cos \theta=\frac{\pi}{2}$
$\theta=\frac{\pi}{2}$. Thus, the magnetic equator is the locus. (b) Angle of dip,
$$ \begin{aligned} & \tan \delta=\frac{B_{V}}{B_{H}}=\frac{\frac{\mu_{0}}{4 \pi} \cdot \frac{2 m \cos \theta}{r^{3}}}{\frac{\mu_{0}}{4 \pi} \cdot \frac{\sin \theta \cdot m}{r^{3}}}=2 \cot \theta \\ & \tan \delta=2 \cot \theta \end{aligned} $$
For dip angle is zero i.e., $\delta=0$
$$ \begin{aligned} \cot \theta & =0 \\ \theta & =\frac{\pi}{2} \end{aligned} $$
It means that locus is again magnetic equator.
(c) $\tan \delta=\frac{B_{V}}{B_{H}}$
Angle of dip i.e., $\delta= \pm 45$
$$ \begin{aligned} \frac{B_{V}}{B_{H}} & =\tan \left( \pm 45^{\circ}\right) \\ \frac{B_{V}}{B_{H}} & =1 \\ 2 \cot \theta & =1 \\ \cot \theta & =\frac{1}{2} \\ \Rightarrow \quad \tan \theta & =2 \\ \theta \quad \theta & =\tan ^{-1}(2) \end{aligned} $$
Thus, $\theta=\tan ^{-1}(2)$ is the locus.
24. Consider the plane $S$ formed by the dipole axis and the axis of earth. Let $P$ be point on the magnetic equator and in $S$. Let $Q$ be the point of intersection of the geographical and magnetic equators. Obtain the declination and dip angles at $P$ and $Q$.
Show Answer
Answer
$P$ is in the plane $S$, needle is in north, so the declination is zero.

$P$ is also on the magnetic equator, so the angle of dip $=0$, because the value of angle of dip at equator is zero. $Q$ is also on the magnetic equator, thus the angle of dip is zero. As earth tilted on its axis by $11.3^{\circ}$, thus the declination at $Q$ is $11.3^{\circ}$.
25. There are two current carrying planar coil made each from identical wires of length $L . C_{1}$ is circular (radius $R$ ) and $C_{2}$ is square (side $a$ ). They are so constructed that they have same frequency of oscillation when they are placed in the same uniform $\mathbf{B}$ and carry the same current. Find $a$ in terms of $R$.
Show Answer
Answer
$C_{1}=$ circular coil of radius $R$, length $L$, number of turns per unit length
$$ n_{1}=\frac{L}{2 \pi R} $$
$C_{2}=$ square of side $a$ and perimeter $L$, number of turns per unit length $n_{2}=\frac{L}{4 a}$

Magnetic moment of $C_{1}$
$\Rightarrow$ Magnetic moment of $C_{2}$
$$ m_{1}=n_{1} I A_{1} $$
$\Rightarrow$
$$ \begin{aligned} m_{2} & =n_{2} I A_{2} \\ m_{1} & =\frac{L \cdot I \cdot \pi R^{2}}{2 \pi R} \\ m_{2} & =\frac{L}{4 a} \cdot I \cdot a^{2} \\ m_{1} & =\frac{L I R}{2} \\ m_{2} & =\frac{L I a}{4} \end{aligned} $$
Moment of inertia of $C_{1} \Rightarrow I_{1}=\frac{M R^{2}}{2}$
Moment of inertia of $C_{2} \Rightarrow I_{2}=\frac{M a^{2}}{12}$
Frequency of $C_{1} \Rightarrow t_{1}=2 \pi \sqrt{\frac{I_{1}}{m_{1} B}}$
Frequency of $C_{2} \Rightarrow f_{2}=2 \pi \sqrt{\frac{I_{2}}{m_{2} B}}$
According to question, $f_{1}=f_{2}$
$$ \begin{aligned} 2 \pi \sqrt{\frac{I_{1}}{m_{1} B}} & =2 \pi \sqrt{\frac{I_{2}}{m_{2} B}} \\ \frac{I_{1}}{m_{1}} & =\frac{I_{2}}{m_{2}} \text { or } \frac{m_{2}}{m_{1}}=\frac{I_{2}}{I_{1}} \end{aligned} $$
Plugging the values by Eqs. (i), (ii), (iii) and (iv)
$$ \begin{aligned} \frac{L I a \cdot 2}{4 \times L I R} & =\frac{M a^{2} \cdot 2}{12 \cdot M R^{2}} \\ \frac{a}{2 R} & =\frac{a^{2}}{6 R^{2}} \\ 3 R & =a \end{aligned} $$
Thus, the value of $a$ is $3 R$.










