Chapter 08 Electromagnetic Waves
Multiple Choice Questions (MCQs)
1. One requires $11 eV$ of energy to dissociate a carbon monoxide molecule into carbon and oxygen atoms. The minimum frequency of the appropriate electromagnetic radiation to achieve the dissociation lies in
(a) visible region
(c) ultraviolet region
(b) infrared region
(d) microwave region
Show Answer
Answer
(c) Given, energy required to dissociate a carbon monoxide molecule into carbon and oxygen atoms $E=11 eV$
We know that, $E=h \nu$, where $h=6.62 \times 10^{-34} J$-s
$$ \begin{aligned} \Rightarrow \quad 11 eV & =h \nu \\ v & =\frac{11 \times 1.6 \times 10^{-19}}{h} J \\ & =\frac{11 \times 1.6 \times 10^{-19}}{6.62 \times 10^{-34}} J \\ & =2.65 \times 10^{15} Hz \end{aligned} $$
$$ \nu=\text { frequency } $$
This frequency radiation belongs to ultraviolet region.
2. A linearly polarised electromagnetic wave given as $\mathbf{E}=E_0 \hat{\mathbf{i}} \cos (k z-\omega t)$ is incident normally on a perfectly reflecting infinite wall at $z=a$. Assuming that the material of the wall is optically inactive, the reflected wave will be given as $\newline$
(a)$ r=E_{o} \hat{i}(k z-\omega t)$ $\newline$
(b)$ r=E_{o} \hat{i} \cos (k z+\omega t)$ $\newline$
(c)$ r=-E_{o} \hat{i} \cos (k z+\omega t)$ $\newline$
(d)$ r=E_{o} \hat{i} \sin (k z-\omega t)$ $\newline$
Show Answer
Thinking Process
When a wave is reflected from a denser medium, then its phase changes by $180^{\circ}$ or $\pi$
Answer
(b) When a wave is reflected from denser medium, then the type of wave doesn’t change but only its phase changes by $180^{\circ}$ or $\pi$ radian.
Thus, for the reflected wave $\hat{\mathbf{z}}=-\hat{\mathbf{z}}, \hat{\mathbf{i}}=-\hat{\mathbf{i}}$ and additional phase of $\pi$ in the incident wave.
Given, here the incident electromagnetic wave is,
$$ \mathbf{E}=E_0 \hat{\mathbf{i}} \cos (k z-\omega t) $$
The reflected electromagnetic wave is given by
$$ \begin{aligned} r & =E_0(\hat{i}) \cos [k(-z)-\omega t+\pi] \\ & =-E_0 \hat{i} \cos [-(k z+\omega t)+\pi] \\ & =E_0 \hat{i} \cos [-(k_{z}+\omega t)=E_0 \hat{\mathbf{i}} \cos (k z+\omega t)] \end{aligned} $$
3. Light with an energy flux of $20 W / cm^{2}$ falls on a non-reflecting surface at normal incidence. If the surface has an area of $30 cm^{2}$, the total momentum delivered (for complete absorption) during $30 min$ is $\newline$
(a) $36 \times 10^{-5} kg-m / s$ $\newline$
(b) $36 \times 10^{-4} kg-m / s$ $\newline$
(c) $108 \times 10^{4} kg-m / s$ $\newline$
(d) $1.08 \times 10^{7} kg-m / s$ $\newline$
Show Answer
Answer
(b) Given, energy flux $\phi=20 W / cm^{2}$
Area, $\quad A=30 cm^{2}$
Time, $\quad t=30 min=30 \times 60 s$
Now, total energy falling on the surface in time $t$ is, $U=\phi A t=20 \times 30 \times(30 \times 60) J$
Momentum of the incident light $=\frac{U}{C}$
$$ =\frac{20 \times 30 \times(30 \times 60)}{3 \times 10^{8}} \Rightarrow=36 \times 10^{-4} kg-ms^{-1} $$
Momentum of the reflected light $=0$
$\therefore$ Momentum delivered to the surface
$$ =36 \times 10^{-4}-0=36 \times 10^{-4} kg-ms^{-1} $$
4. The electric field intensity produced by the radiations coming from $100 W$ bulb at a $3 m$ distance is $E$. The electric field intensity produced by the radiations coming from $50 W$ bulb at the same distance is
(a) $\frac{E}{2}$
(b) $2 E$
(c) $\frac{E}{\sqrt{2}}$
(d) $\sqrt{2} E$
Show Answer
Thinking Process
Electric field intensity on a surface due to incident radiation is,
$$ \begin{aligned} I_{av} \propto E_o^2 \\ \frac{P_{av .}}{A} \propto E_o^2 \\ \end{aligned} $$
Here,
$$ P_{a v} \propto E_0^{2} \quad[\because A \text { is same in bothcases }] $$
Answer
(c) We know that,
$$ E_0 \propto \sqrt{P_{av}} $$
$\frac{(E_O)_1}{(E_O)_2} = \sqrt{\frac{(P_a{av})_1} {(P_a{av})_2} \Rightarrow \frac{E}{E_O}_2} = \sqrt{\frac{1000}{5}} \\ $
according to question, P=50 W, P=100 W
$\therefore \quad$ Putting these value in Eq.(i), we get
$$ \frac{E^{\prime}}{E}=\frac{50}{100} \Rightarrow \frac{E^{\prime}}{E}=\frac{1}{2} \Rightarrow E^{\prime}=\frac{E}{2} $$
5. If $\mathbf{E}$ and $\mathbf{B}$ represent electric and magnetic field vectors of the electromagnetic wave, the direction of propagation of electromagnetic wave is along
(a) $\mathbf{E}$
(b) $\mathbf{B}$
(c) $B \times E$
(d) $\mathbf{E} \times \mathbf{B}$
Show Answer
Answer
(d) The direction of propagation of electromagnetic wave is perpendicular to both electric field vector $\mathbf{E}$ and magnetic field vector $\mathbf{B}$, i.e., in the direction of $\mathbf{E} \times \mathbf{B}$.
This can be seen by the diagram given below
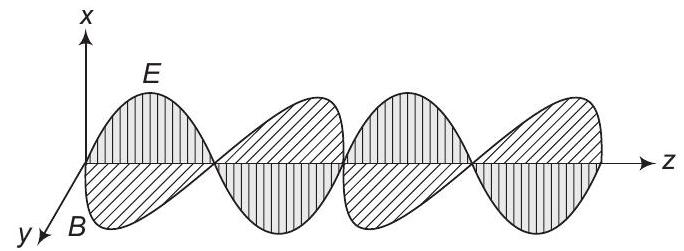
Here, electromagnetic wave is along the $z$-direction which is given by the cross product of $\mathbf{E}$ and $\mathbf{B}$.
6. The ratio of contributions made by the electric field and magnetic field components to the intensity of an EM wave is
(a) $c: 1$
(b) $c^{2}: 1$
(c) $1: 1$
(d) $\sqrt{c}: 1$
Show Answer
Thinking Process
Intensity of electromagnetic wave, $I=U_{a v} c$
where, $\quad U_{a v}=$ Average energy
and $\quad c=$ speed to light
Answer
(c) Intensity in terms of electric field $U_{av}=\frac{1}{2} \varepsilon_0 E_0^{2}$
Intensity in terms of magnetic field $U_{av}=\frac{1}{2} \frac{B_0^{2}}{\mu_0}$
Now taking the intensity in terms of electric field.
$$ \quad(U_{\text{av}}) \text{ electric field } =\frac{1}{2} E_0^{2} $$ $$=\frac{1}{2} (c B_0)^{2} \quad(\because E_0=c B_0) =\frac{1}{2} \times c^{2} B^{2} $$
$$\text { But, } \quad =\frac{1}{\sqrt{\mu_0 }} \therefore\quad(U_{\text{av}})_{\text {Electric field }}$$
Thus, the energy in electromagnetic wave is divided equally between electric field vector and magnetic field vector
Therefore, the ratio of contributions by the electric field and magnetic field components to the intensity of an electromagnetic wave is $1: 1$.
7. An EM wave radiates outwards from a dipole antenna, with $E_{o}$ as the amplitude of its electric field vector. The electric field $E_0$ which transports significant energy from the source falls off as $\newline$
(a) $\frac{1}{r^{3}}$ $\newline$
(b) $\frac{1}{r^{2}}$ $\newline$
(c) $\frac{1}{r}$ $\newline$
(d) remains constant $\newline$
Show Answer
Answer
(c) From a diode antenna, the electromagnetic waves are radiated outwards.
The amplitude of electric field vector $(E_0)$ which transports significant energy from the source falls off intensity inversely as the distance $(r)$ from the antenna, i.e., $E_0 \propto \frac{1}{r}$.
8. An electromagnetic wave travels in vacuum along $z$-direction $\mathbf{E}=(E_1 \hat{\mathbf{i}} E_2 \hat{\mathbf{j}}) \cos (k z-\omega t)$. Choose the correct options from the following
(a) The associated magnetic field is given as
$$ \mathbf{B}=\frac{1}{C}(E_1 \hat{\mathbf{i}}-E_2 \hat{\mathbf{j}}) \cos (k z-\omega t) $$
(b) The associated magnetic field is given as
$$ \mathbf{B}=\frac{1}{C}(E_1 \hat{\mathbf{i}}-E_2 \hat{\mathbf{j}}) \cos (k z-\omega t) $$
(c) The given electromagnetic field is circularly polarised
(d) The given electromagnetic wave is plane polarised
Show Answer
Thinking Process
From Maxwell’s equations, it is seen that the magnitude of the electric and the magnetic fields in an electromagnetic wave are related as
$$ B_0=\frac{E_0}{c} $$
Answer
(d) Here, in electromagnetic wave, the electric field vector is given as,
$$ \mathbf{E}=(E_1 \hat{\mathbf{i}}+E_2 \hat{\mathbf{j}}) \cos (k z-\omega t) $$
In electromagnetic wave, the associated magnetic field vector,
$$ \mathbf{B}=\frac{E}{C}=\frac{E_1 \hat{i}+E_2 \hat{j}}{c} \cos (k z-\omega t) $$
Also, $\mathbf{E}$ and $\mathbf{B}$ are perpendicular to each other and the propagation of electromagnetic wave is perpendicular to $\mathbf{E}$ as well as $\mathbf{B}$, so the given electromagnetic wave is plane polarised.
Multiple Choice Questions (More Than One Options)
9. An electromagnetic wave travelling along $z$-axis is given as $\mathbf{E}=\mathbf{E}_{0} \cos (k z-\omega t)$. Choose the correct options from the following
(a) The associated magnetic field is given as $B=\frac{1}{C} \hat{\mathbf{k}} \times \mathbf{E}=\frac{1}{\omega}(\hat{\mathbf{k}} \times \mathbf{E})$
(b) The electromagnetic field can be written in terms of the associated magnetic field as $\mathbf{E}=c(\mathbf{B} \times \hat{\mathbf{k}})$
(c) $\hat{\mathbf{k}} \cdot \mathbf{E}=0, \hat{\mathbf{k}} \cdot \mathbf{B}=0$
(d) $\hat{\mathbf{k}} \times \mathbf{E}=0, \hat{\mathbf{k}} \times \mathbf{B}=0$
Show Answer
Thinking Process
Given, $E=E_{0} \cos (k z-w t)$. Thus, it acts along negative y-direction.
Answer
$(a, b, c)$
Suppose an electromagnetic wave is travelling along negative z-direction. Its electric field is given by
$$ \mathbf{E}=E_{O} \cos (k z-\omega t) $$
which is perpendicular to $z$-axis. It acts along negative $y$-direction.
The associated magnetic field $\mathbf{B}$ in electromagnetic wave is along $x$-axis i.e., along $\hat{\mathbf{k}} \times \mathbf{E}$.
$$ \begin{array}{ll} \text { As, } & B_{0}=\frac{E_{0}}{C} \\ \therefore & B=\frac{1}{C}(\hat{\mathbf{k}} \times \mathbf{E}) \end{array} $$
The associated electric field can be written in terms of magnetic field as
$$ \mathbf{E}=c(\mathbf{B} \times \hat{\mathbf{k}}) $$
Angle between $\hat{\mathbf{k}}$ and $\mathbf{E}$ is $90^{\circ}$ between $\hat{\mathbf{k}}$ and $\mathbf{B}$ is $90^{\circ}$. Therefore, $\mathbf{E}=1 E \cos 90^{\circ}=0$ and $\hat{\mathbf{k}} \cdot \mathbf{B}=1 E \cos 90^{\circ}=0$
10. A plane electromagnetic wave propagating along $x$-direction can have the following pairs of $\mathbf{E}$ and $\mathbf{B}$. $\newline$
Show Answer
Answer
$(b, d)$ $\newline$
(a) $E_{x}, B_{y}$ $\newline$
(b) $E_{y}, B_{z}$ $\newline$
(c) $B_{x}, E_{y}$ $\newline$
(d) $E_{z}, B_{y}$ $\newline$
As electric and magnetic field vectors $\mathbf{E}$ and $\mathbf{B}$ are perpendicular to each other as well as perpendicular to the direction of propagation of electromagnetic wave.
Here in the question electromagnetic wave is propagating along $x$-direction. So, electric and magnetic field vectors should have either $y$-direction or $z$-direction. $\newline$
11. A charged particle oscillates about its mean equilibrium position with a frequency of $10^{9} \mathrm{~Hz}$. The electromagnetic waves produced $\newline$
(a) will have frequency of $10^{9} \mathrm{~Hz}$ $\newline$
(b) will have frequency of $2 \times 10^{9} \mathrm{~Hz}$ $\newline$
(c) will have wavelength of $0.3 \mathrm{~m}$ $\newline$
(d) fall in the region of radiowaves $\newline$
Show Answer
Thinking Process
The frequency of electromagnetic waves produced by a charged particle is equal to the frequency by which it oscillates about its mean equilibrium position.
Answer
$(a, c, d)$
Given, frequency by which the charged particles oscillates about its mean equilibrium position $=10^{9} \mathrm{~Hz}$.
So, frequency of electromagnetic waves produced by the charged particle is $v=10^{9} \mathrm{~Hz}$.
$$ \text { Wavelength } \lambda=\frac{c}{v}=\frac{3 \times 10^{8}}{10^{9}}=0.3 \mathrm{~m} $$
Also, frequency of $10^{9} \mathrm{~Hz}$ fall in the region of radiowaves.
12. he source of electromagnetic waves can be a charge
(a) moving with a constant velocity
(b) moving in a circular orbit
(c) at rest
(d) falling in an electric field
Show Answer
Thinking Process
An electromagnetic wave can be produced by accelerated or oscillating charge.
Answer
( $b, d)$
Here, in option (b) charge is moving in a circular orbit.
In circular motion, the direction of the motion of charge is changing continuously, thus it is an accelerated motion and this option is correct.
Also, we know that a charge starts accelerating when it falls in an electric field.
13. An EM wave of intensity $I$ falls on a surface kept in vacuum and exerts radiation pressure $p$ on it. Which of the following are true?
(a) Radiation pressure is $\frac{I}{\mathrm{C}}$ if the wave is totally absorbed
(b) Radiation pressure is $\frac{I}{\mathrm{C}}$ if the wave is totally reflected
(c) Radiation pressure is $\frac{2 I}{\mathrm{C}}$ if the wave is totally reflected
(d) Radiation pressure is in the range $\frac{I}{\mathrm{C}}<p<\frac{2 I}{\mathrm{C}}$ for real surfaces
Show Answer
Answer
$(a, c, d)$
Radiation pressure $(p)$ is the force exerted by electromagnetic wave on unit area of the surface, i.e., rate of change of momentum per unit area of the surface.
Momentum per unit time per unit area
$$ =\frac{\text { Intensity }}{\text { Speed of wave }}=\frac{I}{\mathrm{c}} $$
Change in momentum per unit time per unit area $=\frac{\Delta I}{C}=$ radiation pressure $(p)$ i.e.,
$$ p=\frac{\Delta I}{c} $$
Momentum of incident wave per unit time per unit area $=\frac{I}{c}$
When wave is fully absorbed by the surface, the momentum of the reflected wave per unit time per unit area $=0$.
Radiation pressure $(p)=$ change in momentum per unit time per unit area $=\frac{\Delta I}{c}=\frac{I}{c}-0=\frac{I}{c}$.
When wave is totally reflected, then momentum of the reflected wave per unit time per unit area $=-\frac{I}{\mathrm{c}}$, Radiation pressure $p=\frac{I}{\mathrm{c}}–\frac{I}{\mathrm{c}}=\frac{2 I}{\mathrm{c}}$.
Here, $p$ lies between $\frac{I}{c}$ and $\frac{2 I}{c}$.
Very Short Answer Type Questions
14. Why is the orientation of the portable radio with respect to broadcasting station important?
Show Answer
Answer
The orientation of the portable radio with respect to broadcasting station is important because the electromagnetic waves are plane polarised, so the receiving antenna should be parallel to the vibration of the electric or magnetic field of the wave.
15. Why does microwave oven heats up a food item containing water molecules most efficiently?
Show Answer
Answer
Microwave oven heats up the food items containing water molecules most efficiently because the frequency of microwaves matches the resonant frequency of water molecules.
16. The charge on a parallel plate capacitor varies as $q=q_{0} \cos 2 \pi v t$. The plates are very large and close together (area $=A$, separation $=d$ ). Neglecting the edge effects, find the displacement current through the capacitor.
Show Answer
Answer
The displacement current through the capacitor is,
Here,
Putting this value in $\mathrm{Eq}$ (i), we get
$$ I_{d}=I_{C}=\frac{d q}{d t} $$
$$ \begin{aligned} & I_{d}=I_{c}=-q_{0} \sin 2 \pi v t \times 2 \pi v \\ & I_{d}=I_{c}=-2 \pi v q_{0} \sin 2 \pi v t \end{aligned} $$
17. A variable frequency $A C$ source is connected to a capacitor. How will the displacement current change with decrease in frequency?
Show Answer
Thinking Process
Capacities reactance $X_{c}$ is inversely proportional to the displacement current i.e., $X_{c} \propto \frac{1}{I}$.
Answer
Capacitive reaction $X_{C}=\frac{1}{2 \pi f C}$,
$\therefore \quad X_{c} \propto \frac{1}{f}$
As frequency decreases, $X_{C}$ increases and the conduction current is inversely proportional to $X_{c} \because I \propto \frac{1}{X_{c}}$
So, displacement current decreases as the conduction current is equal to the displacement current.
18. The magnetic field of a beam emerging from a filter facing a floodlight is given by
$$ B_{0}=12 \times 10^{-8} \sin \left(1.20 \times 10^{7} z-3.60 \times 10^{15} t\right) \mathrm{T} \text {. } $$
What is the average intensity of the beam?
Show Answer
Answer
Magnetic field $\mathbf{B}=B_{0}$ sin $\omega t$
Given, equation $B=12 \times 10^{-8} \sin \left(1.20 \times 10^{7} z-3.60 \times 10^{15} t\right) \mathrm{T}$.
On comparing this equation with standard equation, we get
$$ B_{0}=12 \times 10^{-8} $$
The average intensity of the beam $I_{\mathrm{av}}=\frac{1}{2} \frac{B_{0}^{2}}{\mu_{0}} \cdot \mathrm{C}=\frac{1}{2} \times \frac{\left(12 \times 10^{-8}\right)^{2} \times 3 \times 10^{8}}{4 \pi \times 10^{-7}}$
$$ =1.71 \mathrm{~W} / \mathrm{m}^{2} $$
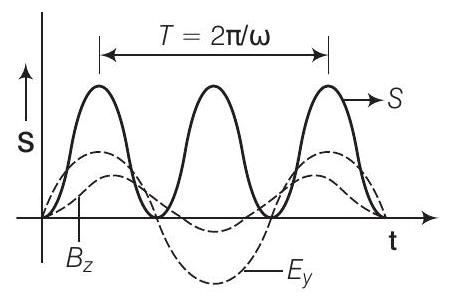
19. Poynting vectors $S$ is defined as a vector whose magnitude is equal to the wave intensity and whose direction is along the direction of wave propogation. Mathematically, it is given by $\mathbf{S}=\frac{1}{\mu_{0}} \mathbf{E} \times \mathbf{B}$. Show the nature of $\mathbf{S}$ versus $t$ graph.
Show Answer
Answer
Consider and electromagnetic waves, let $\mathbf{E}$ be varying along $y$-axis, $\mathbf{B}$ is along z-axis and propagation of wave be along $x$-axis. Then $\mathbf{E} \times \mathbf{B}$ will tell the direction of propagation of energy flow in electromegnetic wave, along $x$-axis.
Let
$$ \begin{aligned} \mathbf{E} & =E_{0} \sin (\omega t-k x) \hat{\mathbf{j}} \\ \mathbf{B} & =B_{0} \sin (\omega t-k x) \hat{\mathbf{k}} \\ \mathbf{S} & =\frac{1}{\mu_{0}}(\mathbf{E} \times \mathbf{B})=\frac{1}{\mu_{0}} E_{0} B_{0} \sin ^{2}(\omega t-k x)[\hat{\mathbf{j}} \times \hat{\mathbf{k}}] \\ & =\frac{E_{0} B_{0}}{\mu_{0}} \sin ^{2}(\omega t-k x) \hat{\mathbf{i}} \end{aligned} $$
The variation of $|\mathbf{S}|$ with time $t$ will be as given in the figure below
20. Professor CV Raman surprised his students by suspending freely a tiny light ball in a transparent vacuum chamber by shining a laser beam on it. Which property of EM waves was he exhibiting? Give one more example of this property.
Show Answer
Answer
An electromagnetic wave carries energy and momentum like other waves.
Since, it carries momentum, an electromagnetic wave also exerts pressure called radiation pressure. This property of electromagnetic waves helped professor CV Raman surprised his students by suspending freely a tiny light ball in a transparent vacuum chamber by shining a laser beam on it. The tails of the camets are also due to radiation pressure.
Short Answer Type Questions
21. Show that the magnetic field $B$ at a point in between the plates of a parallel plate capacitor during charging is $\frac{\mu_{o} \varepsilon_{0} r}{2} \frac{d E}{d t}$ (symbols having usual meaning).
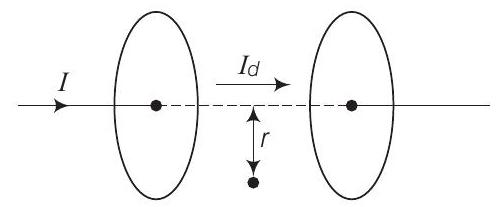
Show Answer
Answer
Consider the figure ginen below to prove that the magneti field $\mathrm{B}$ at a point in between the plater of a paravel- plate copocior during charging is $\frac{\varepsilon_{0} \mu_{0} r}{2} \frac{d E}{d t}$
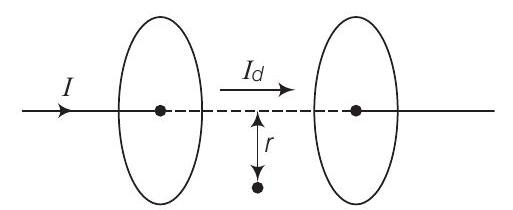
Let $I_{d}$ be the displacement current in the region between two plates of parallel plate capacitor, in the figure.
The magnetic field induction at a point in a region between two plates of capacitor at a perpendicular distance $r$ from the axis of plates is
$$ \begin{array}{rlrl} B & =\frac{\mu_{0} 2 I_{d}}{4 \pi r}=\frac{\mu_{0}}{2 \pi r} I_{d}=\frac{\mu_{0}}{2 \pi r} \times \varepsilon_{0} \frac{d \varphi_{E}}{d t} & & \because I_{d}=\frac{E_{0} d \varphi_{E}}{d t} \\ & =\frac{\mu_{0} \varepsilon_{0}}{2 \pi r} \frac{d}{d t}\left(E r^{2}\right)=\frac{\mu_{0} \varepsilon_{0}}{2 \pi r} \pi^{2} \frac{d E}{d t} & \\ B & =\frac{\mu_{0} \varepsilon_{0} r}{2} \frac{d E}{d t} & & {\left[\because \varphi_{E}=E r^{2}\right]} \end{array} $$
22. Electromagnetic waves with wavelength
(i) $\lambda_{1}$ is used in satellite communication.
(ii) $\lambda_{2}$ is used to kill germs in water purifies.
(iii) $\lambda_{3}$ is used to detect leakage of oil in underground pipelines.
(iv) $\lambda_{4}$ is used to improve visibility in runways during fog and mist conditions.
(a) Identify and name the part of electromagnetic spectrum to which these radiations belong.
(b) Arrange these wavelengths in ascending order of their magnitude.
(c) Write one more application of each.
Show Answer
Answer
(a) (i) Microwave is used in satellite communications.
So, $\lambda_{1}$ is the wavelength of microwave.
(ii) Ultraviolet rays are used to kill germs in water purifier. So, $\lambda_{2}$ is the wavelength of UV rays.
(iii) $X$-rays are used to detect leakage of oil in underground pipelines. So, $\lambda_{3}$ is the wavelength of X-rays.
(iv) Infrared is used to improve visibility on runways during fog and mist conditions. So, it is the wavelength of infrared waves.
(b) Wavelength of X-rays $<$ wavelength of UV $<$ wavelength of infrared $<$ wavelength of microwave.
$$ \Rightarrow \quad \lambda_{3}<\lambda_{2}<\lambda_{4}<\lambda_{1} $$
(c) (i) Microwave is used in radar.
(ii) UV is used in LASIK eye surgery.
(iii) $X$-ray is used to detect a fracture in bones.
(iv) Infrared is used in optical communication.
23. Show that average value of radiant flux density $S$ over a single period $T$ is given by $S=\frac{1}{2 c \mu_{0}} E_{0}^{2}$.
Show Answer
Answer
Radiant flux density $S=\frac{1}{\mu_{0}}(\mathbf{E} \times \mathbf{B})=c^{2} \varepsilon_{0}(\mathbf{E} \times \mathbf{B})$
$$ \because c=\frac{1}{\sqrt{\mu_{0} \varepsilon_{0}}} $$
Suppose electromagnetic waves be propagating along $x$-axis. The electric field vector of electromagnetic wave be along $y$-axis and magnetic field vector be along $z$-axis. Therefore,
and $$ E_0 =E_0 \cos(k x-\omega t) $$ $$ {B} =B_0 \cos(k x-\omega t) \\ $$
$$E \times B =\left(E_0 \times B_0\right) \cos^{2}(k x-\omega t) \\ $$ $$S =c^{2}0(E \times B) \\ =c^{2}0\left(E_0 \times B_0\right) \cos^{2}(k x-\omega t) $$
Average value of the magnitude of radiant flux density over complete cycle is
$$ \begin{array}{rlrl} S_{av} =c^{2} 0\left|E_0 \times B_0\right| \frac{1}{T} \int_{0}^{T} \cos ^{2}(k x-\omega t) d t \newline \\ =c^{2} 0 E_{0} B_{0} \times \frac{1}{T} \times \frac{T}{2} \because \int_{0}^{T} \cos ^{2}(k x-\omega t) d t=\frac{T}{2} \newline \\ \Rightarrow S_{av}=\frac{c^{2}}{2} 0 E_{0} \frac{E_{0}}{c} \text { As, } c=\frac{E_{0}}{B_{0}} \newline \\ =\frac{c}{2} 0 E_{0}^{2}=\frac{c}{2} \times \frac{1}{c^{2} \mu_{0}} E_{0}^{2} c=\frac{1}{\sqrt{\mu_{0} 0}} \text { or } 0=\frac{1}{c^{2} \mu_{0}} \newline \\ \Rightarrow \quad S_{av} =\frac{E_{0}^{2}}{2 \mu_{0} c} \newline\text { Hence proved. } \end{array} $$
24. You are given a $2 \mu \mathrm{F}$ parallel plate capacitor. How would you establish an instantaneous displacement current of $1 \mathrm{~mA}$ in the space between its plates?
Show Answer
Answer
Given, capacitance of capacitor $C=2 \mu \mathrm{F}$,
Displacement current $I_{d}=1 \mathrm{~mA}$ Charge
or
$$ \begin{aligned} q & =C V \\ I_{d} d t & =C d V \\ I_{d} & =C \frac{d V}{d t} \\ 1 \times 10^{-3} & =2 \times 10^{-6} \times \frac{d V}{d t} \\ \frac{d V}{d t} & =\frac{1}{2} \times 10^{+3}=500 \mathrm{~V} / \mathrm{s} \end{aligned} \quad[\because q=i t] $$
$$ \text { or } $$
So, by applying a varying potential difference of $500 \mathrm{~V} / \mathrm{s}$, we would produce a displacement current of desired value.
25. Show that the radiation pressure exerted by an EM wave of intensity $I$ on a surface kept in vacuum is $\frac{I}{C}$.
Show Answer
Answer
Pressure $=\frac{\text { Force }}{\text { Area }}=\frac{F}{A}$
Force is the rate of change of momentum
i.e.,
$$ F=\frac{d p}{d t} $$
Energy in time $d t$,
$$ U=p \cdot \operatorname{Cor} p=\frac{U}{C} $$
$\therefore \quad$ Pressure $=\frac{1}{A} \cdot \frac{U}{C \cdot d t}$
$$ \text { Pressure }=\frac{I}{C} \quad \because I=\text { Intensity }=\frac{U}{A \cdot d t} $$
26. What happens to the intensity of light from a bulb if the distance from the bulb is doubled? As a laser beam travels across the length of room, its intensity essentially remain constant.
What geometrical characteristic of LASER beam is responsible for the constant intensity which is missing in the case of light from the bulb?
Show Answer
Answer
As the distance is doubled, the area of spherical region $\left(4 \pi^{2}\right)$ will become four times, so the intensity becomes one fourth the initial value $\because I \propto \frac{1}{r^{2}}$ but in case of laser it does not spread, so its intensity remain same.
Geometrical characteristic of LASER beam which is responsible for the constant intensity are as following
(i) Unidirection
(ii) Monochromatic
(iii) Coherent light
(iv) Highly collimated
These characteristic are missing in the case of light from the bulb.
27. Even though an electric field $\mathrm{E}$ exer ts a force $q \mathrm{E}$ on a charged particle yet electric field of an EM wave does not contribute to the radiation pressure (but transfers energy). Explain.
Show Answer
Answer
Since, electric field of an EM wave is an oscillating field and so is the electric force caused by it on a charged particle. This electric force averaged over an integral number of cycles is zero, since its direction changes every half cycle.
Hence, electric field is not responsible for radiation pressure.
Long Answer Type Questions
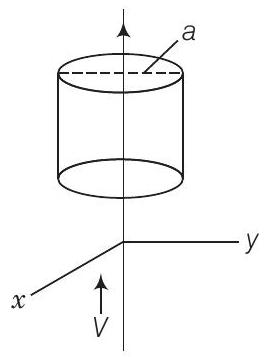
28. An infinitely long thin wire carrying a uniform linear static charge density $\lambda$ is placed along the $z$-axis (figure). The wire is set into motion along its length with a uniform velocity $v=v \hat{k}_{z}$. Calculate the pointing vector $S=\frac{1}{0}(E \times B)$.
Show Answer
Answer
Given,
$$ \begin{aligned} B & =\frac{\mu_{0} i}{2 \pi a} \hat{i}=\frac{\mu_{0} \lambda v}{2 \pi a} \hat{i} \newline\\ \therefore \quad S & =\frac{1}{\mu_{0}}[E \times B]=\frac{1}{\mu_{0}} \frac{\lambda_{\hat{j}}}{2 \pi \varepsilon_{0} a} \times \frac{\mu_{0}}{2 \pi a} \lambda \sqrt{\hat{i}} \newline\\ & =\frac{\lambda^{2} v}{4 \pi^{2} \varepsilon_{0} a^{2}}(\hat{j} \times \hat{i})=-\frac{\lambda^{2} V}{4 \pi^{2} \varepsilon_{0} a^{2}} \hat{k} \end{aligned} \quad\left[\because I=\lambda_{V}\right] $$
$$ E=\frac{\lambda \hat{e}_{s}}{2 \pi 0 a} \hat{j} $$
29. Sea water at frequency $v=4 \times 10^{8} \mathrm{~Hz}$ has permittivity $\varepsilon \approx 80 \varepsilon_{0}$, permeability $\mu \approx \mu_{0}$ and resistivity $\rho=0.25 \mathrm{~m}$. Imagine a parallel plate capacitor immersed in sea water and driven by an alternating voltage source $V(t)=V_{0} \sin (2 \pi v t)$. What fraction of the conduction current density is the displacement current density?
Show Answer
Thinking Process
The conduction current density is given by the Ohm’s law = Electric field between the plates.
Answer
Suppose distance between the parallel plates is $d$ and applied voltage $V_{(t)}=V_{0} 2 \pi v t$.
Thus, electric field
Now using Ohm’s law,
$$ \begin{aligned} E=\frac{V_{0}}{d} \sin (2 \pi v t) \newline \\ J_{c} =\frac{1}{\rho} \frac{V_{0}}{d} \sin (2 \pi v t) \newline \\ =\frac{V_{0}}{\rho d} \sin (2 \pi v t)= \newline \\ J_{0}^{c} =\frac{V_{0}}{\rho d} \end{aligned} $$
$$ \Rightarrow \quad=\frac{V_{0}}{\rho d} \sin (2 \pi v t)=J_{0}^{c} \sin 2 \pi v t $$
Here,
Now the displacement current density is given as
$$ \begin{array}{rlrl} J_{d} =\varepsilon \frac{\delta E}{d t}=\frac{\varepsilon \delta}{d t} \newline \\ =\frac{\varepsilon 2 \pi v V_{0}}{d} \cos (2 \pi v t) \newline \\ \Rightarrow =J_{0}^{d} \cos (2 \pi v t) \newline \\ \text { where, } J_{0}^{d} =\frac{2 \pi V \varepsilon V_{0}}{d} \newline \\ \Rightarrow \frac{J_{0}^{d}}{J_{0}^{c}} =\frac{2 \pi v \varepsilon V_{0}}{d} \cdot \frac{\rho d}{V_{0}}=2 \pi v \varepsilon \rho \newline \\ =2 \pi \times 80 \varepsilon_{0} v \times 0.25=4 \pi \varepsilon_{0} v \times 10 \newline \\ =\frac{10 v}{9 \times 10^{9}}=\frac{4}{9} \end{array} $$
30. A long straight cable of length $l$ is placed symmetrically along $z$-axis and has radius $a(«l)$. The cable consists of a thin wire and a co-axial conducting tube. An alternating current $I(t)=I_{0} \sin (2 \pi v t)$ flows down the central thin wire and returns along the co-axial conducting tube. The induced electric field at a distance $s$ from the wire inside the cable is
$$ E(s, t)=\mu_0 I_0 v \cos (2 \pi v t) \ln \frac sa \hat{k} $$
(i) Calculate the displacement current density inside the cable.
(ii) Integrate the displacement current density across the cross-section of the cable to find the total displacement current $I^{d}$.
(iii) Compare the conduction current $I_{0}$ with the displacement current $I_{0}^{d}$.
Show Answer
Thinking Process
Displacement current density
$$ J_{d}=\varepsilon_{0} \frac{dE}{d t} $$
Answer
(i) Given, the induced electric field at a distance $r$ from the wire inside the cable is
$$ \mathbf{E}(s, t)=\mu_{0} I_{0} v \cos (2 \pi v t) \ln \frac sa \hat{\mathbf{k}} $$
Now, displacement current density,
$$ Jd=\varepsilon_{0} \frac{d \mathbf{E}}{d t}=\varepsilon_{0} \frac{d}{d t} \mu_{0} I_{0} v \cos (2 \pi v t) \ln \frac{s}{a} \hat{\mathbf{k}} $$
(ii)
$\begin{aligned} I _d & =\int J _d s d s d \theta=\int _{s=0}^a J _d s d s \int _0^{2 \pi} d \theta=\int _{s=0}^a J _d s d s \\ & =\int _{s=0}^a[\frac{2 \pi}{\lambda^2} I_0 \log _e(\frac{a}{s}) s d s \sin 2 \pi v t] \times 2 \pi \\ & =(\frac{2 \pi}{\lambda})^2 I _0 \int _{s=0}^a(\frac{a}{s}) s d s \sin 2 \pi v t \\ \Rightarrow \qquad & =(\frac{2 \pi}{\lambda})^2 I _0 \int _{s=0}^a \ln (\frac{a}{s}) \frac{1}{2} d(s^2) \cdot \sin 2 \pi v t \end{aligned}$
$\begin{aligned} & =\frac{a^2}{2}\left(\frac{2 \pi}{\lambda}\right)^2 I_0 \sin 2 \pi v t \int_{s=0}^a \ln \left(\frac{a}{s}\right) \cdot d\left(\frac{s}{a}\right)^2 \\ & =\frac{a^2}{4}\left(\frac{2 \pi}{\lambda}\right)^2 I_0 \sin 2 \pi v t \int_{s=0}^a \ln \left(\frac{a}{s}\right)^2 \cdot d\left(\frac{s}{a}\right)^2 \\ & =-\frac{a^2}{4}\left(\frac{2 \pi}{\lambda}\right)^2 I_0 \sin 2 \pi v t \int_{s=0}^a \ln \left(\frac{s}{a}\right)^2 \cdot d\left(\frac{s}{a}\right)^2 \end{aligned}$
$\begin{array}{rlrl} & =-\frac{a^2}{4}\left(\frac{2 \pi}{\lambda}\right)^2 I_0 \sin 2 \pi v t \times(-1) & {\left[\because \int_{s=0}^a \ln \left(\frac{s}{a}\right)^2 d\left(\frac{s}{a}\right)^2=-1\right]} \\ \therefore & I_d =\frac{a^2}{4}\left(\frac{2 \pi}{\lambda}\right)^2 I_0 \sin 2 \pi v t \\ \Rightarrow \qquad & =\left(\frac{2 \pi a}{2 \lambda}\right)^2 I_0 \sin 2 \pi v t & \end{array}$
(iii) The displacement current,
$$ \begin{aligned} & I_{d}=\frac{2 \pi a^{2}}{2 \lambda} I_{0} \sin 2 \pi v t=I_{0 d} \sin 2 \pi v t \\ \text { Here, } & I_{0 d}=\frac{2 \pi a}{2 \lambda}^{2} I_{0}=\frac{a \pi^{2}}{\lambda} I_{0} \\ \therefore & \frac{I_{0 d}}{I_{0}}=\frac{a \pi^{2}}{\lambda} \end{aligned} $$
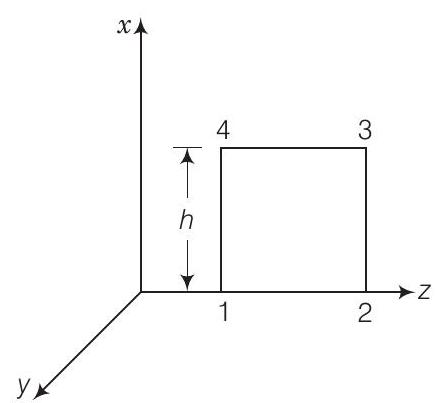
31. A plane $E M$ wave travelling in vacuum along $z$-direction is given by $\mathbf{E}=E_{0} \sin (k z-\omega t) \hat{\mathbf{i}}$ and $\mathbf{B}=B_{0} \sin (k z-\omega t) \hat{\mathbf{j}}$.
(i) Evaluate $\int \mathbf{E} \cdot \mathbf{d l}$ over the rectangular loop 1234 shown in figure.
(ii) Evaluate $\int \mathbf{B} \cdot \mathbf{d s}$ over the surface bounded by loop 1234.
(iii) Use equation $\int \mathbf{E} \cdot \mathbf{d} \mathbf{l}=\frac{-d \varphi_{B}}{d t}$ to prove $\frac{E_{0}}{B_{0}}=c$.
(iv) By using similar process and the equation
$$ \int \mathbf{B} \cdot \mathbf{d l}=\mu_{0} I+\varepsilon_{0} \frac{d \varphi_{E}}{d t}, \text { prove that } c=\frac{1}{\sqrt{\mu_{0} \varepsilon_{0}}} $$
Show Answer
Answer
(i) Consider the figure given below

During the propagation of electromagnetic wave a long $z$-axis, let electric field vector $(\mathbf{E})$ be along $x$-axis and magnetic field vector $\mathbf{B}$ along $y$-axis, i.e., $\mathbf{E}=E_{0} \hat{\mathbf{i}}$ and $\mathbf{B}=B_{0} \hat{\mathbf{j}}$.
Line integral of $E$ over the closed rectangular path 1234 in $x$ - $z$ plane of the figure
$$ \begin{aligned} g \mathbf{E} \cdot \mathbf{d l} & =\int_{1}^{2} E \cdot d l+\sqrt{2} \cdot \frac{3}{E} \cdot d l+\int_{3}^{4} \cdot d l+\int_{4}^{1} E \cdot d l \newline \\ & =\int_{1}^{2} E \cdot d l \cos 90+\int_{2}^{3} E \cdot d l \cos 0+\int_{3}^{4} E \cdot d l \cos 90+\int_{4}^{1} E \cdot d l \cos 180^{\circ} \newline \\ & =E_{0} h\left[\sin \left(k z_{2}-\omega t\right)-\sin \left(k z_{1}-g \omega t\right)\right] \end{aligned} $$
(ii) For evaluating $\boldsymbol{\beta} \mathbf{B} \cdot \mathbf{d s}$, let us consider the rectangle 1234 to be made of strips of are $d s=h d z$ each.
$$ \begin{aligned} \int \mathbf{B} \cdot \mathbf{d} \mathbf{s}=\int \mathbf{B} \cdot \mathbf{d} \mathbf{s} \cos 0 & =\int \mathbf{B} \cdot \mathbf{d} \mathbf{s}=\int_{z_{1}}^{z_{2}} B_{0} \sin (k z-\omega t) h d z \\ & =\frac{-B_{0} h}{k}\left[\cos \left(k z_{2}-\omega t\right)-\cos \left(k z_{1}-\omega t\right)\right] \end{aligned} $$

(iii) Given, $g \mathbf{E} \cdot dl=\frac{-d \varphi_{B}}{d t}=-\frac{d}{d t} g \mathbf{B} \cdot \mathbf{d s}$
Putting the values from Eqs. (i) and (ii), we get
$$ E_{0} h [\sin k z_{2}-\omega t-\sin k z_{1}-\omega t] \\ $$
$$= \frac{-d}{d t} \frac{B_{y} h}{k}t\cos k z_{2}-\omega t-\cos k z_{1}-\omega t. \\ $$ $$= \frac{B_y h}{k} \omega \sin k z_2-\omega t-\sin k z_{1}-\omega t $$
$$\Rightarrow \quad E_{0}=\frac{B_{0} \omega}{k}=B_{y} c \because \frac{\omega}{k}=c$$ $$\Rightarrow \quad \frac{E_0}{B_0}= C$$
(iv) For evaluating $g^{\mathbf{B}} \cdot \mathbf{d l}$, let us consider a loop 1234 in $y$-z plane as shown in figure given below
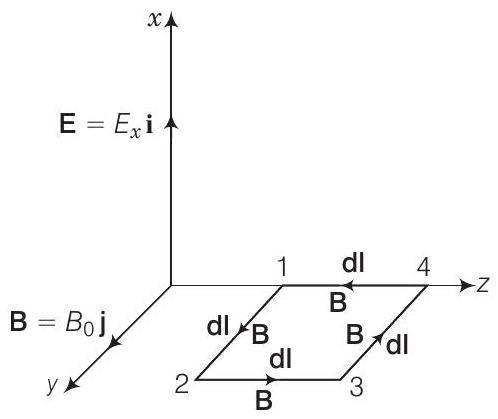
$$ \begin{aligned} \int \mathbf{B} \cdot \mathbf{d l} & =\int_{1}^{2} \mathbf{B} \cdot \mathbf{d l}+\int_{2}^{3} \mathbf{B} \cdot \mathbf{d l}+\int_{3}^{4} \mathbf{B} \cdot \mathbf{d l}+\int_{4}^{1} \mathbf{B} \cdot \mathbf{d l}\newline \\ & =\int_{1}^{2} \mathbf{B} \cdot \mathbf{d l} \cos 0+\int_{2}^{3} \mathbf{B} \cdot \mathbf{d l} \cos 90^{\circ}+\int_{3}^{4} \mathbf{B} \cdot \mathbf{d l} \cos 180^{\circ}+\int_{4}^{1} \mathbf{B} \cdot \mathbf{d l} \cos 90^{\circ}\newline \\ & =B_{0} h\left[\sin \left(k z_{1}-\omega t\right)-\sin \left(k z_{2}-\omega t\right)\right. \end{aligned} $$
Now to evaluate $\varphi_{E}=\int \mathbf{E} \cdot \mathbf{d s}$, let us consider the rectangle 1234 to be made of strips of area $h d_{2}$ each.
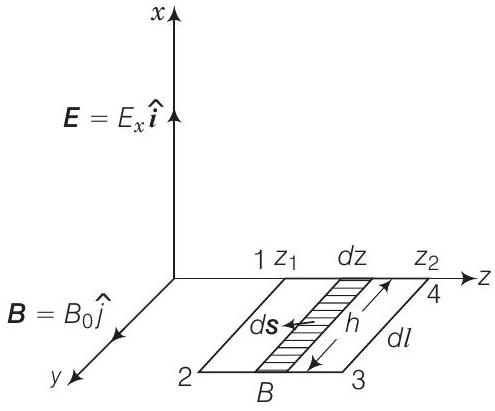
$$ \begin{aligned} \varphi_{E} & =\int E \cdot d s=\int E d s \cos 0=\int E d s=\int_{z_{1}}^{z_{0}^{2}} E_{0} \sin \left(k z_{1}-\omega t\right) h d z \\ & =-\frac{E_{0} h}{k}\left[\cos \left(k z_{2}-\omega t\right)-\cos \left(k z_{1}-\omega t\right)\right] \\ \therefore \quad \frac{d \varphi_{E}}{d t} & =\frac{E_{0} h \omega}{k}\left[\sin \left(k z_{1}-\omega t\right)-\sin \left(k z_{2}-\omega t\right)\right] \end{aligned} $$
$$ \begin{aligned} \text { In } \quad g \mathbf{B} \cdot \mathbf{d l} & =\mu_{0} I+\frac{\varepsilon_{0} d \varphi_{E}}{d t}, I=\text { conduction current } \\ & =0 \text { in vacuum } \\ \therefore \quad \quad \quad g \mathbf{B} \cdot \mathrm{dl} & =\mu_{0} \varepsilon \frac{d \varphi_{E}}{d t} \end{aligned} $$
Using relations obtained in Eqs. (iii) and (iv) and simplifying, we get
$$ \begin{array}{rlrl} B_{0} =E_{0} \frac{\omega \mu_{0} \varepsilon_{0}}{k} \newline \\ \Rightarrow \frac{E_{0}}{B_{0}} \frac{\omega}{k} =\frac{1}{\mu_{0} \varepsilon_{0}} \newline \\ \text { But } \frac{E_{0}}{B_{0}} =c \text { and } \omega=c k \newline \\ \Rightarrow c \cdot c =\frac{1}{\mu_{0} \varepsilon_{0}}, \text { therefore } c=\frac{1}{\sqrt{\mu_{0} \varepsilon_{0}}} \end{array} $$
32. $\mathrm{~A}$ plane $E M$ wave travelling along $z$-direction is described by $\mathbf{E}=E_{0} \sin (k z-\omega t) \hat{\mathbf{i}}$ and $\mathbf{B}=B_{0} \sin (k z-\omega t) \hat{\mathbf{j}}$. Show that
(i) the average energy density of the wave is given by
$$ u_{\mathrm{av}}=\frac{1}{4} \varepsilon_{0} E_{0}^{2}+\frac{1}{4} \frac{B_{0}^{2}}{\mu_{0}} $$
(ii) the time averaged intensity of the wave is given by
$$ I_{\mathrm{av}}=\frac{1}{2} c \varepsilon_{0} E_{0}^{2} . $$
Show Answer
Answer
(i) The electromagnetic wave carry energy which is due to electric field vector and magnetic field vector. In electromagnetic wave, $E$ and $B$ vary from point to point and from moment to moment. Let $E$ and $B$ be their time averages.
The energy density due to electric field $E$ is
$$ u_{E}=\frac{1}{2} \varepsilon_{0} E^{2} $$
The energy density due to magnetic field $B$ is
$$ u_{B}=\frac{1}{2} \frac{B^{2}}{\mu_{0}} $$
Total average energy density of electromagnetic wave
$$ u_{a v}=u_{E}+u_{B}=\frac{1}{2} \varepsilon_{0} E^{2}+\frac{1}{2} \frac{B^{2}}{\mu_{0}} $$
Let the EM wave be propagating along z-direction. The electric field vector and magnetic field vector be represented by
$$ \begin{aligned} & E=E_{0} \sin (k z-\omega t) \\ & B=B_{0} \sin (k z-\omega t) \end{aligned} $$
The time average value of $E^{2}$ over complete cycle $=\frac{E_{0}^{2}}{2}$
and time average value of $B^{2}$ over complete cycle $=\frac{B_{0}^{2}}{2}$
$$ \begin{aligned} u_{\mathrm{av}} & =\frac{1}{2} \frac{\varepsilon_{0} E_{0}^{2}}{2}+\frac{1}{2} \mu_{0} \frac{B_{0}^{2}}{2} \\ & =\frac{1}{4} \varepsilon_{0} E_{0}^{2}+\frac{B_{0}^{2}}{4 \mu_{0}} \end{aligned} $$
(ii) We know that $E_{0}=c B_{0}$ and $c=\frac{1}{\sqrt{\mu_{0} \varepsilon_{0}}}$
$$ \begin{array}{rlrl} \therefore \frac{1}{4} \frac{B_{0}^{2}}{\mu_{0}} =\frac{1}{4} \frac{E_{0}^{2} / c^{2}}{\mu_{0}}=\frac{E_{0}^{2}}{4 \mu_{0}} \times \mu_{0} \varepsilon_{0}=\frac{1}{4} \varepsilon_{0} E_{0}^{2} \newline\\ \therefore u_{B} =u_{E} \newline\\ \text { Hence, } u_{\mathrm{av}} =\frac{1}{4} \varepsilon_{0} E_{0}^{2}+\frac{1}{4} \frac{B_{0}^{2}}{\mu_{0}} \newline\\ =\frac{1}{4} \varepsilon_{0} E_{0}^{2}+\frac{1}{4} \varepsilon_{0} E_{0}^{2} \newline\\ =\frac{1}{2} \varepsilon_{0} E_{0}^{2}=\frac{1}{2} \frac{B_{0}^{2}}{\mu_{0}} \end{array} $$
Time average intensity of the wave
$$ I_{\mathrm{av}}=u_{\mathrm{av}} \mathrm{c}=\frac{1}{2} \varepsilon_{0} E_{0}^{2} \mathrm{C}=\frac{1}{2} \varepsilon_{0} E_{0}^{2} $$










