Chapter 01 Electric Charges and Field
Multiple Choice Questions (MCQs)
1. In figure two positive charges $q_{2}$ and $q_{3}$ fixed along the $y$-axis, exert a net electric force in the $+x$-direction on a charge $q_{1}$ fixed along the $x$-axis. If a positive charge $Q$ is added at $(x, 0)$, the force on $q_{1}$
(i)
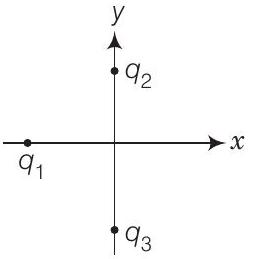
(ii)
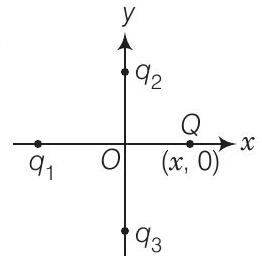
(a) shall increase along the positive $x$-axis
(b) shall decrease along the positive $x$-axis
(c) shall point along the negative $x$-axis
(d) shall increase but the direction changes because of the intersection of $Q$ with $q_{2}$ and $q_{3}$
Show Answer
Thinking Process
Find the nature of force between $q_{1}-q_{2}$ and $a_{1}-q_{3}$. Nature of force will give the type of charge $a_{1}$. Find the nature of force between newly introduced charge and charge $q_{1}$.
Answer
(a) The net force on $q_{1}$ by $q_{2}$ and $q_{3}$ is along the $+x$-direction, so nature of force between $q_{1}, q_{2}$ and $q_{1}, q_{3}$ is attractive. This can be represent by the figure given below
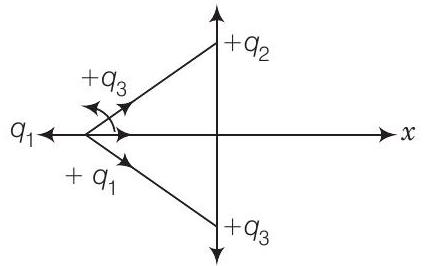
The attractive force between these charges states that $q_{1}$ is a negative charge (since, $q_{2}$ and $q_{3}$ are positive).
Thus, nature of force between $q_{1}$ and newly introduced charge $Q$ (positive) is attractive and net force on $q_{1}$ by $q_{2}, q_{3}$ and $Q$ are along the same direction as given in the diagram below
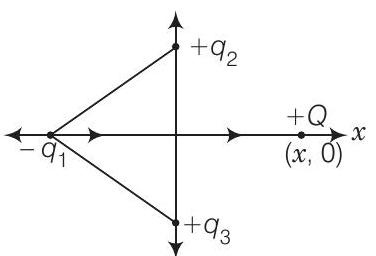
The figure given above clearly shows that the force on $q_{1}$ shall increase along the positive $x$-axis due to the positive charge $Q$.
Note Unlike charges repel each other and like charges attract each other.
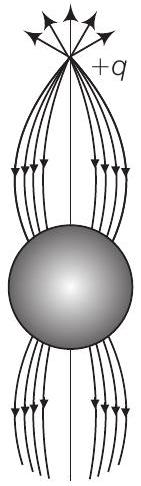
2. A point positive charge is brought near an isolated conducting sphere (figure). The electric field is best given by
(a)
(b)
(c)
(d)
Show Answer
Thinking Process
Bringing the point positive charge towards the conducting sphere, charges the sphere by induction process. Electric field lines passes through a charged body following some rules.
Answer
(a) When a positive point charge is brought near an isolated conducting sphere without touching the sphere, then the free electrons in the sphere are attracted towards the positive charge. This leaves an excess of positive charge on the rear (right) surface of sphere.
Both kinds of charges are bound in the metal sphere and cannot escape. They, therefore, reside on the surface.
Thus, the left surface of sphere has an excess of negative charge and the right surface of sphere has an excess of positive charge as given in the figure below
attracted negative
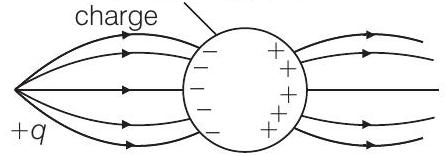
An electric field lines start from positive charge and ends at negative charge (in this case from point positive charge to negative charge created inside the sphere).
Also, electric field line emerges from a positive charge, in case of single charge and ends at infinity.
Here, all these conditions are fulfilled in Fig. (a).
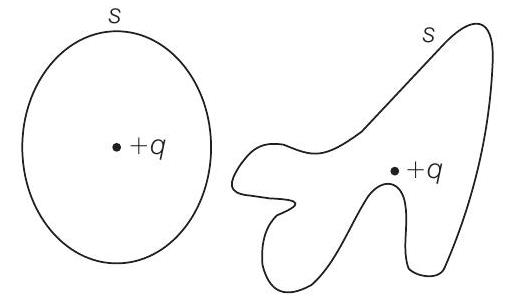
3. The electric flux through the surface
(i)
(ii)
(iv)
(a) in Fig. (iv) is the largest
(b) in Fig. (iii) is the least
(c) in Fig. (ii) is same as Fig. (iii) but is smaller than Fig. (iv)
(d) is the same for all the figures
Show Answer
Answer
(d) Gauss’ law of electrostatics state that the total of the electric flux out of a closed surface is equal to the charge enclosed decided by the permittivity i.e., $Q$ electric $=\frac{Q}{\varepsilon_{0}}$.
Thus, electric flux through a surface doesn’t depend on the shape, size or area of a surface but it depends on the number of charges enclosed by the surface.
So, here in this question, all the figures same electric flux as all of them has single positive charge.
4. Five charges $q_{1}, q_{2}, q_{3}, q_{4}$, and $q_{5}$ are fixed at their positions as shown in Figure, $S$ is a Gaussian surface. The Gauss’ law is given by $\int_{5} E . d S=\frac{q}{\varepsilon_{0}}$. Which of the following statements is correct?
(a) $\mathbf{E}$ on the LHS of the above equation will have a contribution from $q_{1}, q_{5}$ and $q_{1}, q_{5}$ and $q_{3}$ while $q$ on the RHS will have a contribution from $q_{2}$ and $q_{4}$ only
(b) $\mathbf{E}$ on the LHS of the above equation will have a contribution from all charges while $q$ on the RHS will have a contribution from $q_{2}$ and $q_{4}$ only

(c) $\mathbf{E}$ on the LHS of the above equation will have a contribution from all charges while $q$ on the RHS will have a contribution from $q_{1}, q_{3}$ and $q_{5}$ only
(d) Both $\mathbf{E}$ on the LHS and $q$ on the RHS will have contributions from $q_{2}$ and $q_{4}$ only
Show Answer
Answer
(b) According to Gauss’ law, the term $q$ on the right side of the equation $\int_{S} E \cdot d S=\frac{q}{\varepsilon_{0}}$ includes the sum of all charges enclosed by the surface.
The charges may be located anywhere inside the surface, if the surface is so chosen that there are some charges inside and some outside, the electric field on the left side of equation is due to all the charges, both inside and outside $S$.
So, $\mathrm{E}$ on LHS of the above equation will have a contribution from all charges while $q$ on the RHS will have a contribution from $q_{2}$ and $q_{4}$ only.
5. Figure shows electric field lines in which an electric dipole $P$ is placed as shown. Which of the following statements is correct?
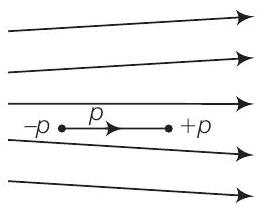
(a) The dipole will not experience any force
(b) The dipole will experience a force towards right
(c) The dipole will experience a force towards left
(d) The dipole will experience a force upwards
Show Answer
Thinking Process
Find the electric field strength on the charges of dipole.
Force varies directly with electric field strength i.e., higher the electric field strength greater the force and vice-versa.
Answer
(c) The space between the electric field lines is increasing, here from left to right and its characteristics states that, strength of electric field decreases with the increase in the space between electric field lines. As a result force on charges also decreases from left to right.
Thus, the force on charge $-q$ is greater than force on charge $+q$ in turn dipole will experience a force towards left.
6. A point charge $+q$ is placed at a distance $d$ from an isolated conducting plane. The field at a point $P$ on the other side of the plane is
(a) directed perpendicular to the plane and away from the plane
(b) directed perpendicular to the plane but towards the plane
(c) directed radially away from the point charge
(d) directed radially towards the point charge
Show Answer
Answer
(a) When a point positive charge brought near an isolated conducting plane, some negative charge developes on the surface of the plane towards the charge and an equal positive charge developes on opposite side of the plane. This process is called charging by induction.
7. A hemisphere is uniformely charged positively. The electric field at a point on a diameter away from the centre is directed
(a) perpendicular to the diameter
(b) parallel to the diameter
(c) at an angle tilted towards the diameter
(d) at an angle tilted away from the diameter
Show Answer
Answer
(a) When the point is situated at a point on diameter away from the centre of hemisphere charged uniformly positively, the electric field is perpendicular to the diameter. The component of electric intensity parallel to the diameter cancel out.
Multiple Choice Questions (More Than One Options)
8. If $\int_{5} E . d S=0$ over a surface, then
(a) the electric field inside the surface and on it is zero
(b) the electric field inside the surface is necessarily uniform
(c) the number of flux lines entering the surface must be equal to the number of flux lines leaving it
(d) all charges must necessarily be outside the surface
Thinking Process
Go through Gauss’ law in detail.
Show Answer
Answer
(c, $d)$
$\oint_{S} E . d S=0$ represents electric flux over the closed surface.
In general, $\oint_{S}$ E.dS means the algebraic sum of number of flux lines entering the surface and number of flux lines leaving the surface.
When $g_{S} E . d S=0$, it means that the number of flux lines entering the surface must be equal to the number of flux lines leaving it.
Now, from Gauss’ law, we know that $g_{S} E . d S=\frac{q}{\varepsilon_{0}}$ where $q$ is charge enclosed by the surface. When $\int_{S} E \cdot d S=0, q=0$ i.e., net charge enclosed by the surface must be zero. Therefore, all other charges must necessarily be outside the surface. This is because charges outside because of the fact that charges outside the surface do not contribute to the electric flux.
9. The electric field at a point is
(a) always continuous
(b) continuous if there is no charge at that point
(c) discontinuous only if there is a negative charge at that point
(d) discontinuous if there is a charge at that point
Show Answer
Answer
$(b, d)$
The electric field due to a charge $Q$ at a point in space may be defined as the force that a unit positive charge would experience if placed at that point. Thus, electric field due to the charge $Q$ will be continuous, if there is no charge at that point. It will be discontinuous if there is a charge at that point.
10. If there were only one type of charge in the universe, then
(a) $\oint_{S} \mathbf{E} . \mathbf{d S} \neq 0$ on any surface
(b) $\oint_{S} \mathbf{E} \cdot \mathbf{d S}=0$ if the charge is outside the surface
(c) $\oint_{S} \mathbf{E} \cdot \mathbf{d S}$ could not be defined
(d) $\oint_{S} \mathbf{E} \cdot \mathbf{d} \mathbf{S}=\frac{q}{\varepsilon_{0}}$ if charges of magnitude $q$ were inside the surface
Show Answer
Answer
(c, $d)$
Gauss’ law states that $\oint_{s} E . d S=\frac{q}{\varepsilon_{0}}$, where $q$ is the charge enclosed by the surface. If the charge is outside the surface, then charge enclosed by the surface is $q=0$ and thus, $\oint_{s}$ E.dS $=0$. Here, electric flux doesn’t depend on the type or nature of charge.
11. Consider a region inside which there are various types of charges but the total charge is zero. At points outside the region,
(a) the electric field is necessarily zero
(b) the electric field is due to the dipole moment of the charge distribution only
(c) the dominant electric field is $\propto \frac{1}{r_{3}}$, for large $r$, where $r$ is the distance from a origin in this regions
(d) the work done to move a charged particle along a closed path, away from the region, will be zero
Show Answer
Answer
(c, $d)$
When there are various types of charges in a region, but the total charge is zero, the region can be supposed to contain a number of electric dipoles.
Therefore, at points outside the region (may be anywhere w.r.t. electric dipoles), the dominant electric field $\propto \frac{1}{r_{3}}$ for large $r$.
Further, as electric field is conservative, work done to move a charged particle along a closed path, away from the region will be zero.
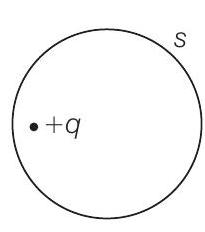
12. Refer to the arrangement of charges in figure and a Gaussian surface of radius $R$ with $Q$ at the centre. Then,
(a) total flux through the surface of the sphere is $\frac{-Q}{\varepsilon_{0}}$
(b) field on the surface of the sphere is $\frac{-Q}{4 \pi \varepsilon_{0} R^{2}}$
(c) flux through the surface of sphere due to $5 Q$ is zero
(d) field on the surface of sphere due to $-2 \mathrm{Q}$ is same everywhere

Show Answer
Answer
$(a, c)$
Gauss’ law states that total electric flux of an enclosed surface is given by $\frac{q}{\varepsilon_{0}}$ where $q$ is the charge enclosed by the surface. Thus, from figure,
Total charge inside the surface is $=Q-2 Q=-Q$
$\therefore$ Total flux through the surface of the sphere $=\frac{-Q}{\varepsilon_{0}}$
Now, considering charge $5 Q$. Charge $5 Q$ lies outside the surface, thus it makes no contribution to electric flux through the given surface.
13. A positive charge $Q$ is uniformly distributed along a circular ring of radius $R$.A small test charge $q$ is placed at the centre of the ring figure. Then,
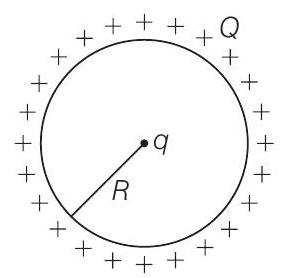
(a) if $q>0$ and is displaced away from the centre in the plane of the ring, it will be pushed back towards the centre
(b) if $q<0$ and is displaced away from the centre in the plane of the ring, it will never return to the centre and will continue moving till it hits the ring
(c) if $q<0$, it will perform SHM for small displacement along the axis
(d) $q$ at the centre of the ring is in an unstable equilibrium within the plane of the ring for $q>0$
Show Answer
Answer
$(a, b, c)$
The positive charge $Q$ is uniformly distributed at the outer surface of the enclosed sphere. Thus, electric field inside the sphere is zero.
So, the effect of electric field on charge $q$ due to the positive charge $Q$ is zero.
Now, the only governing factor is the attractive and repulsive forces between charges
$(Q$ and $q)$ there are two cases arise.
Case I When charge $q>0$ i.e., $q$ is a positive charge, there creates a repulsive force between charge $q$ and $Q$.
The repulsive forces of charge $Q$ from all around the charge $q$ will push it towards the centre if it is displaced from the centre of the ring.
Case II When charge $q<0$ i.e., $q$ is a negative charge then there is an attractive force between charge $Q$ and $q$
If $q$ is shifted from the centre, then the positive charges nearer to this charge will attract it towards itself and charge $q$ will never return to the centre.
Very Short Answer Type Questions
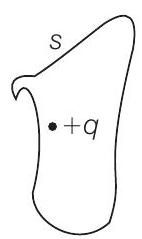
14. An arbitrary surface encloses a dipole. What is the electric flux through this surface?
Answer From Gauss’ law, the electric flux through an enclosed surface is given by $\oint_{s} E \cdot d S=\frac{q}{\varepsilon_{0}}$.
Here, $q$ is the net charge inside that enclosed surface. Now, the net charge on a dipole is given by $-q+q=0$ $\therefore \quad$ Electric flux through a surface enclosing a dipole $=\frac{-q+q}{\varepsilon_{0}}=\frac{0}{\varepsilon_{0}}=0$Show Answer
15. A metallic spherical shell has an inner radius $R_{1}$ and outer radius $R_{2}$. A charge $Q$ is placed at the centre of the spherical cavity. What will be surface charge density on $\newline$
(i) the inner surface $\newline$
(ii) the outer surface? $\newline$
Show Answer
Thinking Process
Let us draw the diagram as per the given situation. Using the induction process of charging distribute the charge on whole spherical shell. Now, find the required surface charge density.
Answer
Here, the charge placed at the centre of the spherical cavity is positively charged. So, the charge created at the inner surface of the sphere, due to induction will be $-Q$ and due to this charge created at outer surface of the sphere is $+Q$.
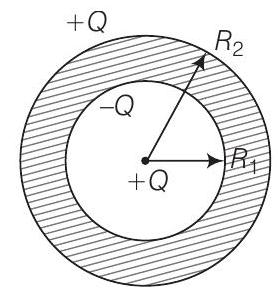
Now, surface charge density on the inner surface $=\frac{-Q}{4 \pi R_{1}^{2}}$ and
Surface charge density on the inner surface $=\frac{+Q}{4 \pi R_{2}^{2}}$
16. The dimensions of an atom are of the order of an Angstrom. Thus, there must be large electric fields between the protons and electrons. Why, then is the electrostatic field inside a conductor zero?
Show Answer
Answer
The protons and electrons are bound into a atom with distinct and independent existence and neutral in charge.
Electrostatic fields are caused by the presence of excess charges.
But there can be no excess charge on the inter surface of an isolated conductor. So, the electrostatic fields inside a conductor is zero despite the fact that the dimensions of an atom are of the order of an Angstrom.
17. If the total charge enclosed by a surface is zero, does it imply that the elecric field everywhere on the surface is zero? Conversely, if the electric field everywhere on a surface is zero, does it imply that net charge inside is zero.
Show Answer
Answer
Gauss’ law also implices that when the surface is so chosen that there are some chargas inside and some outside.
The flux in such situation is given by $\oint$ E.dS $=\frac{q}{\varepsilon_{0}}$.
In such situations, the electric field in the LHS is due to all the charges both inside and outside the surface. The term $q$ on the right side of the equation given by Gauss’ law represent only the total charge inside the surface.
Thus, despite being total charge enclosed by a surface zero, it doesn’t imply that the electric field everywhere on the surface is zero, the field may be normal to the surface.
Also, conversely if the electric field everywhere on a surface is zero, it doesn’t imply that net charge inside it is zero.
i.e.,
we get
$$ \text { Putting } E=0 \text { in } g \text { E.dS }=\frac{q}{\varepsilon_{0}} $$
$$ q=0 . $$
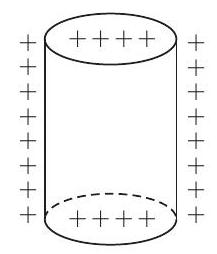
18. Sketch the electric field lines for a uniformly charged hollow cylinder shown in figure.
Show Answer
Thinking Process
According to general properties, electric field lines start from positive charges and end at negative charges. If there is a single charge, they may start or end at infinity.
Answer
Thus, the electric field lines will start from positive charges and move towards infinity as given in the figure below
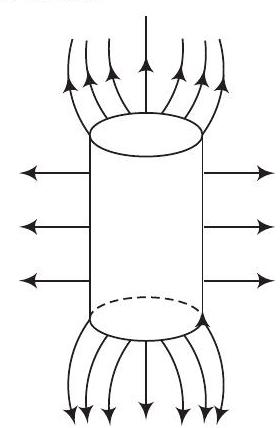
Side view
Top view
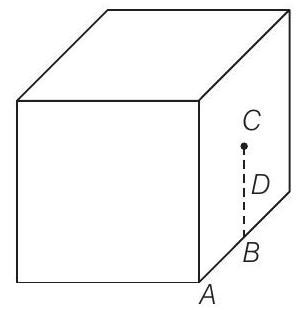
19. What will be the total flux through the faces of the cube as given in the figure with side of length $a$ if a charge $q$ is placed at?
(a) $A$ a corner of the cube
(b) $B$ mid-point of an edge of the cube
(c) $C$ centre of a face of the cube
(d) $D$ mid-point of $B$ and $C$
$K$ Thinking Process
Imagine logically about a symmetric figure in such a way that placed charge arrives at the centre of imaginated figure. Thus,
applying Gauss’ theorem to find flux linked with imaginary figure. Thereafter find flux linked with the given figure.
Show Answer
Answer
(a) There are eight corners in a cube so, total charge for the cube is $\frac{q}{8}$.
Thus, electric flux at $A=\frac{q}{8 \varepsilon_{0}}$.
(b) When the charge $q$ is place at $B$, middle point of an edge of the cube, it is being shared equally by 4 cubes. Therefore, total flux through the faces of the given cube $=q / 4 \varepsilon_{0}$.
(c) When the charge $q$ is placed at $C$, the centre of a face of the cube, it is being shared equally by 2 cubes. Therefore, total flux through the faces of the given cube $=q / 2 \varepsilon_{0}$.
(d) Similarly, when charge $q$ is placed at $Q$, the mid-point of $B$ and $C$, it is being shared equally by 2 cubes. Therefore, total flux through the faces of the given cube $=q / 2 \varepsilon_{0}$.
Short Answer Type Questions
20. A paisa coin is made up of Al-Mg alloy and weight $0.75 \mathrm{~g}$. It has a square shape and its diagonal measures $17 \mathrm{~mm}$. It is electrically neutral and contains equal amounts of positive and negative charges.
Show Answer
Thinking Process
Treating the paisa coins made up of only Al, find the magnitude of equal number of positive and negative charges. What conclusion do you draw from this magnitude?
Answer
Here, given quantities are
Mass of a paisa coin $=0.75 \mathrm{~g}$
Atomic mass of aluminium $=26.9815 \mathrm{~g}$
Avogadro’s number $=6.023 \times 10^{23}$
$\therefore \quad$ Number of aluminium atoms in one paisa coin,
$$ N=\frac{6.023 \times 10^{23}}{26.9815} \times 0.75=1.6742 \times 10^{22} $$
As charge number of $\mathrm{Al}$ is 13 , each atom of $\mathrm{Al}$ contains 13 protons and 13 electrons.
$\therefore \quad$ Magnitude of positive and negative charges in one paisa coin $=\mathrm{N}$ ze
$$ \begin{aligned} & =1.6742 \times 10^{22} \times 13 \times 1.60 \times 10^{-19} \mathrm{C} \ & =3.48 \times 10^{4} \mathrm{C}=34.8 \mathrm{kC} \end{aligned} $$
This is a very large amount of charge. Thus, we can conclude that ordinary neutral matter contains enormous amount of $\pm$ charges.
21. Consider a coin of Question 20. It is electrically neutral and contains equal amounts of positive and negative charge of magnitude $34.8 \mathrm{kC}$. Suppose that these equal charges were concentrated in two point charges separated by
(i) $1 \mathrm{~cm} \sim \frac{1}{2} \times$ diagonal of the one paisa coin
(ii) $100 \mathrm{~m}$ ( length of a long building)
(iii) $10^{6} \mathrm{~m}$ (radius of the earth).
Find the force on each such point charge in each of the three cases. What do you conclude from these results?
Show Answer
Thinking Process
Force on a point charge $=\frac{|q|^{2}}{4 \pi \varepsilon_{0} r^{2}}$. Here, $q=$ magnitude of one charge, $r=$ distance between two charges.
Answer
Here,
$$ \begin{aligned} q= \pm 34.8 \mathrm{RC}= \pm 3.48 \times 10^{4} \mathrm{C} \newline\ r_{1}=1 \mathrm{~cm}=10^{-2} \mathrm{~m}, r_{2}=100 \mathrm{~m}, r_{3}=10^{6} \mathrm{~m} \newline \quad \quad \quad \quad \quad \quad \text { and } \frac{1}{4 \pi \varepsilon_{0}}=9 \times 10^{9} \newline \ F_{1}=\frac{|q|^{2}}{4 \pi \varepsilon_{0} r_{1}^{2}}=\frac{9 \times 10^{9}\left(3.48 \times 10^{4}\right)^{2}}{\left(10^{-2}\right)^{2}}=1.09 \times 10^{23} \mathrm{~N} \newline \ F_{2}=\frac{|q|^{2}}{4 \pi \varepsilon_{0} r_{2}^{2}}=\frac{9 \times 10^{9}\left(3.48 \times 10^{4}\right)^{2}}{(100)^{2}}=1.09 \times 10^{15} \mathrm{~N} \newline \ F_{3}=\frac{|q|^{2}}{4 \pi \varepsilon_{0} r_{3}^{2}}=\frac{9 \times 10^{9}\left(3.48 \times 10^{4}\right)^{2}}{\left(10^{6}\right)^{2}}=1.09 \times 10^{7} \mathrm{~N} \end{aligned} \newline $$
Conclusion from this result We observe that when $\pm$ charges in ordinary neutral matter are separated as point charges, they exert an enormous force. Hence, it is very difficult to disturb electrical neutrality of matter.
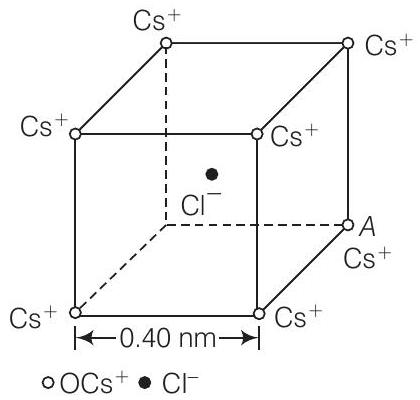
22. Figure represents a crystal unit of cesium chloride, $\mathrm{CsCl}$. The cesium atoms, represented by open circles are situated at the corners of a cube of side $0.40 \mathrm{~nm}$, whereas a $\mathrm{Cl}$ atom is situated at the centre of the cube. The Cs atoms are deficient in one electron while the $\mathrm{Cl}$ atom carries an excess electron.
(i) What is the net electric field on the $\mathrm{Cl}$ atom due to eight $\mathrm{Cs}$ atoms?
(ii) Suppose that the $\mathrm{Cs}$ atom at the corner $A$ is missing. What is the net force now on the $\mathrm{Cl}$ atom due to seven remaining $\mathrm{Cs}^{2}$ atoms?
Show Answer
Thinking Process
(i) Net force on a charge due to two equal and opposite charges will be zero. Also electric field on a charge is given by $E=\frac{F}{q}$ where $E=$ electric field, $F=$ force on charge q due to electric field, $q=$ magnitude of charge $q$
(ii) If a Cs atom is removed from the corner A then a singly charged negative Cs ion at A will appear.
Answer
(i) From the given figure, we can analyse that the chlorine atom is at the centre of the cube i.e., at equal distance from all the eight corners of cube where cesium atoms are placed. Thus, due to symmetry the forces due to all Cs tons, on $\mathrm{Cl}$ atom will cancel out.
Hence,
$$ E=\frac{F}{q} \text { where } F=0 $$
$$ \therefore \quad E=0 $$
(ii) Thus, net force on $\mathrm{Cl}$ atom at $A$ would be,
$$ F=\frac{e^{2}}{4 \pi \varepsilon_{0} r^{2}} $$
where, $\quad r=$ distance between $\mathrm{Cl}$ ion and $\mathrm{Cs}$ ion.
Applying Pythagorous theorem, we get
Now,
$$ \begin{aligned} r & =\sqrt{(0.20)^{2}+(0.20)^{2}+(0.20)^{2}} \times 10^{-9} \mathrm{~m} \newline \ & =0.346 \times 10^{-9} \mathrm{~m} \newline \ F & =\frac{q^{2}}{4 \pi \varepsilon_{0} r^{2}}=\frac{e^{2}}{4 \pi \varepsilon_{0} r_{2}} \newline \ & =\frac{9 \times 10^{9}\left(1.6 \times 10^{-19}\right)^{2}}{\left(0.346 \times 10^{-9}\right)^{2}}=1.92 \times 10^{-9} \mathrm{~N} \newline \end{aligned} $$
23. Two charges $q$ and $-3 q$ are placed fixed on $x$-axis separated by distance $d$. Where should a third charge $2 q$ be placed such that it will not experience any force?
Show Answer
Thinking Process
The force on any charge will be zero only if all forces are balanced i.e., force of attraction is balanced by force of repulsion.
Answer
Here, let us keep the charge $2 q$ at a distance $r$ from $A$.
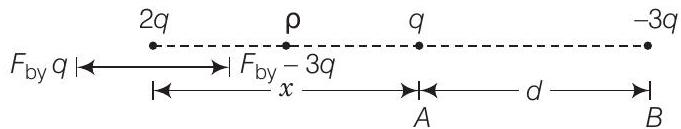
Thus, charge $2 q$ will not experience any force.
When, force of repulsion on it due to $q$ is balanced by force of attraction on it due to $-3 q$, at $B$, where $A B=d$.
Thus, force of attraction by $-3 q=$ Force of repulsion by $q$
$$ \begin{array}{rlrl} \Rightarrow & & \frac{2 q \times q}{4 \pi \varepsilon_{0} x^{2}} & =\frac{2 q \times 3 q}{4 \pi \varepsilon_{0}(x+d)^{2}} \ \Rightarrow & & (x+d)^{2} & =3 x^{2} \ \Rightarrow & x^{2}+d^{2}+2 x d & =3 x^{2} \ \Rightarrow & & =2 x^{2}-d^{2} \end{array} $$
$$ \begin{aligned} \therefore \quad 2 x^{2}-2 d x-d^{2} & =0 \ x & =\frac{d}{2} \pm \frac{\sqrt{3} d}{2} \end{aligned} $$
(Negative sign be between $q$ and $-3 q$ and hence is unadaptable.)
$$ \begin{aligned} x & =-\frac{d}{2}+\frac{\sqrt{3} d}{2} \ & =\frac{d}{2}(1+\sqrt{3}) \text { to the left of } q . \end{aligned} $$
24. Figure shows the electric field lines around three point charges $A, B$ and $C$
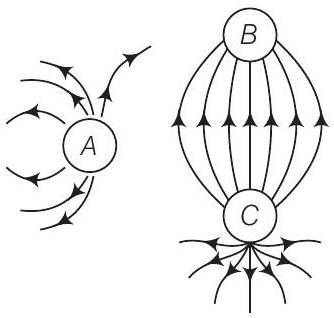
(i) Which charges are positive?
(ii) Which charge has the largest magnitude? Why?
(iii) In which region or regions of the picture could the electric field be zero? Justify your answer. $\newline$
(a) Near $A$ $\newline$
(b) Near $B$ $\newline$
(c) Near C $\newline$
(d) Nowhere $\newline$
Show Answer
Thinking Process
(i) Electric lines of forces always starts from a positive charge and ends at a negative charge. In case of a single charge, electric lines of force start from positive charge ends at infinity.
(ii) The magnitude of a charge depends on the number of lines of force enamating from a charge i.e., higher the number of lines of forces, higher the magnitude of charge and vice-versa.
Answer
(i) Here, in the figure, the electric lines of force emanate from $A$ and $C$. Therefore, charges $A$ and $C$ must be positive.
(ii) The number of electric lines of forces enamating is maximum for charge $C$ here, so $C$ must have the largest magnitude.
(iii) Point between two like charges where electrostatic force is zero is called netural point. So, the neutral point lies between $A$ and $C$ only.
Now the position of neutral point depends on the strength of the forces of charges. Here, more number of electric lines of forces shows higher strength of charge $C$ than $A$. So, neutral point lies near $A$.
25. Five charges, $q$ each are placed at the corners of a regular pentagon of side.
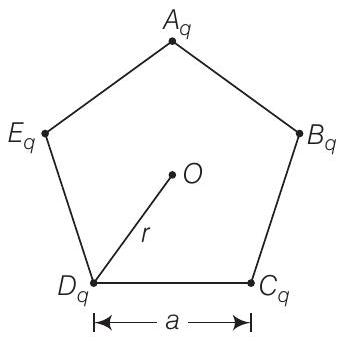
(a) (i) What will be the electric field at 0 , the centre of the pentagon?
(ii) What will be the electric field at 0 if the charge from one of the corners (say $A$ ) is removed?
(iii) What will be the electric field at 0 if the charge $q$ at $A$ is replaced by $-q$ ?
(b) How would your answer to (a) be affected if pentagon is replaced by $n$-sided regular polygon with charge $q$ at each of its corners?
Show Answer
Thinking Process
Due to symmetry forces by all the charges are cancelled out.
Answer
(a) (i) The point $O$ is equidistant from all the charges at the end point of pentagon. Thus, due to symmetry, the forces due to all the charges are cancelled out. As a result electric field at $O$ is zero.
(ii) When charge $q$ is removed a negative charge will develop at $A$ giving electric field $E=\frac{q \times 1}{4 \pi \varepsilon_{0} r^{2}}$ along $O A$.
(iii) If charge $q$ at $A$ is replaced by $-q$, then two negative charges $-2 q$ will develop there. Thus, the value of electric field $E=\frac{2 q}{4 \pi \varepsilon_{0} r^{2}}$ along $O A$.
(b) When pentagon is replaced by $n$ sided regular polygon with charge $q$ at each of its corners, the electric field at $O$ would continue to be zero as symmetricity of the charges is due to the regularity of the polygon. It doesn’t depend on the number of sides or the number of charges.
Long Answer Type Questions
26. In 1959 Lyttleton and Bondi suggested that the expansion of the universe could be explained if matter carried a net charge. Suppose that the universe is made up of hydrogen atoms with a number density $N$, which is maintained a constant. Let the charge on the proton be $e_{p}=-(1+y) e$ where $e$ is the electronic charge.
(a) Find the critical value of $y$ such that expansion may start.
(b) Show that the velocity of expansion is proportional to the distance from the centre.
Show Answer
Thinking Process
Expansion of the universe will start if the coulomb repulsion on a hydrogen atom, at $R$, is larger than the gravitational attraction.
Answer
(a) Let us suppose that universe is a perfect sphere of radius $R$ and its constituent hydrogen atoms are distributed uniformly in the sphere.
As hydrogen atom contains one proton and one electron, charge on each hydrogen atom.
$$ e_{H}=e_{P}+e=-(1+Y) e+e=-Y e=(Y e) $$
If $E$ is electric field intensity at distance $R$, on the surface of the sphere, then according to Gauss’ theorem,
$$ \begin{aligned} \text { g. E.ds } & =\frac{q}{\varepsilon_{0}} \text { i.e., } E\left(4 \pi R^{2}\right)=\frac{4}{3} \frac{\pi R^{3} N|Y e|}{\varepsilon_{0}} \newline\ E & =\frac{1}{3} \frac{N|Y e| R}{\varepsilon_{0}} \newline \end{aligned} $$
Now, suppose, mass of each hydrogen atom $\simeq m_{P}=$ Mass of a proton, $G_{R}=$ gravitational field at distance $R$ on the sphere.
Then
$$ -4 \pi R^{2} G_{R}=4 \pi G m_{P} \quad \frac{4}{3} \pi R^{3} N $$
$$ \Rightarrow \quad G_{R}=\frac{-4}{3} \pi G m_{P} N R $$
$\therefore$ Gravitational force on this atom is $F_{G}=m_{P} \times G_{R}=\frac{-4 \pi}{3} G m_{P}^{2} N R$
Coulomb force on hydrogen atom at $R$ is $F_{C}=(Y e) E=\frac{1}{3} \frac{N Y^{2} e^{2} R}{\varepsilon_{0}}$
Now, to start expansion $F_{C}>F_{G}$ and critical value of $Y$ to start expansion would be when
$$ \begin{aligned} F_{C} & =F_{G} \newline \ \Rightarrow \quad \frac{1}{3} \frac{N Y^{2} e^{2} R}{\varepsilon_{0}} & =\frac{4 \pi}{3} G m_{P}^{2} N R \newline \ \Rightarrow \quad Y^{2} & =\left(4 \pi \varepsilon_{0}\right) G \frac{m_{P}{ }^{2}}{e} \newline \ & =\frac{1}{9 \times 10^{9}} \times\left(6.67 \times 10^{-11}\right) \frac{\left(1.66 \times 10^{-27}\right)^{2}}{\left(1.6 \times 10^{-19}\right)^{2}}=79.8 \times 10^{-38} \newline \ \Rightarrow \quad Y & =\sqrt{79.8 \times 10^{-38}}=8.9 \times 10^{-19} \simeq 10^{-18} \end{aligned} $$
Thus, $10^{-18}$ is the required critical value of $Y$ corresponding to which expansion of universe would start. (b) Net force experience by the hydrogen atom is given by
$$ F=F_{C}-F_{G}=\frac{1}{3} \frac{N Y^{2} e^{2} R}{\varepsilon_{0}}-\frac{4 \pi}{3} G m_{P}^{2} N R $$
If acceleration of hydrogen atom is represent by $d^{2} R / d t^{2}$, then
$$ \begin{aligned} m_{p} \frac{d^{2} R}{d t^{2}} & =F=\frac{1}{3} \frac{N Y^{2} e^{2} R}{\varepsilon_{0}}-\frac{4 \pi}{3} G m_{p}^{2} N R \newline \ & =\frac{1}{3} \frac{N Y^{2} e^{2}}{\varepsilon_{0}}-\frac{4 \pi}{3} G m_{p}^{2} N R \newline \ \therefore \quad \frac{d^{2} R}{d t^{2}} & =\frac{1}{m_{p}} \frac{1}{3} \frac{N Y^{2} e^{2}}{\varepsilon_{0}}-\frac{4 \pi}{3} G m_{p}^{2} N R=\alpha^{2} R \end{aligned} $$
where, $\quad \alpha^{2}=\frac{1}{m_{p}} \frac{1}{3} \frac{N Y^{2} e^{2}}{\varepsilon_{0}}-\frac{4 \pi}{3} G m_{p}^{2} N$
The general solution of Eq (iv) is given by $R=A e^{\alpha t}+B e^{-\alpha t}$. We are looking for expansion, here, so $B=0$ and $R=A e^{\alpha t}$.
$\Rightarrow \quad$ Velocity of expansion, $v=\frac{d R}{d t}=A e^{\alpha t}(\alpha)=\alpha A e^{\alpha t}=\alpha R$
Hence, $v \propto R$ i.e., velocity of expansion is proportional to the distance from the centre.
27. Consider a sphere of radius $R$ with charge density distributed as $p(r)=k r$ for $r \leq R=0$ for $r>R$.
(a) Find the electric field as all points $r$.
(b) Suppose the total charge on the sphere is $2 e$ where $e$ is the electron charge. Where can two protons be embedded such that the force on each of them is zero. Assume that the introduction of the proton does not alter the negative charge distribution.
Show Answer
Thinking Process
According to the given charge density distribution of the sphere of radius $R$ i.e., $p(r)=K r$ for $r \leq R=0$ for $r>R$ it is obvious that the electric field is radial.
Answer
(a) Let us consider a sphere $S$ of radius $R$ and two hypothetic sphere of radius $r<R$ and $r>R$.
Now, for point $r<R$, electric field intensity will be given by,
$$\phi E.ds = \frac{1}{e_0} \int pdV $$
$ [for dV , V = \frac{4}{3} \Pi r^3 \Rightarrow dV = 3 $ $\times \frac{4}{3} \Pi r^3dr = 4 \pi r^2] \newline $ $$\phi E.ds = \frac{1}{e_0} 4 \pi K \int _0^r r^3dr $$ $$E = \frac{1}{4E_0}Kr^2$$

$(\because p(r)=K r)$
Here, charge density is positive.
So, direction of $\mathrm{E}$ is radially outwards.
For points $r>R$, electric field intensity will be given by
$$ \begin{array}{rlrl} \text { gE.dS } =\frac{1}{\varepsilon_{0}} \int \rho . d V \newline \ \Rightarrow E\left(4 \pi^{2}\right) =\frac{4 \pi K}{\varepsilon_{0}} \int_{0}^{R} r^{3} d r=\frac{4 \pi K}{\varepsilon_{0}} \frac{R^{4}}{4} \newline \ \Rightarrow E =\frac{K}{4 \varepsilon_{0}} \frac{R^{4}}{r^{2}} \newline \end{array} $$
Charge density is again positive. So, the direction of $\mathrm{E}$ is radially outward.
(b) The two protons must be on the opposite sides of the centre along a diameter following the rule of symmetry. This can be shown by the figure given below. Charge on the sphere,
$$ \begin{aligned} q & =\int_{0}^{R} \rho d V=\int_{0}^{R}(K r) 4 \pi r^{2} d r \newline \ q & =4 \pi K \frac{R^{4}}{4}=2 e \newline \ \therefore \quad K & =\frac{2 e}{\pi R^{4}} \end{aligned} $$

If protons 1 and 2 are embedded at distance $r$ from the centre of the sphere as shown,then attractive force on proton 1 due to charge distribution is
$$ F_{1}=e E=\frac{-e K r^{2}}{4 \varepsilon_{0}} $$
Repulsive force on proton 1 due to proton 2 is
Net force on proton 1 ,
$$ F_{2}=\frac{e^{2}}{4 \pi \varepsilon_{0}(2 r)^{2}} $$
So,
$$ \begin{aligned} & F=F_{1}+F_{2} \newline \ & F=\frac{-e K r^{2}}{4 \varepsilon_{0}}+\frac{e^{2}}{16 \pi \varepsilon_{0} r^{2}} \newline \ & F=\frac{-r^{2}}{4 \varepsilon_{0}} \frac{Z e}{\pi R^{4}}+\frac{e^{2}}{16 \pi \varepsilon_{0} r^{4}} \end{aligned} $$
Thus, net force on proton 1 will be zero, when
$$ \begin{array}{rlrl} & & \frac{e r^{2} 2 e}{4 \varepsilon_{0} \pi R^{4}} & =\frac{e^{2}}{16 \pi \varepsilon_{0} r} \ \Rightarrow & r^{4} & =\frac{R^{4}}{8} \ \Rightarrow & r & =\frac{R}{(8)^{1 / 4}} \end{array} $$
This is the distance of each of the two protons from the centre of the sphere.
28. Two fixed, identical conducting plates $(\alpha$ and $\beta)$, each of surface area $S$ are charged to $-Q$ and $q$, respectively, where $Q>q>0$. A third identical plate $(\gamma)$, free to move is located on the other side of the plate with charge $q$ at a distance $d$ (figure). The third plate is released and collides with the plate $\beta$. Assume the collision is elastic and the time of collision is sufficient to redistribute charge amongst $\beta$ and $\gamma$.
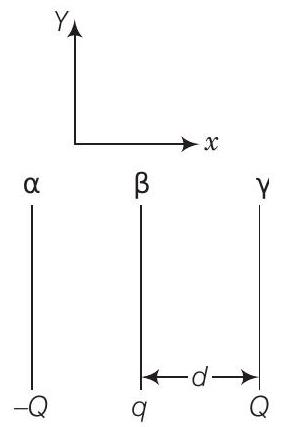
(a) Find the electric field acting on the plate $\gamma$ before collision.
(b) Find the charges on $\beta$ and $\gamma$ after the collision.
(c) Find the velocity of the plate $\gamma$ after the collision and at a distance $d$ from the plate $\beta$.
Show Answer
Answer
(a) Net electric field at plate $\gamma$ before collision is equal to the sum of electric field at plate $\gamma$ due to plate $\alpha$ and $\beta$.
The electric field at plate $\gamma$ due to plate $\alpha$ is $E_{1}=\frac{-Q}{S\left(2 \varepsilon_{0}\right)}$, to the left.
The electric field at plate $\gamma$ due to plate $\beta$ is $E_{2}=\frac{q}{S\left(2 \varepsilon_{0}\right)}$, to the right.
Hence, the net electric field at plate $\gamma$ before collision.
$$ E=E_{1}+E_{2}=\frac{q-Q}{S\left(2 \varepsilon_{0}\right)} \text {, to the left, if } Q>q $$
(b) During collision, plates $\beta$ and $\gamma$ are together. Their potentials become same.
Suppose charge on plate $\beta$ is $q_{1}$ and charge on plate $\gamma$ is $q_{2}$. At any point $O$, in between the two plates, the electric field must be zero.
Electric field at $O$ due to plate $\alpha=\frac{-Q}{S\left(2 \varepsilon_{0}\right)}$, to the left
Electric field at $O$ due to plate $\beta=\frac{q_{1}}{S\left(2 \varepsilon_{0}\right)}$, to the right
Electric field at $O$ due to plate $\gamma=\frac{q_{2}}{S\left(2 \varepsilon_{0}\right)}$, to the left
As the electric field at $O$ is zero, therefore
$$ \begin{aligned} \frac{Q+q_{2}}{S\left(2 \varepsilon_{0}\right)} & =\frac{q_{1}}{S\left(2 \varepsilon_{0}\right)} \ Q+q_{2} & =q_{1} \ Q & =q_{1}-q_{2} \end{aligned} $$
As there is no loss of charge on collision,
$$ Q+q=q_{1}+q_{2} $$
On solving Eqs. (i) and (ii), we get
$$ \begin{aligned} & q_{1}=(Q+q / 2)=\text { charge on plate } \beta \ & q_{2}=(q / 2)=\text { charge on plate } \gamma \end{aligned} $$
(c) After collision, at a distance $d$ from plate $\beta$,
Let the velocity of plate $\gamma$ be $v$. After the collision, electric field at plate $\gamma$ is
$$ E_{2}=\frac{-Q}{2 \varepsilon_{0} S}+\frac{(Q+q / 2)}{2 \varepsilon_{0} S}=\frac{q / 2}{2 \varepsilon_{0} S} \text { to the right. } $$
Just before collision, electric field at plate $\gamma$ is $E_{1}=\frac{Q-q}{2 \varepsilon_{0} S}$.
If $F_{1}$ is force on plate $\gamma$ before collision, then $F_{1}=E_{1} Q=\frac{(Q-q) Q}{2 \varepsilon_{0} S}$
Total work done by the electric field is round trip movement of plate $\gamma$
$$ \begin{aligned} W & =\left(F_{1}+F_{2}\right) d \ & =\frac{\left[(Q-q) Q+(q / 2)^{2}\right] d}{2 \varepsilon_{0} S}=\frac{(Q-q / 2)^{2} d}{2 \varepsilon_{0} S} \end{aligned} $$
If $m$ is mass of plate $\gamma$, the KE gained by plate $\gamma=\frac{1}{2} m v^{2}$
According to work-energy principle, $\frac{1}{2} m v^{2}=W=\frac{(Q-q / 2)^{2} d}{2 \varepsilon_{0} S}$
$$ Y=(Q-q / 2){\frac{d}{m \varepsilon_{0} S}}^{1 / 2} $$
29. There is another useful system of units, besides the SI/MKS. A system, called the CGS (Centimeter-Gram-Second) system. In this system, Coulomb’s law is given by $\mathbf{F}=\frac{Q q}{r^{2}} \hat{\mathbf{r}}$.
where the distance $r$ is measured in $\mathrm{cm}\left(=10^{-2} \mu\right), F$ in dynes $\left(=10^{-5} \mathrm{~N}\right)$ and the charges in electrostatic units (es units), where 1 es unit of charge $=\frac{1}{[3]} \times 10^{-9} \mathrm{C}$. The number [3] actually arises from the speed of light in vacuum which is now taken to be exactly given by $c=2.99792458 \times 10^{8} \mathrm{~m} / \mathrm{s}$. An approximate value of $c$, then is $c=3 \times 10^{8} \mathrm{~m} / \mathrm{s}$.
(i) Show that the Coulomb’s law in CGS units yields 1 esu of charge $=1(\text { dyne })^{1 / 2} \mathrm{~cm}$. Obtain the dimensions of units of charge in terms of mass $M$, length $L$ and time $T$. Show that it is given in terms of fractional powers of $M$ and $L$.
(ii) Write 1 esu of charge $=x C$, where $x$ is a dimensionless number. Show that this gives $\frac{1}{4 \pi \varepsilon_{0}}=\frac{10^{-9}}{x^{2}} \frac{\mathrm{Nm}^{2}}{\mathrm{C}^{2}}$. With $x=\frac{1}{[3]} \times 10^{-9}$, we have $\frac{1}{4 \pi \varepsilon_{0}}=[3]^{2} \times 10^{9} \frac{\mathrm{Nm}^{2}}{\mathrm{C}^{2}}, \frac{1}{4 \pi \varepsilon_{0}}=(2.99792458)^{2} \times 10^{9} \frac{\mathrm{Nm}^{2}}{\mathrm{C}^{2}}$ (exactly).
Show Answer
Answer
(i) From the relation, $F=\frac{Q q}{r^{2}}=1$ dyne $=\frac{[1 \text { esu of charge }]^{2}}{[1 \mathrm{~cm}]^{2}}$
So, 1 esu of charge $=(1 \text { dyne })^{1 / 2} \times 1 \mathrm{~cm}=F^{1 / 2} \cdot L=\left[\mathrm{MLT}^{-2}\right]^{1 / 2} \mathrm{~L}$
$\Rightarrow 1$ esu of charge $=\mathrm{M}^{1 / 2} \mathrm{~L}^{3 / 2} \mathrm{~T}^{-1}$.
Thus, esu of charge is represented in terms of fractional powers $\frac{1}{2}$ of $M$ and $\frac{3}{2}$ of $L$.
(ii) Let 1 esu of charge $=x C$, where $x$ is a dimensionless number. Coulomb force on two charges, each of magnitude 1 esu separated by $1 \mathrm{~cm}$ is dyne $=10^{-5} \mathrm{~N}$. This situation is equivalent to two charges of magnitude $x C$ separated by $10^{-2} \mathrm{~m}$.
$$ \begin{array}{ll} \therefore F=\frac{1}{4 \pi \varepsilon_{0}} \frac{x^{2}}{\left(10^{-2}\right)^{2}}=1 \text { dyne }=10^{-5} \mathrm{~N} \newline \newline \ \therefore \frac{1}{4 \pi \varepsilon_{0}}=\frac{10^{-9}}{x^{2}} \frac{\mathrm{Nm}^{2}}{\mathrm{C}^{2}} \newline \newline \ \text { Taking, } x=\frac{1}{|3| \times 10^{9}}, \newline \newline \ \text { we get, } \frac{1}{4 \pi \varepsilon_{0}}=10^{-9} \times|3|^{2} \times 10^{18} \frac{\mathrm{Nm}^{2}}{\mathrm{C}^{2}}=9 \times 10^{9} \frac{\mathrm{Nm}^{2}}{\mathrm{C}^{2}} \newline \newline \ \text { If }|3| \rightarrow 2.99792458 \text {, we get } \frac{1}{4 \pi \varepsilon_{0}}=8.98755 \times 10^{9} \mathrm{Nm}^{2} \mathrm{C}^{-2} . \end{array} $$
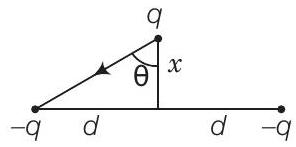
30. Two charges $-q$ each are fixed separated by distance $2 d$. A third charge $q$ of mass $m$ placed at the mid-point is displaced slightly by $x(x<d)$ perpendicular to the line joining the two fixed charged as shown in figure. Show that $q$ will perform simple harmonic oscillation of time period.
$$ T={\frac{8 \pi^{3} \varepsilon_{0} m d^{3}}{q^{2}}}^{1 / 2} $$
Show Answer
Thinking Process
For motion of charge a to be simple harmonic, force on charge q must be proportional to its distance from the centre $\mathrm{O}$ and is directed towards $\mathrm{O}$.
Answer
Let us elaborate the figure first.
Given, two charge $-q$ at $A$ and $B$
$$ A B=A O+O B=2 d $$
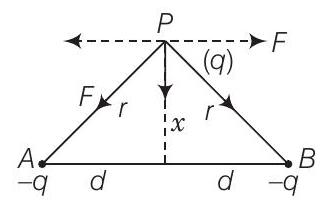
$x=$ small distance perpendicular to $O$.
i.e., $x<d$ mass of charge $q$ is. So, force of attraction at $P$ towards $A$ and $B$ are each $F=\frac{q(q)}{4 \pi \varepsilon_{0} r^{2}}$, where $A P=B P=r$
Horizontal components of these forces $F_{n}$ are cancel out. Vertical components along $P O$ add.
If $\angle A P O=O$, the net force on $q$ along $P O$ is $F^{\prime}=2 F \cos Q$
$$ \begin{aligned} & =\frac{2 q^{2}}{4 \pi \varepsilon_{0} r^{2}} \frac{x}{r} \newline \ & =\frac{2 q^{2} x}{4 \pi \varepsilon_{0}\left(d^{2}+x^{2}\right)^{3 / 2}} \end{aligned} $$
When,
$$ x«d, F^{\prime}=\frac{2 q^{2} x}{4 \pi \varepsilon_{0} d^{3}}=K x $$
where,
$$ K=\frac{2 q^{2}}{4 \pi \varepsilon_{0} d^{3}} $$
$\Rightarrow$
$$ F \propto x $$
i.e., force on charge $q$ is proportional to its displacement from the centre $\mathrm{O}$ and it is directed towards $\mathrm{O}$.
Hence, motion of charge $q$ would be simple harmonic, where
and
$$ \begin{aligned} \omega & =\sqrt{\frac{K}{m}} \newline \ T & =\frac{2 \pi}{\omega}=2 \pi \sqrt{\frac{m}{K}} \newline \ & =2 \pi \sqrt{\frac{m \cdot 4 \pi \varepsilon_{0} d^{3}}{2 q^{2}}}=\frac{8 \pi^{3} \varepsilon_{0} m d^{3}}{q^{2}} \end{aligned} $$
31. Total charge $-Q$ is uniformly spread along length of a ring of radius $R$.A small test charge $+q$ of mass $m$ is kept at the centre of the ring and is given a gentle push along the axis of the ring.
(a) Show that the particle executes a simple harmonic oscillation.
(b) Obtain its time period.
Thinking Process
For simple harmonic oscillation, force on $q$ is proportional to negative of its displacement.
Show Answer
Answer
Let us draw the figure according to question,
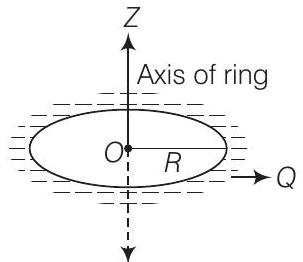
A gentle push on $q$ along the axis of the ring gives rise to the situation shown in the figure below.
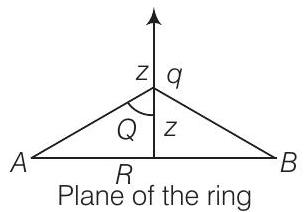
Taking line elements of charge at $A$ and $B$, having unit length, then charge on each elements.
$$ d F=2-\frac{Q}{2 \pi R} q \times \frac{1}{4 \pi \varepsilon_{0}} \frac{1}{r^{2}} \cos \theta $$
Total force on the charge $q$, due to entire ring
$$ \begin{aligned} F & =-\frac{Q q}{\pi R}(\pi R) \cdot \frac{1}{4 \pi \varepsilon_{0}} \frac{1}{r^{2}} \cdot \frac{2}{r} \ F & =-\frac{Q q z}{4 \pi \varepsilon_{0}\left(Z^{2}+R^{2}\right)^{3 / 2}} \end{aligned} $$
Here, $Z<R, \quad F=-\frac{Q q z}{4 \pi \varepsilon_{0} R^{3}}=-K z$
where
$$ \frac{Q q}{4 \pi \varepsilon_{0} R^{3}}=\text { constant } $$
$F \propto-Z$
$\Rightarrow$
Clearly, force on $q$ is proportional to negative of its displacement. Therefore, motion of $q$ is simple harmonic.
$\Rightarrow \quad T=2 \pi \sqrt{\frac{4 \pi \varepsilon_{0} m R^{3}}{Q q}}$
$$ \begin{aligned} \omega=\sqrt{\frac{K}{m}} \text { and } T=\frac{2 \pi}{\omega}=2 \pi \sqrt{\frac{m}{K}} \newline \ T=2 \pi \sqrt{\frac{m 4 \pi \varepsilon_{0} R^{3}}{Q q}} \newline \ T=2 \pi \sqrt{\frac{4 \pi \varepsilon_{0} m R^{3}}{Q q}} \end{aligned} $$










