Equilibrium
Exercise
7.1 A liquid is in equilibrium with its vapour in a sealed container at a fixed temperature. The volume of the container is suddenly increased.
a) What is the initial effect of the change on vapour pressure?
b) How do rates of evaporation and condensation change initially?
c) What happens when equilibrium is restored finally and what will be the final vapour pressure?
Show Answer
Answer
(a) If the volume of the container is suddenly increased, then the vapour pressure would decrease initially. This is because the amount of vapour remains the same, but the volume increases suddenly. As a result, the same amount of vapour is distributed in a larger volume.
(b) Since the temperature is constant, the rate of evaporation also remains constant. When the volume of the container is increased, the density of the vapour phase decreases. As a result, the rate of collisions of the vapour particles also decreases. Hence, the rate of condensation decreases initially.
(c) When equilibrium is restored finally, the rate of evaporation becomes equal to the rate of condensation. In this case, only the volume changes while the temperature remains constant. The vapour pressure depends on temperature and not on volume. Hence, the final vapour pressure will be equal to the original vapour pressure of the system.
7.2 What is $K_{\mathrm{c}}$ for the following equilibrium when the equilibrium concentration of each substance is: $\left[\mathrm{SO_2}\right]=0.60 \mathrm{M},\left[\mathrm{O_2}\right]=0.82 \mathrm{M}$ and $\left[\mathrm{SO_3}\right]=1.90 \mathrm{M}$ ?
$$ 2 \mathrm{SO_2}(\mathrm{~g})+\mathrm{O_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{SO_3}(\mathrm{~g}) $$
Show Answer
Answer
The equilibrium constant $(K_c)$ for the give reaction is:
$ \begin{aligned} K_c & =\frac{[SO_3]^{2}}{[SO_2]^{2}[O_2]} \\ & =\frac{(1.90)^{2} M^{2}}{(0.60)^{2}(0.821) M^{3}} \\ & =12.239 M^{-1} \text{ (approximately) } \end{aligned} $
Hence, $K_c$ for the equilibrium is $12.239 M^{-1}$.
7.3 At a certain temperature and total pressure of $10^{5} \mathrm{~Pa}$, iodine vapour contains $40 %$ by volume of I atoms
$$ \mathrm{I_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{I}(\mathrm{g}) $$
Calculate $K_{p}$ for the equilibrium.
Show Answer
Answer
Partial pressure of I atoms,
$p_1=\frac{40}{100} \times p _{\text{total }}$
$=\frac{40}{100} \times 10^{5}$
$=4 \times 10^{4} Pa$
Partial pressure of $I_2$ molecules,
$ \begin{aligned} & p _{I_2}=\frac{60}{100} \times p _{\text{total }} \\ & =\frac{60}{100} \times 10^{5} \\ & =6 \times 10^{4} Pa \end{aligned} $
Now, for the given reaction,
$ \begin{aligned} K_p & =\frac{(p I)^{2}}{p _{I_2}} \\ & =\frac{(4 \times 10^{4})^{2} Pa^{2}}{6 \times 10^{4} Pa} \\ & =2.67 \times 10^{4} Pa \end{aligned} $
7.4 Write the expression for the equilibrium constant, $K_{c}$ for each of the following reactions:
(i) $2 \mathrm{NOCl}(\mathrm{g}) \rightleftharpoons 2 \mathrm{NO}(\mathrm{g})+\mathrm{Cl_2}(\mathrm{~g})$
(ii) $2 \mathrm{Cu}\left(\mathrm{NO_3}\right)_{2}(\mathrm{~s}) \rightleftharpoons 2 \mathrm{CuO}(\mathrm{s})+4 \mathrm{NO_2}(\mathrm{~g})+\mathrm{O_2}(\mathrm{~g})$
(iii) $\mathrm{CH_3} \mathrm{COOC_2} \mathrm{H_5}(\mathrm{aq})+\mathrm{H_2} \mathrm{O}(\mathrm{l}) \rightleftharpoons \mathrm{CH_3} \mathrm{COOH}(\mathrm{aq})+\mathrm{C_2} \mathrm{H_5} \mathrm{OH}(\mathrm{aq})$
(iv) $\mathrm{Fe}^{3+}(\mathrm{aq})+3 \mathrm{OH}^{-}(\mathrm{aq}) \rightleftharpoons \mathrm{Fe}(\mathrm{OH})_{3}(\mathrm{~s})$
(v) $\mathrm{I_2}(\mathrm{~s})+5 \mathrm{~F_2} \rightleftharpoons 2 \mathrm{IF_5}$
Show Answer
Answer
(i) $\quad K_c=\frac{[NO _{(g)}]^{2}[Cl _{2(g)}]}{[NOCl _{(g)}]^{2}}$
(ii) $\quad K_c=\frac{[CuO _{(s)}]^{2}[NO _{2(g)}]^{4}[O _{2(g)}]}{[Cu(NO_3) _{2(s)}]^{2}}$
$ =[NO _{2(g)}]^{4}[O _{2(g)}] $
(iii) $K_c=\frac{[CH_3 COOH _{(a q)}][C_2 H_5 OH _{(a q)}]}{[CH_3 COOC_2 H _{5(a q)}][H_2 O _{(l)}]}=\frac{[CH_3 COOH _{(a q)}][C_2 H_5 OH _{(a q)}]}{[CH_3 COOC_2 H _{5(a q)}]}$
(iv) $K_c=\frac{[Fe(OH) _{3(s)}]}{[Fe _{(a q)}^{3+}][OH _{(a q)}^{-}]^{3}}$
$ =\frac{1}{[Fe _{(a q)}^{3+}][OH _{(a q)}^{-}]^{3}} $
(v) $\quad K_c=\frac{[IF_5]^{2}}{[I _{2(s)}][F_2]^{5}}$
$=\frac{[IF_5]^{2}}{[F_2]^{5}}$
7.5 Find out the value of $K_{c}$ for each of the following equilibria from the value of $K_{p}$ :
(i) $2 \mathrm{NOCl}(\mathrm{g}) \rightleftharpoons 2 \mathrm{NO}(\mathrm{g})+\mathrm{Cl_2}(\mathrm{~g}) ; K_{p}=1.8 \times 10^{-2}$ at $500 \mathrm{~K}$
(ii) $\mathrm{CaCO_3}$ (s) $\rightleftharpoons \mathrm{CaO}$ (s) $+\mathrm{CO_2}(\mathrm{~g}) ; K_{p}=167$ at $1073 \mathrm{~K}$
Show Answer
Answer
The relation between $K_p$ and $K_c$ is given as:
$K_p=K_c(RT)^{\Delta n}$
(a) Here,
$\Delta n=3-2=1$
$R=0.0831$ barLmol $^{-1} K^{-1}$
$T=500 K$
$K_p=1.8 \times 10^{-2}$
Now,
$K_p=K_c(R T)^{\Delta n}$
$\Rightarrow 1.8 \times 10^{-2}=K_c(0.0831 \times 500)^{1}$
$\Rightarrow K_c=\frac{1.8 \times 10^{-2}}{0.0831 \times 500}$
$ =4.33 \times 10^{-4} \text{ (approximately) } $
(b) Here,
$\Delta n=2$ - $1=1$
$R=0.0831$ barLmol $^{-1} K^{-1}$
$T=1073 K$
$K_p=167$
Now,
$K_p=K_c(R T)^{\Delta n}$
$\Rightarrow 167=K_c(0.0831 \times 1073)^{\Delta n}$
$\Rightarrow K_c=\frac{167}{0.0831 \times 1073}$
$=1.87$ (approximately)
7.6 For the following equilibrium, $K_{c}=6.3 \times 10^{14}$ at $1000 \mathrm{~K}$ $\mathrm{NO}(\mathrm{g})+\mathrm{O_3}(\mathrm{~g}) \rightleftharpoons \mathrm{NO_2}(\mathrm{~g})+\mathrm{O_2}(\mathrm{~g})$
Both the forward and reverse reactions in the equilibrium are elementary bimolecular reactions. What is $K_{c}$, for the reverse reaction?
Show Answer
Answer
It is given that $K_C$ for the forward reaction is $6.3 \times 10^{14}$.
Then, $K_C$ for the reverse reaction will be,
$ \begin{aligned} K_C^{\prime} & =\frac{1}{K_C} \\ & =\frac{1}{6.3 \times 10^{14}} \\ & =1.59 \times 10^{-15} \end{aligned} $
7.7 Explain why pure liquids and solids can be ignored while writing the equilibrium constant expression?
Show Answer
Answer
For a pure substance (both solids and liquids),
$ \begin{aligned} {[\text{ Pure substance }] } & =\frac{\text{ Number of moles }}{\text{ Volume }} \\ & =\frac{\text{ Mass } / \text{ molecular mass }}{\text{ Volume }} \\ & =\frac{\text{ Mass }}{\text{ Volume } \times \text{ Molecular mass }} \\ & =\frac{\text{ Density }}{\text{ Molecular mass }} \end{aligned} $
Now, the molecular mass and density (at a particular temperature) of a pure substance is always fixed and is accounted for in the equilibrium constant. Therefore, the values of pure substances are not mentioned in the equilibrium constant expression.
7.8 Reaction between $\mathrm{N_2}$ and $\mathrm{O_2-}$ takes place as follows:
$$ 2 \mathrm{~N_2}(\mathrm{~g})+\mathrm{O_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{~N_2} \mathrm{O}(\mathrm{g}) $$
If a mixture of $0.482 \mathrm{~mol} \mathrm{~N_2}$ and $0.933 \mathrm{~mol}$ of $\mathrm{O_2}$ is placed in a $10 \mathrm{~L}$ reaction vessel and allowed to form $\mathrm{N_2} \mathrm{O}$ at a temperature for which $K_{c}=2.0 \times 10^{-37}$, determine the composition of equilibrium mixture.
Show Answer
Answer
Let the concentration of $N_2 O$ at equilibrium be $x$.
The given reaction is:
| $2 N _{2(g)}+$ | $O _{2(g)}$ | $\longleftrightarrow$ | |
|---|---|---|---|
| Initial conc. $\quad 0.482 mol$ | $0.933 mol$ | 0 | |
| At equilibrium $(0.482-x) mol$ | $(1.933-x) mol$ | $x$ mol |
Therefore, at equilibrium, in the $10 L$ vessel:
$[N_2]=\frac{0.482-x}{10},[O_2]=\frac{0.933-x / 2}{10},[N_2 O]=\frac{x}{10}$
The value of equilibrium constant i.e., $K_C=2.0 \times 10^{9 a+37}$ is very small. Therefore, the amount of $N_2$ and $O_2$ reacted is also very small. Thus, $x$ can be neglected from the expressions of molar concentrations of $N_2$ and $O_2$.
Then,
$[N_2]=\frac{0.482}{10}=0.0482 mol L^{-1}$ and $[O_2]=\frac{0.933}{10}=0.0933 mol L^{-1}$
Now,
$ \begin{aligned} & K_C=\frac{[N_2 O _{(g)}]^{2}}{[N _{2(g)}]^{2}[O _{2(g)}]} \\ & \Rightarrow 2.0 \times 10^{-37}=\frac{(\frac{x}{10})^{2}}{(0.0482)^{2}(0.0933)} \\ & \Rightarrow \frac{x^{2}}{100}=2.0 \times 10^{-37} \times(0.0482)^{2} \times(0.0933) \\ & \Rightarrow x^{2}=43.35 \times 10^{-40} \\ & \Rightarrow x=6.6 \times 10^{-20} \\ & {[N_2 O]=\frac{x}{10}=\frac{6.6 \times 10^{-20}}{10}} \\ & =6.6 \times 10^{-21} \end{aligned} $
7.9 Nitric oxide reacts with $\mathrm{Br_2}$ and gives nitrosyl bromide as per reaction given below:
$$ 2 \mathrm{NO}(\mathrm{g})+\mathrm{Br_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{NOBr}(\mathrm{g}) $$
When $0.087 \mathrm{~mol}$ of $\mathrm{NO}$ and $0.0437 \mathrm{~mol}$ of $\mathrm{Br_2}$ are mixed in a closed container at constant temperature, $0.0518 \mathrm{~mol}$ of $\mathrm{NOBr}$ is obtained at equilibrium. Calculate equilibrium amount of $\mathrm{NO}$ and $\mathrm{Br_2}$.
Show Answer
Answer
The given reaction is:
$ 2 NO _{(g)}+Br _{2(g)} \longleftrightarrow 2 NOBr _{(g)} $
$2 mol \quad 1 mol \quad 2 mol$
Now, 2 mol of $NOBr$ are formed from $2 mol$ of $NO$. Therefore, $0.0518 mol$ of $NOBr$ are formed from $0.0518 mol$ of $NO$.
Again, 2 mol of $NOBr$ are formed from $1 mol$ of $Br$.
Therefore, $0.0518 mol$ of $NOBr$ are formed from $\frac{0.0518}{2} mol$ of $Br$, or $0.0259 mol$ of NO.
The amount of $NO$ and $Br$ present initially is as follows:
$[NO]=0.087 mol[Br_2]=0.0437 mol$
Therefore, the amount of NO present at equilibrium is:
$[N O]=0.087$ - 0.0518
$=0.0352 mol$
And, the amount of $Br$ present at equilibrium is:
$[Br_2]=0.0437$ - 0.0259
$=0.0178 mol$
7.10 At $450 \mathrm{~K}, K_{p}=2.0 \times 10^{10} /$ bar for the given reaction at equilibrium. What is $K_{c}$ at this temperature ? $$ 2 \mathrm{SO_2}(\mathrm{~g})+\mathrm{O_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{SO_3}(\mathrm{~g}) $$
Show Answer
Answer
For the given reaction,
$\Delta n=2$ - 3 = - 1
$T=450 K$
$R=0.0831$ bar $L$ bar $K^{- 1 }$
$K_p=2.0 \times 10^{10} bar^{-1}$
We know that,
$ \begin{aligned} & K_P=K_C(R T) \Delta n \\ & \begin{aligned} & \Rightarrow 2.0 \times 10^{10} bar^{-1}=K_C(0.0831 L bar K^{-1} mol^{-1} \times 450 K)^{-1} \\ & \Rightarrow K_C=\frac{2.0 \times 10^{10} bar^{-1}}{(0.0831 L bar K^{-1} mol^{-1} \times 450 K)^{-1}} \\ &=(2.0 \times 10^{10} bar^{-1})(0.0831 Lbar K^{-1} mol^{-1} \times 450 K) \\ &=74.79 \times 10^{10} L mol^{-1} \\ &=7.48 \times 10^{11} L mol^{-1} \\ &=7.48 \times 10^{11} M^{-1} \end{aligned} \end{aligned} $
7.11 A sample of $\mathrm{HI}(\mathrm{g})$ is placed in flask at a pressure of $0.2 \mathrm{~atm}$. At equilibrium the partial pressure of $\mathrm{HI}(\mathrm{g})$ is $0.04 \mathrm{~atm}$. What is $K_{p}$ for the given equilibrium ?
$$ 2 \mathrm{HI}(\mathrm{g}) \rightleftharpoons \mathrm{H_2}(\mathrm{~g})+\mathrm{I_2}(\mathrm{~g}) $$
Show Answer
Answer
The initial concentration of $HI$ is $0.2 atm$. At equilibrium, it has a partial pressure of $0.04 atm$. Therefore, a decrease in the pressure of $HI$ is $0.2 \hat{a} E^{\prime \prime} 0.04=0.16$. The given reaction is:
$ 2 HI _{(g)} \longleftrightarrow H _{2(g)}+I _{2(g)} $
Initial conc. $\quad 0.2 atm$
$0 \quad 0$
At equilibrium $\quad 0.04 atm$
$ \begin{aligned} & \frac{0.16}{2} \quad \frac{2.15}{2} \\ & =0.08 atm=0.08 atm \end{aligned} $
Therefore,
$ \begin{aligned} K_p & =\frac{p _{H_2} \times p _{I_2}}{p_HI^{2}} \\ & =\frac{0.08 \times 0.08}{(0.04)^{2}} \\ & =\frac{0.0064}{0.0016} \\ & =4.0 \end{aligned} $
Hence, the value of $K_p$ for the given equilibrium is 4.0 .
7.12 A mixture of $1.57 \mathrm{~mol}$ of $\mathrm{N_2}, 1.92 \mathrm{~mol}$ of $\mathrm{H_2}$ and $8.13 \mathrm{~mol}$ of $\mathrm{NH_3}$ is introduced into a $20 \mathrm{~L}$ reaction vessel at $500 \mathrm{~K}$. At this temperature, the equilibrium constant, $K_{\mathrm{c}}$ for the reaction $\mathrm{N_2}(\mathrm{~g})+3 \mathrm{H_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{NH_3}(\mathrm{~g})$ is $1.7 \times 10^{2}$. Is the reaction mixture at equilibrium? If not, what is the direction of the net reaction?
Show Answer
Answer
The given reaction is:
$N _{2(g)}+3 H _{2(g)} \longleftrightarrow 2 NH _{3(g)}$
The given concentration of various species is
$[N_2]=\frac{1.57}{20} mol L^{-1} \quad[H_2]=\frac{1.92}{20} mol L^{-1}$
$[NH_3]=\frac{8.13}{20} mol L^{-1}$
Now, reaction quotient $Q_c$ is:
$ \begin{aligned} Q_C & =\frac{[NH_3]^{2}}{[N_2][H_2]^{3}} \\ & =\frac{(\frac{(8.13)}{20})^{2}}{(\frac{1.57}{20})(\frac{1.92}{20})^{3}} \\ & =2.4 \times 10^{3} \end{aligned} $
Since $Q_C \neq K_C$, the reaction mixture is not at equilibrium.
Again, $Q_C>K_C$. Hence, the reaction will proceed in the reverse direction.
7.13 The equilibrium constant expression for a gas reaction is,
$$ K_{c}=\frac{\left[\mathrm{NH_3}\right]^{4}\left[\mathrm{O_2}\right]^{5}}{[\mathrm{NO}]^{4}\left[\mathrm{H_2} \mathrm{O}\right]^{6}} $$
Write the balanced chemical equation corresponding to this expression.
Show Answer
Answer
The balanced chemical equation corresponding to the given expression can be written as:
$4 NO _{(g)}+6 H_2 O _{(g)} \longleftrightarrow 4 NH _{3(g)}+5 O _{2(g)}$
7.14 One mole of $\mathrm{H_2} \mathrm{O}$ and one mole of $\mathrm{CO}$ are taken in $10 \mathrm{~L}$ vessel and heated to $725 \mathrm{~K}$. At equilibrium $40 %$ of water (by mass) reacts with $\mathrm{CO}$ according to the equation,
$$ \mathrm{H_2} \mathrm{O}(\mathrm{g})+\mathrm{CO}(\mathrm{g}) \rightleftharpoons \mathrm{H_2}(\mathrm{~g})+\mathrm{CO_2}(\mathrm{~g}) $$
Calculate the equilibrium constant for the reaction.
Show Answer
Answer
The given reaction is:
$ \begin{aligned} & H_2 O _{(g)}+CO _{(g)} \longleftrightarrow H _{2(g)}+CO _{2(g)} \\ & \begin{matrix} \text{ Initial conc. } & \frac{1}{10} M & \frac{1}{10} M & 0 & 0 \end{matrix} \\ & \text{ At equilibrium } \quad \frac{1-0.4}{10} M \quad \frac{1-0.4}{10} M \quad \frac{0.4}{10} M \quad \frac{0.4}{10} M \\ & =0.06 M \quad=0.06 M \quad=0.04 M \quad=0.04 M \end{aligned} $
Therefore, the equilibrium constant for the reaction,
$ \begin{aligned} K_C & =\frac{[H_2][CO_2]}{[H_2 O][CO]} \\ & =\frac{0.04 \times 0.04}{0.06 \times 0.06} \\ & =0.444 \text{ (approximately) } \end{aligned} $
7.15 At $700 \mathrm{~K}$, equilibrium constant for the reaction: $$ \mathrm{H_2}(\mathrm{~g})+\mathrm{I_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{HI}(\mathrm{g}) $$ is 54.8. If $0.5 \mathrm{~mol} \mathrm{~L}^{-1}$ of $\mathrm{HI}(\mathrm{g})$ is present at equilibrium at $700 \mathrm{~K}$, what are the concentration of $\mathrm{H_2}(\mathrm{~g})$ and $\mathrm{I_2}(\mathrm{~g})$ assuming that we initially started with $\mathrm{HI}(\mathrm{g})$ and allowed it to reach equilibrium at $700 \mathrm{~K}$ ?
Show Answer
Answer
It is given that equilibrium constant $K_C$ for the reaction
$H _{2(g)}+I _{2(g)} \longleftrightarrow 2 HI _{(g)}$ is 54.8.
Therefore, at equilibrium, the equilibrium constant $K_C^{\prime}$ for the reaction
$2 HI _{(g)} \longleftrightarrow H _{2(g)}+I _{2(g)}$ will be $\frac{1}{54.8}$.
$[HI]=0.5 molL^{-1}$
Let the concentrations of hydrogen and iodine at equilibrium be $x molL^{-1}$
$[H_2]=[I_2]=x mol L^{-1}$
Therefore, $\frac{[H_2][I_2]}{[HI]^{2}}=K_C^{\prime}$
$\Rightarrow \frac{x \times x}{(0.5)^{2}}=\frac{1}{54.8}$
$\Rightarrow x^{2}=\frac{0.25}{54.8}$
$\Rightarrow x=0.06754$
$x=0.068 molL^{-1}$ (approximately)
Hence, at equilibrium, $[H_2]=[I_2]=0.068 mol L^{-1}$.
7.16 What is the equilibrium concentration of each of the substances in the equilibrium when the initial concentration of $\mathrm{ICl}$ was $0.78 \mathrm{M}$ ?
$2 \mathrm{ICl}(\mathrm{g}) \rightleftharpoons \mathrm{I_2}(\mathrm{~g})+\mathrm{Cl_2}(\mathrm{~g}) ; \quad K_{c}=0.14$
Show Answer
Answer
The given reaction is:
| $2 ICl(g)$ | $Cl_{(g)}$ | $I_2(g)+$ | |
|---|---|---|---|
| Initial conc. | $0.78 M$ | 0 | 0 |
| At equilibrium | $(0.78-2 x) M$ | $x M$ | $x M$ |
Now, we can write, $\frac{[I_2][Cl_2]}{[ICl]^{2}}=K_C$
$\Rightarrow \frac{x \times x}{(0.78-2 x)^{2}}=0.14$
$\Rightarrow \frac{x^{2}}{(0.78-2 x)^{2}}=0.14$
$\Rightarrow \frac{x}{0.78-2 x}=0.374$
$\Rightarrow x=0.292-0.748 x$
$\Rightarrow 1.748 x=0.292$
$\Rightarrow x=0.167$
Hence, at equilibrium,
$[ICl]=[I 2]=$
7.17 $K_{p}=0.04 \mathrm{~atm}$ at $899 \mathrm{~K}$ for the equilibrium shown below. What is the equilibrium concentration of $\mathrm{C_2} \mathrm{H_6}$ when it is placed in a flask at $4.0 \mathrm{~atm}$ pressure and allowed to come to equilibrium?
$$ \mathrm{C_2} \mathrm{H_6}(\mathrm{~g}) \rightleftharpoons \mathrm{C_2} \mathrm{H_4}(\mathrm{~g})+\mathrm{H_2}(\mathrm{~g}) $$
Show Answer
Answer
Let $p$ be the pressure exerted by ethene and hydrogen gas (each) at equilibrium.
Now, according to the reaction,
Initial conc.
$ C_2 H _{6(g)} \longleftrightarrow C_2 H _{4(g)}+H _{2(g)} $
At equilibrium 4.0atm 0 0
We can write,
$ \begin{aligned} & \frac{p _{C_2 H_4} \times p _{H_2}}{p _{C_2 H_6}}=K_p \\ & \Rightarrow \frac{p \times p}{4.0-p}=0.04 \\ & \Rightarrow p^{2}+0.16-0.04 p \\ & \Rightarrow p^{2}+0.04 p-0.16=0 \\ & \text{ Now }, p=\frac{-0.04 \pm \sqrt{(0.04)^{2}-4 \times 1 \times(-0.16)}}{2 \times 1} \\ & \quad=\frac{-0.04 \pm 0.80}{2} \\ & \quad=\frac{0.76}{2} \quad(\text{ Taking positive value}) \\ & \quad=0.38 \end{aligned} $
Hence, at equilibrium,
$ \begin{aligned} {[C_2 H_6]-4-p } & =4-0.38 \\ & =3.62 atm \end{aligned} $
7.18 Ethyl acetate is formed by the reaction between ethanol and acetic acid and the equilibrium is represented as:
$$ \mathrm{CH_3} \mathrm{COOH}(1)+\mathrm{C_2} \mathrm{H_5} \mathrm{OH}(1) \rightleftharpoons \mathrm{CH_3} \mathrm{COOC_2} \mathrm{H_5}(1)+\mathrm{H_2} \mathrm{O}(1) $$
(i) Write the concentration ratio (reaction quotient), $Q_{\mathrm{c}}$, for this reaction (note: water is not in excess and is not a solvent in this reaction)
(ii) At $293 \mathrm{~K}$, if one starts with $1.00 \mathrm{~mol}$ of acetic acid and $0.18 \mathrm{~mol}$ of ethanol, there is $0.171 \mathrm{~mol}$ of ethyl acetate in the final equilibrium mixture. Calculate the equilibrium constant.
(iii) Starting with $0.5 \mathrm{~mol}$ of ethanol and $1.0 \mathrm{~mol}$ of acetic acid and maintaining it at $293 \mathrm{~K}, 0.214 \mathrm{~mol}$ of ethyl acetate is found after sometime. Has equilibrium been reached?
Show Answer
Answer
(i) Reaction quotient,
$ Q_C=\frac{[CH_3 COOC_2 H_5][H_2 O]}{[CH_3 COOH][C_2 H_5 OH]} $
(ii) Let the volume of the reaction mixture be $V$. Also, here we will consider that water is a solvent and is present in excess.
The given reaction is:
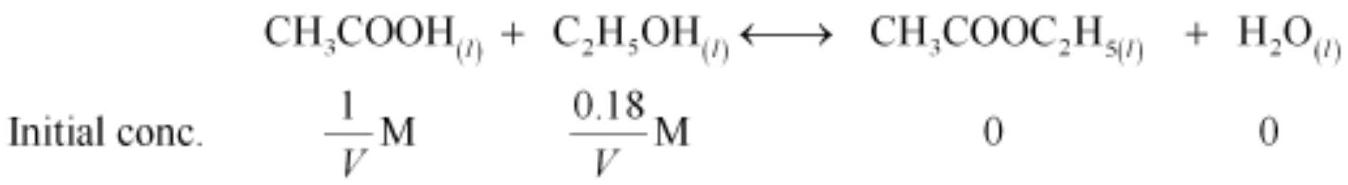
$ \begin{matrix} \text{ At equilibrium } & \frac{1-0.171}{V} & \frac{0.18-0.171}{V} & \frac{0.171}{V} M & \frac{0.171}{V} M \\ = & \frac{0.829}{V} M & =\frac{0.009}{V} M & \end{matrix} $
Therefore, equilibrium constant for the given reaction is:
$ \begin{aligned} K_C & =\frac{[CH_3 COOC_2 H_5][H_2 O]}{[CH_3 COOH][C_2 H_5 OH]} \\ & =\frac{\frac{0.171}{V} \times \frac{0.171}{V}}{\frac{0.829}{V} \times \frac{0.009}{V}}=3.919 \\ = & 3.92(\text{ approximately }) \end{aligned} $
(iii) Let the volume of the reaction mixture be $V$.
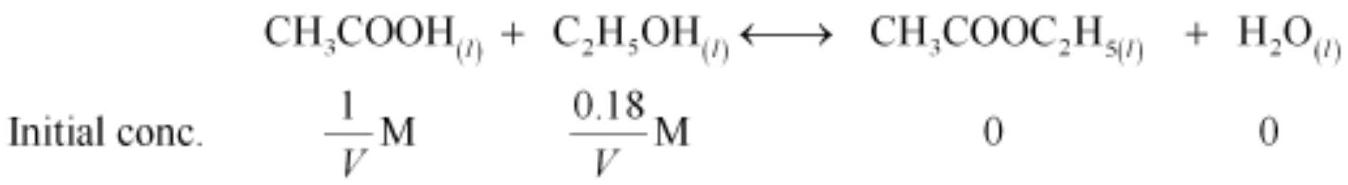 $
\begin{matrix}
\text{ After some time } & \frac{10-0.214}{V} & \frac{0.5-0.214}{V} & \frac{0.214}{V} M & \frac{0.214}{V} M \\\
& =\frac{0.786}{V} M & =\frac{0.286}{V} M &
\end{matrix}
$
$
\begin{matrix}
\text{ After some time } & \frac{10-0.214}{V} & \frac{0.5-0.214}{V} & \frac{0.214}{V} M & \frac{0.214}{V} M \\\
& =\frac{0.786}{V} M & =\frac{0.286}{V} M &
\end{matrix}
$
Therefore, the reaction quotient is,
$ \begin{aligned} Q_C & =\frac{[CH_3 COOC_2 H_5][H_2 O]}{[CH_3 COOH][C_2 H_5 OH]} \\ & =\frac{\frac{0.214}{V} \times \frac{0.214}{V}}{\frac{0.786}{V} \times \frac{0.286}{V}} \\ & =0.2037 \\ & =0204 \text{ (approximately) } \end{aligned} $
Since $Q_C<K_C$, equilibrium has not been reached.
7.19 A sample of pure $\mathrm{PCl_5}$ was introduced into an evacuated vessel at 473 K. After equilibrium was attained, concentration of $\mathrm{PCl_5}$ was found to be $0.5 \times 10^{-1} \mathrm{~mol} \mathrm{~L}^{-1}$. If value of $K_{\mathrm{c}}$ is $8.3 \times 10^{-3}$, what are the concentrations of $\mathrm{PCl_3}$ and $\mathrm{Cl_2}$ at equilibrium?
$$ \mathrm{PCl_5}(\mathrm{~g}) \rightleftharpoons \mathrm{PCl_3}(\mathrm{~g})+\mathrm{Cl_2}(\mathrm{~g}) $$
Show Answer
Answer
Let the concentrations of both $PCl_3$ and $Cl_2$ at equilibrium be $x$ molL ${ }^{\hat{e} e^{-1}}$. The given reaction is:
concentration of $PCL_5$ was found to be $ 0.5 \times 10^{-1} molL^{-1} $. If value of $K_c$ is $8.3 \times 10 ^{-3}$, what are the
At equilibrium $0.5 \times 10^{-1} mol L^{-1} \quad x mol L^{-1} \quad x mol L^{-1}$
It is given that the value of equilibrium constant, $K_C$ is $8.3 \times 10^{-3}$.
Now we can write the expression for equilibrium as:
$ \begin{aligned} & \frac{[PCl_2][Cl_2]}{[PCl_5]}=K_C \\ & \Rightarrow \frac{x \times x}{0.5 \times 10^{-1}}=8.3 \times 10^{-3} \\ & \Rightarrow x^{2}=4.15 \times 10^{-4} \\ & \Rightarrow x=2.04 \times 10^{-2} \\ & \quad=0.0204 \\ & \quad=0.02 \text{ (approximately) } \end{aligned} $
Therefore, at equilibrium,
$[PCl_3]=[Cl_2]=0.02 mol L{ }^{-1}$.
7.20 One of the reaction that takes place in producing steel from iron ore is the reduction of iron(II) oxide by carbon monoxide to give iron metal and $\mathrm{CO_2}$.
$\mathrm{FeO}(\mathrm{s})+\mathrm{CO}(\mathrm{g}) \rightleftharpoons \mathrm{Fe}(\mathrm{s})+\mathrm{CO_2}(\mathrm{~g}) ; K_{p}=0.265 \mathrm{~atm}$ at $1050 \mathrm{~K}$
What are the equilibrium partial pressures of $\mathrm{CO}$ and $\mathrm{CO_2}$ at $1050 \mathrm{~K}$ if the initial partial pressures are: $p_{\mathrm{CO}}=1.4 \mathrm{~atm}$ and $p_{\mathrm{CO_2}}=0.80 \mathrm{~atm}$ ?
Show Answer
Answer
For the given reaction,
$ FeO_{(g)} + CO_{(g)} \longleftrightarrow Fe_{(s)} + CO_{2(g)} $
Initialy, $\quad 1.4 atm \quad 0.80 atm$
$ \begin{aligned} Q_p & =\frac{p _{CO_2}}{p _{CO}} \\ & =\frac{0.80}{1.4} \\ & =0.571 \end{aligned} $
It is given that $K_p=0.265$.
Since $Q_P>K_P$, the reaction will proceed in the backward direction.
Therefore, we can say that the pressure of $CO$ will increase while the pressure of $CO_2$ will decrease.
Now, let the increase in pressure of $CO=$ decrease in pressure of $CO_2$ be $p$.
Then, we can write,
$ \begin{aligned} & K_P=\frac{p _{CO_2}}{p _{CO}} \\ & \Rightarrow 0.265=\frac{0.80-p}{1.4+p} \\ & \Rightarrow 0.371+0.265 p=0.80-p \\ & \Rightarrow 1.265 p=0.429 \\ & \Rightarrow p=0.339 atm \end{aligned} $
Therefore, equilibrium partial of $CO_2, p _{CO_2}=0.80-0.339=0.461 atm$.
And, equilibrium partial pressure of $CO, p _{CO}=1.4+0.339=1.739 atm$.
7.21 Equilibrium constant, $K_{c}$ for the reaction $$ \mathrm{N_2}(\mathrm{~g})+3 \mathrm{H_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{NH_3}(\mathrm{~g}) \text { at } 500 \mathrm{~K} \text { is } 0.061 $$
At a particular time, the analysis shows that composition of the reaction mixture is $3.0 \mathrm{~mol} \mathrm{~L}^{-1} \mathrm{~N_2}, 2.0 \mathrm{~mol} \mathrm{~L}^{-1} \mathrm{H_2}$ and $0.5 \mathrm{~mol} \mathrm{~L}^{-1} \mathrm{NH_3}$. Is the reaction at equilibrium? If not in which direction does the reaction tend to proceed to reach equilibrium?
Show Answer
Answer
The given reaction is:
$ N _{2(g)}+3 H _{2(g)} \longleftrightarrow \quad 2 NH _{3(g)} $
At a particular time: $3.0 mol L^{-1} \quad 2.0 mol L^{-1} \quad 0.5 mol L^{-1}$
Now, we know that,
$ \begin{aligned} Q_C & =\frac{[NH_3]^{2}}{[N_2][H_2]^{3}} \\ & =\frac{(0.5)^{2}}{(3.0)(2.0)^{3}} \\ & =0.0104 \end{aligned} $
It is given that $K_C=0.061$.
Since $Q_C \neq K_C$, the reaction is not at equilibrium.
Since $Q_C<K_C$, the reaction will proceed in the forward direction to reach equilibrium.
7.22 Bromine monochloride, $\mathrm{BrCl}$ decomposes into bromine and chlorine and reaches the equilibrium:
$$ 2 \mathrm{BrCl}(\mathrm{g}) \rightleftharpoons \mathrm{Br_2}(\mathrm{~g})+\mathrm{Cl_2}(\mathrm{~g}) $$
for which $K_{\mathrm{c}}=32$ at $500 \mathrm{~K}$. If initially pure $\mathrm{BrCl}$ is present at a concentration of $3.3 \times 10^{-3} \mathrm{~mol} \mathrm{~L}^{-1}$, what is its molar concentration in the mixture at equilibrium?
Show Answer
Answer
Let the amount of bromine and chlorine formed at equilibrium be $x$. The given reaction is:
$2BrCl_{(g)} \longleftrightarrow Br_{2(g)} + Cl_{2(g)} $
Initail conc. $ 3.3 \times 10^{-3} m$
At equilibrium $ 3.3 \times 10 ^{-2} -2a$
Now, we can write,
$ \begin{aligned} & \frac{[Br_2][Cl_2]}{[BrCl^{2}]}=K_C \\ \Rightarrow & \frac{x \times x}{(3.3 \times 10^{-3}-2 x)^{2}}=32 \\ \Rightarrow & \frac{x}{3.3 \times 10^{-3}-2 x}=5.66 \\ \Rightarrow & x=18.678 \times 10^{-3}-11.32 x \\ \Rightarrow & 12.32 x=18.678 \times 10^{-3} \\ \Rightarrow & x=1.5 \times 10^{-3} \end{aligned} $
Therefore, at equilibrium,
$ \begin{aligned} {[BrCl] } & =3.3 \times 10^{-3}-(2 \times 1.5 \times 10^{-3}) \\ & =3.3 \times 10^{-3}-3.0 \times 10^{-3} \\ & =0.3 \times 10^{-3} \\ & =3.0 \times 10^{-4} molL^{-1} \end{aligned} $
7.23 At $1127 \mathrm{~K}$ and $1 \mathrm{~atm}$ pressure, a gaseous mixture of $\mathrm{CO}$ and $\mathrm{CO_2}$ in equilibrium with soild carbon has $90.55 %$ CO by mass
$$ \mathrm{C}(\mathrm{s})+\mathrm{CO_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{CO}(\mathrm{g}) $$
Calculate $K_{\mathrm{c}}$ for this reaction at the above temperature.
Show Answer
Answer
Let the total mass of the gaseous mixture be $100 g$.
Mass of $CO=90.55 g$
And, mass of $CO_2=(100 a \in " 90.55)=9.45 g$
Now, number of moles of $CO$,
$ n _{CO}=\frac{90.55}{28}=3.234 mol $
Number of moles of $CO_2$
$ n _{CO_2}=\frac{9.45}{44}=0.215 mol $
Partial pressure of $CO$,
$ \begin{aligned} p _{CO} & =\frac{n _{CO}}{n _{CO}+n _{CO_2}} \times p _{\text{total }} \\ & =\frac{3.234}{3.234+0.215} \times 1 \\ & =0.938 atm \end{aligned} $
Partial pressure of $CO_2$,
$ \begin{aligned} p _{CO_2} & =\frac{n _{CO_2}}{n _{CO}+n _{CO_2}} \times p _{\text{total }} \\ & =\frac{0.215}{3.234+0.215} \times 1 \\ & =0.062 atm \end{aligned} $
Therefore, $K_P=\frac{[CO]^{2}}{[CO_2]}$
$ \begin{aligned} & =\frac{(0.938)^{2}}{0.062} \\ & =14.19 \end{aligned} $
For the given reaction,
$\Delta n=2 -1=1$
We know that,
$K_p=K_C(R T)^{\Delta r}$
$\Rightarrow 14.19=K_C(0.082 \times 1127)^{\prime}$
$\Rightarrow K_C=\frac{14.19}{0.082 \times 1127}$
$=0.154$ (approximately)
7.24 Calculate a) $\Delta G^{\ominus}$ and b) the equilibrium constant for the formation of $\mathrm{NO_2}$ from $\mathrm{NO}$ and $\mathrm{O_2}$ at $298 \mathrm{~K}$
$$ \mathrm{NO}(\mathrm{g})+1 / 2 \mathrm{O_2}(\mathrm{~g}) \rightleftharpoons \mathrm{NO_2}(\mathrm{~g}) $$
where
$\Delta_{\mathrm{f}} G^{\ominus}\left(\mathrm{NO_2}\right)=52.0 \mathrm{~kJ} / \mathrm{mol}$
$\Delta_{\mathrm{f}} G^{\ominus}(\mathrm{NO})=87.0 \mathrm{~kJ} / \mathrm{mol}$
$\Delta_{\mathrm{f}} G^{\ominus}\left(\mathrm{O_2}\right)=0 \mathrm{~kJ} / \mathrm{mol}$
Show Answer
Answer
(a) For the given reaction,
$\Delta G^{\circ}=\Delta G^{\circ}$ ( Products) â $\epsilon^{\prime \prime} \Delta G^{\circ}$ ( Reactants)
$\Delta G^{\circ}=52.0$ - ${87.0+0}$
= - $35.0 kJ mol|^{\hat{\epsilon} e^{\prime \prime}}$
(b) We know that,
$\Delta G^{\circ}=RT \log K_c$
$\Delta G^{\circ}=2.303 RT \log K_c$
$K_c=\frac{-35.0 \times 10^{-3}}{-2.303 \times 8.314 \times 298}$
$=6.134$
$\therefore K_c=$ antilog (6.134)
$=1.36 \times 10^{6}$
Hence, the equilibrium constant for the given reaction $K_c$ is $1.36 \times 10^{6}$
7.25 Does the number of moles of reaction products increase, decrease or remain same when each of the following equilibria is subjected to a decrease in pressure by increasing the volume?
(a) $\mathrm{PCl} 5(\mathrm{~g}) \rightleftharpoons \mathrm{PCl} 3(\mathrm{~g})+\mathrm{Cl} 2(\mathrm{~g})$
(b) $\mathrm{CaO}(\mathrm{s})+\mathrm{CO} 2(\mathrm{~g}) \rightleftharpoons \mathrm{CaCO} 3(\mathrm{~s})$
(c) $3 \mathrm{Fe}(\mathrm{s})+4 \mathrm{H_2} \mathrm{O}(\mathrm{g}) \rightleftharpoons \mathrm{Fe_3} \mathrm{O_4}(\mathrm{~s})+4 \mathrm{H_2}(\mathrm{~g})$
Show Answer
Answer
(a) The number of moles of reaction products will increase. According to Le Chatelier’s principle, if pressure is decreased, then the equilibrium shifts in the direction in which the number of moles of gases is more. In the given reaction, the number of moles of gaseous products is more than that of gaseous reactants. Thus, the reaction will proceed in the forward direction. As a result, the number of moles of reaction products will increase.
(b) The number of moles of reaction products will decrease.
(c) The number of moles of reaction products remains the same.
7.26 Which of the following reactions will get affected by increasing the pressure? Also, mention whether change will cause the reaction to go into forward or backward direction.
(i) $\mathrm{COCl_2}(\mathrm{~g}) \rightleftharpoons \mathrm{CO}(\mathrm{g})+\mathrm{Cl_2}(\mathrm{~g})$
(ii) $\mathrm{CH} 4(\mathrm{~g})+2 \mathrm{~S} 2(\mathrm{~g}) \rightleftharpoons \mathrm{CS} 2(\mathrm{~g})+2 \mathrm{H} 2 \mathrm{~S}(\mathrm{~g})$
(iii) $\mathrm{CO} 2(\mathrm{~g})+\mathrm{C}(\mathrm{s}) \rightleftharpoons 2 \mathrm{CO}(\mathrm{g})$
(iv) $2 \mathrm{H} 2(\mathrm{~g})+\mathrm{CO}(\mathrm{g}) \rightleftharpoons \mathrm{CH} 3 \mathrm{OH}(\mathrm{g})$
(v) $\mathrm{CaCO} 3(\mathrm{~s}) \rightleftharpoons \mathrm{CaO}(\mathrm{s})+\mathrm{CO} 2(\mathrm{~g})$
(vi) $4 \mathrm{NH_3}(\mathrm{~g})+5 \mathrm{O_2}(\mathrm{~g}) \rightleftharpoons 4 \mathrm{NO}(\mathrm{g})+6 \mathrm{H_2} \mathrm{O}(\mathrm{g})$
Show Answer
Answer
The reactions given in (i), (iii), (iv), (v), and (vi) will get affected by increasing the pressure.
The reaction given in (iv) will proceed in the forward direction because the number of moles of gaseous reactants is more than that of gaseous products.
The reactions given in (i), (iii), (v), and (vi) will shift in the backward direction because the number of moles of gaseous reactants is less than that of gaseous products.
7.27 The equilibrium constant for the following reaction is $1.6 \times 10^{5}$ at $1024 \mathrm{~K}$ $\mathrm{H_2}(\mathrm{~g})+\mathrm{Br_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{HBr}(\mathrm{g})$
Find the equilibrium pressure of all gases if 10.0 bar of $\mathrm{HBr}$ is introduced into a sealed container at $1024 \mathrm{~K}$.
Show Answer
Answer
Given,
$K _{\text{P for the reaction i.e., }} H _{2(g)}+Br _{2(g)} \longleftrightarrow 2 HBr _{(g)}$ is $1.6 \times 10^{5}$.
Therefore, for the reaction $2 HBr _{(g)} \longleftrightarrow H _{2(g)}+Br _{2(g)}$, the equilibrium constant will be,
$ \begin{aligned} K_P^{\prime} & =\frac{1}{K_P} \\ & =\frac{1}{1.6 \times 10^{5}} \\ & =6.25 \times 10^{-6} \end{aligned} $
Now, let $p$ be the pressure of both $H_2$ and $Br_2$ at equilibrium.
| $2 HBr _{(g)}$ | $\longleftrightarrow$ | $H _{2(g)}+Br _{2(g)}$ | ||
|---|---|---|---|---|
| Initial conc. | 10 | 0 | 0 | |
| At equilibrium | $10-2 p$ | $p$ | $p$ |
Now, we can write,
$ \begin{aligned} & \frac{p _{HBr} \times p_2}{p_HBr^{2}}=K_P^{\prime} \\ & \frac{p \times p}{(10-2 p)^{2}}=6.25 \times 10^{-6} \end{aligned} $
$\frac{p}{10-2 p}=2.5 \times 10^{-3}$
$p=2.5 \times 10^{-2}-(5.0 \times 10^{-3}) p$
$p+(5.0 \times 10^{-3}) p=2.5 \times 10^{-2}$
$(1005 \times 10^{-3}) p=2.5 \times 10^{-2}$
$p=2.49 \times 10^{-2}$ bar $=2.5 \times 10^{-2}$ bar (approximately)
Therefore, at equilibrium,
$[H_2]=[Br_2]=2.49 \times 10^{-2}$ bar
$[HBr]=10-2 \times(2.49 \times 10^{-2})$ bar
$=9.95 bar=10 bar$ (approximately)
7.28 Dihydrogen gas is obtained from natural gas by partial oxidation with steam as per following endothermic reaction:
$\mathrm{CH_4}(\mathrm{~g})+\mathrm{H_2} \mathrm{O}(\mathrm{g}) \rightleftharpoons \mathrm{CO}(\mathrm{g})+3 \mathrm{H_2}(\mathrm{~g})$
(a) Write as expression for $\mathrm{Kp}$ for the above reaction.
(b) How will the values of $\mathrm{Kp}$ and composition of equilibrium mixture be affected by
(i) increasing the pressure
(ii) increasing the temperature
(iii) using a catalyst?
Show Answer
Answer
(a) For the given reaction, $K_P = \frac{p_{CO} \times p^3_{H_2}}{p_{CH_4} \times p_{H_2 O}}$
(b) (i) According to Le Chatelier’s principle, the equilibrium will shift in the backward direction.
(ii) According to Le Chatelier’s principle, as the reaction is endothermic, the equilibrium will shift in the forward direction.
(iii) The equilibrium of the reaction is not affected by the presence of a catalyst. A catalyst only increases the rate of a reaction. Thus, equilibrium will be attained quickly.
7.29 Describe the effect of:
a) addition of $\mathrm{H} 2$
b) addition of $\mathrm{CH} 3 \mathrm{OH}$
c) removal of $\mathrm{CO}$
d) removal of $\mathrm{CH_3} \mathrm{OH}$
on the equilibrium of the reaction:
$$ 2 \mathrm{H_2}(\mathrm{~g})+\mathrm{CO}(\mathrm{g}) \rightleftharpoons \mathrm{CH_3} \mathrm{OH}(\mathrm{g}) $$
Show Answer
Answer
(a) According to Le Chatelier’s principle, on addition of $H_2$, the equilibrium of the given reaction will shift in the forward direction.
(b) On addition of $CH_3 OH$, the equilibrium will shift in the backward direction.
(c) On removing $CO$, the equilibrium will shift in the backward direction.
(d) On removing $CH_3 OH$, the equilibrium will shift in the forward direction.
7.30 At $473 \mathrm{~K}$, equilibrium constant $K_{c}$ for decomposition of phosphorus pentachloride, $\mathrm{PCl_5}$ is $8.3 \times 10^{-3}$. If decomposition is depicted as, $\mathrm{PCl_5}(\mathrm{~g}) \rightleftharpoons \mathrm{PCl_3}(\mathrm{~g})+\mathrm{Cl_2}(\mathrm{~g}) \quad \Delta_{\mathrm{r}} H^{\ominus}=124.0 \mathrm{~kJ} \mathrm{~mol}{ }^{-1}$
a) write an expression for $\mathrm{Kc}$ for the reaction.
b) what is the value of $\mathrm{Kc}$ for the reverse reaction at the same temperature?
c) what would be the effect on $K_{c}$ if (i) more $\mathrm{PCl_5}$ is added (ii) pressure is increased (iii) the temperature is increased?
Show Answer
Answer (a)
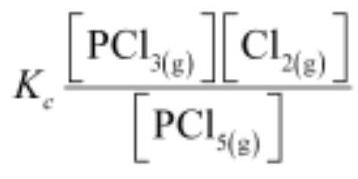
(b) Value of $K_c$ for the reverse reaction at the same temperature is:
$ \begin{aligned} K_c^{\prime} & =\frac{1}{K_c} \\ & =\frac{1}{8.3 \times 10^{-3}}=1.2048 \times 10^{2} \\ & =120-48 \end{aligned} $
(c) (i) $K_c$ would remain the same because in this case, the temperature remains the same.
(ii) $K_c$ is constant at constant temperature. Thus, in this case, $K_c$ would not change.
(iii) In an endothermic reaction, the value of $K_c$ increases with an increase in temperature. Since the given reaction in an endothermic reaction, the value of $K_c$ will increase if the temperature is increased.
7.31 Dihydrogen gas used in Haber’s process is produced by reacting methane from natural gas with high temperature steam. The first stage of two stage reaction involves the formation of $\mathrm{CO}$ and $\mathrm{H_2}$. In second stage, $\mathrm{CO}$ formed in first stage is reacted with more steam in water gas shift reaction,
$\mathrm{CO}(\mathrm{g})+\mathrm{H_2} \mathrm{O}(\mathrm{g}) \rightleftharpoons \mathrm{CO_2}(\mathrm{~g})+\mathrm{H_2}(\mathrm{~g})$
If a reaction vessel at $400^{\circ} \mathrm{C}$ is charged with an equimolar mixture of $\mathrm{CO}$ and steam such that $p_{\text {co }}=p_{\mathrm{H_2} \mathrm{O}}=4.0$ bar, what will be the partial pressure of $\mathrm{H_2}$ at equilibrium? $K_{p}=10.1$ at $400^{\circ} \mathrm{C}$
Show Answer
Answer
Let the partial pressure of both carbon dioxide and hydrogen gas be $p$. The given reaction is:
$ CO _{(g)}+H_2 O _{(g)} \longleftrightarrow CO _{2(g)}+H _{2(g)} $
$\begin{matrix} \text{ Initial conc. } & 4.0 bar & 4.0 bar & 0\end{matrix} $
$\begin{matrix} \text{ At equilibrium } & 4.0-p & 4.0-p & p\end{matrix} $
It is given that $K_p=10.1$.
Now,
$ \begin{aligned} & \frac{p _{CO_2} \times p _{H_2}}{p _{COH} \times p_2}=K_P \\ & \Rightarrow \frac{p \times p}{(4.0-p)(4.0-p)}=10.1 \\ & \Rightarrow \frac{p}{4.0-p}=3.178 \\ & \Rightarrow p=12.712-3.178 p \\ & \Rightarrow 4.178 p=12.712 \\ & \Rightarrow p=3.04 \end{aligned} $
Hence, at equilibrium, the partial pressure of $H_2$ will be 3.04 bar.
7.32 Predict which of the following reaction will have appreciable concentration of reactants and products:
a) $\mathrm{Cl} 2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{Cl}(\mathrm{g}) \mathrm{Kc}=5 \times 10-39$
b) $\mathrm{Cl} 2(\mathrm{~g})+2 \mathrm{NO}(\mathrm{g}) \rightleftharpoons 2 \mathrm{NOCl}(\mathrm{g}) \quad \mathrm{Kc}=3.7 \times 108$
c) $\mathrm{Cl} 2(\mathrm{~g})+2 \mathrm{NO_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{NO_2} \mathrm{Cl}(\mathrm{g}) K_{c}=1.8$
Show Answer
Answer
If the value of $K_c$ lies between $10^{-3}$ and $10^{3}$, a reaction has appreciable concentration of reactants and products. Thus, the reaction given in (c) will have appreciable concentration of reactants and products.
7.33 The value of $K_{c}$ for the reaction $3 \mathrm{O_2}(\mathrm{~g}) \rightleftharpoons 2 \mathrm{O_3}(\mathrm{~g})$ is $2.0 \times 10^{-50}$ at $25^{\circ} \mathrm{C}$. If the equilibrium concentration of $\mathrm{O_2}$ in air at $25^{\circ} \mathrm{C}$ is $1.6 \times 10^{-2}$, what is the concentration of $\mathrm{O_3}$ ?
Show Answer
Answer
The given reaction is: $3 O _{2(g)} \longleftrightarrow 2 O _{3(g)}$
Then, $K_C=\frac{[O _{3(g)}]^{2}}{[O _{2(g)}]^{3}}$
It is given that $K_C=2.0 \times 10^{-50}$ and $[O _{2(g)}]=1.6 \times 10^{-2}$.
Then, we have,
$2.0 \times 10^{-50}=\frac{[O _{3(g)}]^{2}}{[1.6 \times 10^{-2}]^{3}}$
$\Rightarrow[O _{3(g)}]^{2}=2.0 \times 10^{-50} \times(1.6 \times 10^{-2})^{3}$
$\Rightarrow[O _{3(g)}]^{2}=8.192 \times 10^{-56}$
$\Rightarrow[O _{3(g)}]=2.86 \times 10^{-28} M$
Hence, the concentration of $O_3$ is $2.86 \times 10^{-28} M$.
7.34 The reaction, $\mathrm{CO}(\mathrm{g})+3 \mathrm{H_2}(\mathrm{~g}) \rightleftharpoons \mathrm{CH_4}(\mathrm{~g})+\mathrm{H_2} \mathrm{O}(\mathrm{g})$ is at equilibrium at $1300 \mathrm{~K}$ in a $1 \mathrm{~L}$ flask. It also contain $0.30 \mathrm{~mol}$ of $\mathrm{CO}, 0.10 \mathrm{~mol}$ of $\mathrm{H_2}$ and $0.02 \mathrm{~mol}$ of $\mathrm{H_2} \mathrm{O}$ and an unknown amount of $\mathrm{CH_4}$ in the flask. Determine the concentration of $\mathrm{CH_4}$ in the mixture. The equilibrium constant, $K_{c}$ for the reaction at the given temperature is 3.90 .
Show Answer
Answer
Let the concentration of methane at equilibrium be $x$.
$ CO _{(g)}+3 H _{2(g)} \longleftrightarrow CH _{4(g)}+H_2 O _{(g)} $
At equilibrium $\frac{0.3}{1}=0.3 M \quad \frac{0.1}{1}=0.1 M \quad x \quad \frac{0.02}{1}=0.02 M$
It is given that $K_c=3.90$.
Therefore,
$ \begin{gathered} \frac{[CH _{4(g)}][H_2 O _{(g)}]}{[CO _{(g)}][H _{2(g)}]^{3}}=K_c \\ \Rightarrow \frac{x \times 0.02}{0.3 \times(0.1)^{3}}=3.90 \\ \Rightarrow x=\frac{3.90 \times 0.3 \times(0.1)^{3}}{0.02} \\ \quad=\frac{0.00117}{0.02} \\ =0.0585 M \\ =5.85 \times 10^{-2} M \end{gathered} $
Hence, the concentration of $CH_4$ at equilibrium is $5.85 \times 10^{-2} M$.
7.35 What is meant by the conjugate acid-base pair? Find the conjugate acid/base for the following species:
$\mathrm{HNO_2}, \mathrm{CN}^{-}, \mathrm{HClO_4} \mathrm{~F}^{-}, \mathrm{OH}^{-}, \mathrm{CO_3}^{2-}$, and $\mathrm{S}^{2-}$
Show Answer
Answer
A conjugate acid-base pair is a pair that differs only by one proton.
The conjugate acid-base for the given species is mentioned in the table below.
7.36 Which of the followings are Lewis acids? $\mathrm{H_2} \mathrm{O}, \mathrm{BF_3}, \mathrm{H}^{+}$, and $\mathrm{NH_4}^{+}$
Show Answer
Answer
Lewis acids are those acids which can accept a pair of electrons. For example, $BF_3, H^{+}$, and $NH_4^{+}$are Lewis acids.
7.37 What will be the conjugate bases for the Brönsted acids: $\mathrm{HF}, \mathrm{H_2} \mathrm{SO_4}$ and $\mathrm{HCO_3}^{-}$?
Show Answer
Answer
The table below lists the conjugate bases for the given Bronsted acids.
Bronsted acid
$HF$
$H_2 SO_4$
$HCO_3^{-}$
Conjugate base
$Fi$
$HSO_4^{-}$
$CO_3^{2-}$
7.38 Write the conjugate acids for the following Brönsted bases: $\mathrm{NH_2}^{-}, \mathrm{NH_3}$ and $\mathrm{HCOO}^{-}$.
Show Answer
Answer
The table below lists the conjugate acids for the given Bronsted bases.
Bronsted base
$NH_2^{-}$
$NH_3$
$HCOO^{-}$
Conjugate acid
$NH_3$
$NH_4^{+}$
$HCOOH$
7.39 The species: $\mathrm{H_2} \mathrm{O}, \mathrm{HCO_3}^{-}, \mathrm{HSO_4}^{-}$and $\mathrm{NH_3}$ can act both as Brönsted acids and bases. For each case give the corresponding conjugate acid and base.
Show Answer
Answer
The table below lists the conjugate acids and conjugate bases for the given species.
Species
$H_2 O$
$HCO_3^{-}$
$HSO_4^{-}$
$NH_3$
Conjugate acid
$H_3 O^{+}$
$H_2 CO_3$
$H_2 SO_4$
$NH_4^{+}$
Conjugate base
$OH^{-}$
$CO_3^{2-}$
$SO_4^{2-}$
$NH_2^{-}$
7.40 Classify the following species into Lewis acids and Lewis bases and show how these act as Lewis acid/base: (a) $\mathrm{OH}^{-}$(b) $\mathrm{F}^{-}$(c) $\mathrm{H}^{+}$(d) $\mathrm{BCl_3}$.
Show Answer
Answer
(a) $OH^{-}$is a Lewis base since it can donate its lone pair of electrons.
(b) $F^{-}$is a Lewis base since it can donate a pair of electrons.
(c) $H^{+}$is a Lewis acid since it can accept a pair of electrons.
(d) $BCl_3$ is a Lewis acid since it can accept a pair of electrons.
7.41 The concentration of hydrogen ion in a sample of soft drink is $3.8 \times 10^{-3} \mathrm{M}$. What is its $\mathrm{pH}$ ?
Show Answer
Answer
Given,
$[H^{+}]=3.8 \times 10^{-3} M$
$\therefore pH$ value of soft drink
$=-\log [H^{+}]$
$ \begin{aligned} & =-\log (3.8 \times 10^{-3}) \\ & =-\log 3.8-\log 10^{-3} \\ & =-\log 3.8+3 \\ & =-0.58+3 \\ & =2.42 \end{aligned} $
7.42 The $\mathrm{pH}$ of a sample of vinegar is 3.76. Calculate the concentration of hydrogen ion in it.
Show Answer
Answer
Given,
$pH=3.76$
It is known that,
$ \begin{aligned} & pH=-\log [H^{+}] \\ & \Rightarrow \log [H^{+}]=-pH \\ & \Rightarrow[H^{+}]=antilog(-pH) \\ & =\text{ antil og }(-3.76) \\ & =1.74 \times 10^{-4} M \end{aligned} $
Hence, the concentration of hydrogen ion in the given sample of vinegar is $1.74 \times 10^{-4} M$.
7.43 The ionization constant of $\mathrm{HF}, \mathrm{HCOOH}$ and $\mathrm{HCN}$ at $298 \mathrm{~K}$ are $6.8 \times 10^{-4}$, $1.8 \times 10^{-4}$ and $4.8 \times 10^{-9}$ respectively. Calculate the ionization constants of the corresponding conjugate base.
Show Answer
Answer
It is known that,
$K_b=\frac{K_w}{K_a}$
Given,
$K_a$ of $HF=6.8 \times 10^{-44}$
Hence, $K_b$ of its conjugate base $F^{-}$ $=\frac{K_w}{K_a}$
$=\frac{10^{-14}}{6.8 \times 10^{-4}}$
$=1.5 \times 10^{-11}$
Given,
$K_a$ of $HCOOH=1.8 \times 10^{-4 }$
Hence, $K_b$ of its conjugate base $HCOO^{-}$
$=\frac{K_w}{K_a}$
$=\frac{10^{-14}}{1.8 \times 10^{-4}}$
$=5.6 \times 10^{-11}$
Given,
$K _{\text{a }}$ of $HCN=4.8 \times 10^{-9}$
Hence, $K_b$ of its conjugate base $CN^{\text{ate- }}$
$=\frac{K_w}{K_a}$
$=\frac{10^{-14}}{4.8} \times 10^{-9}$
$=2.08 \times 10^{-6}$
7.44 The ionization constant of phenol is $1.0 \times 10^{-10}$. What is the concentration of phenolate ion in $0.05 \mathrm{M}$ solution of phenol? What will be its degree of ionization if the solution is also $0.01 \mathrm{M}$ in sodium phenolate?
Show Answer
Answer
Ionization of phenol:
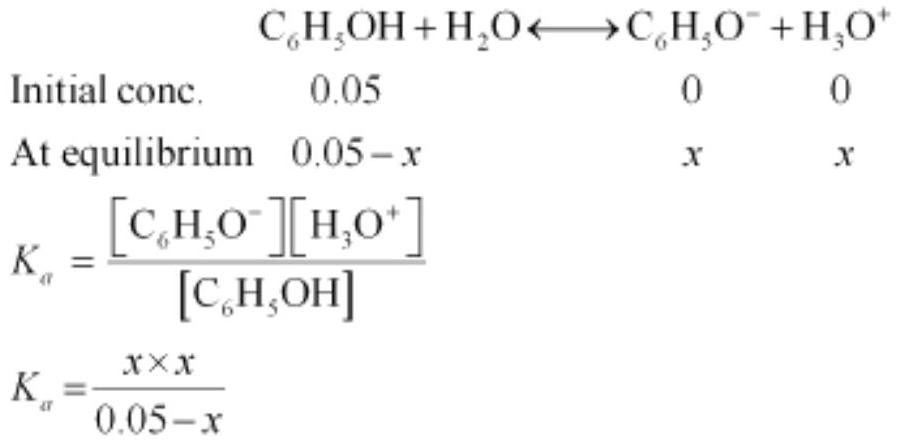
As the value of the ionization constant is very less, $x$ will be very small. Thus, we can ignore $x$ in the denominator.
$ \begin{aligned} \therefore x & =\sqrt{1 \times 10^{-10} \times 0.05} \\ & =\sqrt{5 \times 10^{-12}} \\ & =2.2 \times 10^{-6} M=[H_3 O^{+}] \end{aligned} $
Since $[H_3 O^{+}]=[C_6 H_5 O^{-}]$,
$[C_6 H_5 O^{-}]=2.2 \times 10^{-6} M$.
Now, let $\alpha$ be the degree of ionization of phenol in the presence of $0.01 M C_6 H_5 ONa$.
$ C_6 H_5 ONa \longrightarrow C_6 H_5 O^{-}+Na^{+} $
Conc.
$ 0.01 $
Also,
$ \begin{aligned} & \quad C_6 H_5 OH+H_2 O \longleftrightarrow C_6 H_5 O^{-}+H_3 O^{+} \\ & \text{Conc. } 0.05-0.05 \alpha \\ & {[C_6 H_5 OH]=0.05-0.05 \alpha ; 0.05 M} \end{aligned} $
$ \begin{aligned} & {[C_6 H_5 O^{-}]=0.01+0.05 \alpha ; 0.01 M} \\ & {[H_3 O^{+}]=0.05 \alpha} \\ & K_a=\frac{[C_6 H_5 O^{-}][H_3 O^{+}]}{[C_6 H_5 OH]} \\ & K_a=\frac{(0.01)(0.05 \alpha)}{0.05} \\ & 1.0 \times 10^{-10}=.01 \alpha \\ & \alpha=1 \times 10^{-8} \end{aligned} $
7.45 The first ionization constant of $\mathrm{H_2} \mathrm{~S}$ is $9.1 \times 10^{-8}$. Calculate the concentration of $\mathrm{HS}^{-}$ion in its $0.1 \mathrm{M}$ solution. How will this concentration be affected if the solution is $0.1 \mathrm{M}$ in $\mathrm{HCl}$ also? If the second dissociation constant of $\mathrm{H_2} \mathrm{~S}$ is $1.2 \times 10^{-13}$, calculate the concentration of $\mathrm{S}^{2-}$ under both conditions.
Show Answer
Answer
(i) To calculate the concentration of $HS^{-}$ ion:
Case I (in the absence of $HCl$ ):
Let the concentration of $HS^{-}$ be $x M$.
$ \begin{aligned} & H_2 S \longleftrightarrow H^{+}+HS^{-} \\ & \begin{matrix} C_i & 0.1 & 0 & 0 \end{matrix} \\ & \begin{matrix} C_f & 0.1-x & x & x \end{matrix} \\ & \text{ Then, } K _{a_1}=\frac{[H^{+}][HS^{-}]}{[H_2 S]} \\ & 9.1 \times 10^{-8}=\frac{(x)(x)}{0.1-x} \\ & (9.1 \times 10^{-8})(0.1-x)=x^{2} \end{aligned} $
Taking $0.1-x M ; 0.1 M$, we have $(9.1 \times 10^{-8})(0.1)=x^{2}$.
$ \begin{aligned} & 9.1 \times 10^{-9}=x^{2} \\ & x=\sqrt{9.1 \times 10^{-9}} \\ & \quad=9.54 \times 10^{-5} M \\ & \Rightarrow[HS^{-}]=9.54 \times 10^{-5} M \end{aligned} $
Case II (in the presence of $HCl$ ):
In the presence of $0.1 M$ of $HCl$, let $[HS^{-}]$be $y M$.
Then, $\quad H_2 S \longleftrightarrow HS^{-}+H^{+}$
$\begin{matrix} C_i & 0.1 & 0 & 0\end{matrix} $
$C_f \quad 0.1-y \quad y \quad y$
Also, $\quad HCl \longleftrightarrow H^{+}+Cl^{-}$
$0.1 \quad 0.1$
Now, $K _{a_1}=\frac{[HS^{-}][H^{+}]}{[H_2 S]}$
$K_{a_1}=\frac{[y] (0.1+y)}{(0.1-y)}$
$9.1 \times 10^{-8}=\frac{y \times 0.1}{0.1} \quad(\because 0.1-y ; 0.1 M)$ (and $0.1+y ; 0.1 M$ )
$9.1 \times 10^{-8}=y$
$\Rightarrow[HS^{-}]=9.1 \times 10^{-8}$
(ii) To calculate the concentration of $[S^{2-}]$ :
Case I (in the absence of $0.1 M HCl$ ):
$HS^{-} \longleftrightarrow H^{+}+S^{2-}$
$[HS^{-}]=9.54 \times 10^{-5} M$ (From first ionization, case I)
Let $[S^{2-}]$ be $X$.
Also, $[H^{+}]=9.54 \times 10^{-5} M$ (From first ionization, case I)
$K _{a_2}=\frac{[H^{+}][S^{2-}]}{[HS^{-}]}$
$K _{a_2}=\frac{(9.54 \times 10^{-5})(X)}{9.54 \times 10^{-5}}$
$1.2 \times 10^{-13}=X=[S^{2-}]$
Case II (in the presence of $0.1 M HCl$ ):
Again, let the concentration of $HS^{-}$ be $X^{\prime} M$.
$ \begin{aligned} & {[HS^{-}]=9.1 \times 10^{-8} M \text{ (From first ionization, case II) }} \\ & {[H^{+}]=0.1 M _{(\text{From } HCl, \text{ case II) }}} \end{aligned} $
$ \begin{aligned} & {[S^{2-}]=X^{\prime}} \\ & \text{ Then, } K _{a_2}=\frac{[H^{+}][S^{2-}]}{[HS^{-}]} \\ & 1.2 \times 10^{-13}=\frac{(0.1)(X^{\prime})}{9.1 \times 10^{-8}} \\ & 10.92 \times 10^{-21}=0.1 X^{\prime} \\ & \frac{10.92 \times 10^{-21}}{0.1}=X^{\prime} \\ & X^{\prime}=\frac{1.092 \times 10^{-20}}{0.1} \\ & =1.092 \times 10^{-19} M \\ & \Rightarrow K _{a_1}=1.74 \times 10^{-5} \end{aligned} $
7.46 The ionization constant of acetic acid is $1.74 \times 10^{-5}$. Calculate the degree of dissociation of acetic acid in its $0.05 \mathrm{M}$ solution. Calculate the concentration of acetate ion in the solution and its $\mathrm{pH}$.
Show Answer
Answer
Method 1
- $\quad CH_3 COOH \longleftrightarrow CH_3 COO^{-}+H^{+} \quad K_a=1.74 \times 10^{-5}$
- $H_2 O+H_2 O \longleftrightarrow H_3 O^{+}+OH^{-} \quad K_w=1.0 \times 10^{-14}$
Since $K a \gg K_w$ :
$ \begin{matrix} CH_3 COOH+H_2 O \longleftrightarrow CH_3 COO^{-}+H_3 O^{+} \\ 0.05 & 0 & 0 \\ 0.05-.05 \alpha & 0.05 \alpha & 0.05 \alpha \\ K_a & =\frac{(.05 \alpha)(.05 \alpha)}{(.05-0.05 \alpha)} \\ & =\frac{(.05 \alpha)(0.05 \alpha)}{.05(1-\alpha)} \\ & =\frac{.05 \alpha^{2}}{1-\alpha} \end{matrix} $
$ \begin{aligned} & 1.74 \times 10^{-5}=\frac{0.05 \alpha^{2}}{1-\alpha} \\ & 1.74 \times 10^{-5}-1.74 \times 10^{-5} \alpha=0.05 \alpha^{2} \\ & 0.05 \alpha^{2}+1.74 \times 10^{-5} \alpha-1.74 \times 10^{-5} \\ & D=b^{2}-4 a c \\ & =(1.74 \times 10^{-5})^{2}-4(.05)(1.74 \times 10^{-5}) \\ & =3.02 \times 10^{-25}+.348 \times 10^{-5} \\ & \alpha=\sqrt{\frac{K_a}{c}} \\ & \alpha=\sqrt{\frac{1.74 \times 10^{-5}}{.05}} \\ & =\sqrt{\frac{34.8 \times 10^{-5} \times 10}{10}} \\ & =\sqrt{3.48 \times 10^{-6}} \\ & =CH_3 COOH \longleftrightarrow CH_3 COO^{-}+H^{+} \end{aligned} $
$\alpha \underline{1.86 \times 10^{-3}}$
$ \begin{aligned} {[CH_3 COO^{-}] } & =0.05 \times 1.86 \times 10^{-3} \\ & =\frac{0.93 \times 10^{-3}}{1000} \\ & =.000093 \end{aligned} $
Method 2
Degree of dissociation,
$\alpha=\sqrt{\frac{K_a}{c}}$
$c=0.05 M$
$K_a=1.74 \times 10^{-5}$
Then, $\alpha=\sqrt{\frac{1.74 \times 10^{-5}}{.05}}$
$ \begin{aligned} & \alpha=\sqrt{34.8 \times 10^{-5}} \\ & \alpha=\sqrt{3.48} \times 10^{-4} \\ & \alpha=1.8610^{-2} \end{aligned} $
$CH_3 COOH \longleftrightarrow CH_3 COO^{-}+H^{+}$
Thus, concentration of $CH_3 COO^-=$ c.a
$ \begin{aligned} & =.05 \times 1.86 \times 10^{-2} \\ & =.093 \times 10^{-2} \\ & =.00093 M \\ & \text{ Since }[oAc^{-}]=[H^{+}] \\ & {[H^{+}]=.00093=.093 \times 10^{-2} .} \\ & pH=-\log [H^{+}] \\ & =-\log (.093 \times 10^{-2}) \\ & \therefore pH=3.03 \end{aligned} $
Hence, the concentration of acetate ion in the solution is $0.00093 M$ and its $Ph$ is 3.03 .
7.47 It has been found that the $\mathrm{pH}$ of a $0.01 \mathrm{M}$ solution of an organic acid is 4.15. Calculate the concentration of the anion, the ionization constant of the acid and its $\mathrm{p} K_{\mathrm{a}}$.
Show Answer
Answer
Let the organic acid be HA.
$\Rightarrow HA \longleftrightarrow H^{+}+A^{-}$
Concentration of $HA=0.01 M$
$pH=4.15$
$-\log [H^{+}]=4.15$
$[H^{+}]=7.08 \times 10^{-5}$
$K_a=\frac{[H^{+}][A^{-}]}{[HA]}$
$[H^{+}]=[A^{-}]=7.08 \times 10^{-5}$
$[HA]=0.01$
Then,
$ \begin{aligned} K_a & =\frac{(7.08 \times 10^{-5})(7.08 \times 10^{-5})}{0.01} \\ K_a & =5.01 \times 10^{-7} \\ p K_a & =-\log K_a \\ & =-\log (5.01 \times 10^{-7}) \\ p K_a & =6.3001 \end{aligned} $
7.48 Assuming complete dissociation, calculate the $\mathrm{pH}$ of the following solutions:
(a) $0.003 \mathrm{M} \mathrm{HCl}$
(b) $0.005 \mathrm{M} \mathrm{NaOH}$
(c) $0.002 \mathrm{M} \mathrm{HBr}$
(d) $0.002 \mathrm{M} \mathrm{KOH}$
Show Answer
Answer
(i) $0.003 MHCl$ :
$H_2 O+HCl \longleftrightarrow H_3 O^{+}+Cl^{-}$
Since $HCl$ is completely ionized,
$ \begin{aligned} & {[H_3 O^{+}]=[HCl] .} \\ & \Rightarrow[H_3 O^{+}]=0.003 \end{aligned} $
Now,
$ \begin{aligned} pH & =-\log [H_3 O^{+}]=-\log (.003) \\ & =2.52 \end{aligned} $
Hence, the $pH$ of the solution is 2.52 .
(ii) $0.005 MNaOH$ :
$ \begin{aligned} & NaOH _{(a q)} \longleftrightarrow Na _{(a q)}^{+}+HO _{(a q)}^{-} \\ & {[HO^{-}]=[NaOH]} \\ & \Rightarrow[HO^{-}]=.005 \\ & pOH=-\log [HO^{-}]=-\log (.005) \\ & pOH=2.30 \\ & \therefore pH=14-2.30 \\ & \quad=11.70 \end{aligned} $
Hence, the $pH$ of the solution is 11.70 .
(iii) $0.002 HBr$ :
$ \begin{aligned} & HBr+H_2 O \longleftrightarrow H_3 O^{+}+Br^{-} \\ & {[H_3 O^{+}]=[HBr]} \\ & \Rightarrow[H_3 O^{+}]=.002 \\ & \therefore pH=-\log [H_3 O^{+}] \\ & \quad=-\log (0.002) \\ & \quad=2.69 \end{aligned} $
Hence, the $pH$ of the solution is 2.69 .
(iv) $0.002 M KOH$ :
$ \begin{aligned} & KOH _{(a q)} \longleftrightarrow K _{(a q)}^{+}+OH _{(a q)}^{-} \\ & {[OH^{-}]=[KOH]} \\ & \Rightarrow[OH^{-}]=.002 \\ & \text{ Now, pOH }=-\log [OH^{-}] \\ & \quad=2.69 \\ & \begin{matrix} \therefore pH=14-2.69 \\ =11.31 \end{matrix} \end{aligned} $
Hence, the $pH$ of the solution is 11.31 .
7.49 Calculate the $\mathrm{pH}$ of the following solutions:
a) $2 \mathrm{~g}$ of $\mathrm{T1OH}$ dissolved in water to give 2 litre of solution.
b) $0.3 \mathrm{~g}$ of $\mathrm{Ca}(\mathrm{OH})_{2}$ dissolved in water to give $500 \mathrm{~mL}$ of solution.
c) $0.3 \mathrm{~g}$ of $\mathrm{NaOH}$ dissolved in water to give $200 \mathrm{~mL}$ of solution.
d) $1 \mathrm{~mL}$ of $13.6 \mathrm{M} \mathrm{HCl}$ is diluted with water to give 1 litre of solution.
Show Answer
Answer
(a) For $2 g$ of $TIOH$ dissolved in water to give $2 L$ of solution:
$ \begin{aligned} {[TlOH _{(a q)}] } & =\frac{2}{2} g / L \\ & =\frac{2}{2} \times \frac{1}{221} M \\ & =\frac{1}{221} M \end{aligned} $
$TlOH _{(a q)} \longrightarrow Tl _{(a q)}^{+}+OH _{(a q)}^{-}$
$[OH _{(a q)}^{-}]=[TlOH _{(o q)}]=\frac{1}{221} M$
$K_w=[H^{+}][OH^{-}]$
$10^{-14}=[H^{+}] (\frac{1}{221})$
$221 \times 10^{-14}=[H^{+}]$
$ \begin{aligned} \Rightarrow pH=-\log [H^{+}] & =-\log (221 \times 10^{-14}) \\ & =-\log (2.21 \times 10^{-12}) \\ & =11.65 \end{aligned} $
(b) For $0.3 g$ of $Ca(OH)_2$ dissolved in water to give $500 mL$ of solution:
$ \begin{aligned} & Ca(OH)_2 \longrightarrow Ca^{2+}+2 OH^{-} \\ & {[Ca(OH)_2]=0.3 \times \frac{1000}{500}=0.6 M} \\ & {[OH^{-} _{a q}]=2 \times[Ca(OH) _{2 a q}]=2 \times 0.6} \\ & =1.2 M \\ & {[H^{+}]=\frac{K_w}{[OH _{a q}^{-}]}} \\ & =\frac{10-14}{1.2} M \\ & =0.833 \times 10^{-14} \\ & pH=-\log (0.833 \times 10^{-14}) \\ & =-\log (8.33 \times 10^{-13}) \\ & =(-0.902+13) \\ & =12.098 \end{aligned} $
(c) For $0.3 g$ of $NaOH$ dissolved in water to give $200 mL$ of solution: $NaOH \longrightarrow Na _{(a q)}^{+}+OH _{(a q)}^{-}$
$[NaOH]=0.3 \times \frac{1000}{200}=1.5 M$
$[OH^{-} _{a q}]=1.5 M$
Then, $[H^{+}]=\frac{10^{-14}}{1.5}$
$ =6.66 \times 10^{-13} $
$pH=-\log (6.66 \times 10^{-13})$
$ =12.18 $
(d) For $1 mL$ of $13.6 M HCl$ diluted with water to give $1 L$ of solution:
$13.6 \times 1 mL=M_2 \times 1000 mL$
(Before dilution) (After dilution)
$13.6 \times 10^{-43}=M_2 \times 1 L$
$M_2=1.36 \times 10^{-2}$
$[H^{+}]=1.36 \times 10^{\text{-2 }}$
$pH=- \log (1.36 \times 10^{-2})$
$=(- 0.1335+2)$
$=1.866 - 41.87$
7.50 The degree of ionization of a $0.1 \mathrm{M}$ bromoacetic acid solution is 0.132 . Calculate the $\mathrm{pH}$ of the solution and the $p K_{\mathrm{a}}$ of bromoacetic acid.
Show Answer
Answer
Degree of ionization, $\alpha=0.132$
Concentration, $c=0.1 M$
Thus, the concentration of $H_3 O^{+}=c . \alpha$
$=0.1 \times 0.132$
$=0.0132$
$ \begin{aligned} pH & =-\log [H^{+}] \\ & =-\log (0.0132) \\ & =1.879: 1.88 \end{aligned} $
Now,
$ \begin{aligned} K_a & =C \alpha^{2} \\ & =0.1 \times(0.132)^{2} \\ K_a & =.0017 \\ p K_a & =2.75 \end{aligned} $
7.51 The $\mathrm{pH}$ of $0.005 \mathrm{M}$ codeine $\left(\mathrm{C_18} \mathrm{H_21} \mathrm{NO_3}\right)$ solution is 9.95. Calculate its ionization constant and $\mathrm{p} K_{\mathrm{b}}$.
Show Answer
Answer
$c=0.005$
$pH=9.95$
$pOH=4.05$
$pH=- \log (4.105)$
$4.05=-\log [OH^{-}]$
$[OH^{-}]=8.91 \times 10^{-5}$
$c \alpha=8.91 \times 10^{-5}$
$\alpha=\frac{8.91 \times 10^{-5}}{5 \times 10^{-3}}=1.782 \times 10^{-2}$
Thus, $K_b=c \alpha^{2}$
$ \begin{aligned} & =0.005 \times(1.782)^{2} \times 10^{-4} \\ & =0.005 \times 3.1755 \times 10^{-4} \\ & =0.0158 \times 10^{-4} \end{aligned} $
$K_b=1.58 \times 10^{-6}$
$P k_b=-\log K_b$
$=-\log (1.58 \times 10^{-6})$
$=5.80$
7.52 What is the $\mathrm{pH}$ of $0.001 \mathrm{M}$ aniline solution? The ionization constant of aniline can be taken from Table 6.7. Calculate the degree of ionization of aniline in the solution. Also calculate the ionization constant of the conjugate acid of aniline.
Show Answer
Answer
$K_b=4.27 \times 10^{-10}$
$c=0.001 M$
$pH=$ ?
$\alpha=$ ?
$k_b=c \alpha^{2}$
$4.27 \times 10^{-10}=0.001 \times \alpha^{2}$
$4270 \times 10^{-10}=\alpha^{2}$
$65.34 \times 10^{-5}=\alpha=6.53 \times 10^{-4}$
Then, $[$ anion $]=c \alpha=.001 \times 65.34 \times 10^{-5}$
$ =.065 \times 10^{-5} $
$pOH=-\log (.065 \times 10^{-5})$
$ =6.187 $
$pH=7.813$
Now,
$K_a \times K_b=K_w$
$\therefore 4.27 \times 10^{-10} \times K_a=K_w$
$K_a=\frac{10^{-14}}{4.27 \times 10^{-10}}$
$ =2.34 \times 10^{-5} $
Thus, the ionization constant of the conjugate acid of aniline is $2.34 \times 10^{- 5}$.
7.53 Calculate the degree of ionization of $0.05 \mathrm{M}$ acetic acid if its $\mathrm{p} K_{\mathrm{a}}$ value is 4.74. How is the degree of dissociation affected when its solution also contains (a) $0.01 \mathrm{M}$ (b) $0.1 \mathrm{M}$ in $\mathrm{HCl}$ ?
Show Answer
Answer
$ \begin{aligned} & c=0.05 M \\ & p K_a=4.74 \\ & p K_a=-\log (K_a) \\ & K_a=1.82 \times 10^{-5} \\ & K_a=c \alpha^{2} \quad \alpha=\sqrt{\frac{K_a}{c}} \\ & \alpha=\sqrt{\frac{1.82 \times 10^{-5}}{5 \times 10^{-2}}}=1.908 \times 10^{-2} \end{aligned} $
When $HCl$ is added to the solution, the concentration of $H^{+}$ions will increase. Therefore, the equilibrium will shift in the backward direction i.e., dissociation of acetic acid will decrease.
Case I: When $0.01 M HCl$ is taken.
Let $x$ be the amount of acetic acid dissociated after the addition of $HCl$.
| $CH_3 COOH$ | $\longleftrightarrow$ | $H^{+}$ | + | |
|---|---|---|---|---|
| Initial conc. | $0.05 M$ | 0 | 0 | |
| After dissociation | $0.05-x$ | $0.01+x$ | $x$ |
As the dissociation of a very small amount of acetic acid will take place, the values i.e., 0.05 - $x$ and $0.01+x$ can be taken as 0.05 and 0.01 respectively.
$ \begin{aligned} K_a & =\frac{[CH_3 COO^{-}][H^{+}]}{[CH_3 COOH]} \\ \therefore K_a & =\frac{(0.01) x}{0.05} \\ x & =\frac{1.82 \times 10^{-5} \times 0.05}{0.01} \\ x & =1.82 \times 10^{-3} \times 0.05 M \end{aligned} $
Now,
$ \begin{aligned} & =\frac{1.82 \times 10^{-3} \times 0.05}{0.05} \\ & =1.82 \times 10^{-3} \end{aligned} $
$ \alpha=\frac{\text{ Amount of acid dissociated }}{\text{ Amount of acid taken }} $
Case II: When $0.1 M HCl$ is taken.
Let the amount of acetic acid dissociated in this case be $X$. As we have done in the first case, the concentrations of various species involved in the reaction are:
$ \begin{aligned} & {[CH_3 COOH]=0.05-X ; 0.05 M} \\ & {[CH_3 COO^{-}]=X} \\ & {[H^{+}]=0.1+X ; 0.1 M} \\ & K_a=\frac{[CH_3 COO^{-}][H^{+}]}{[CH_3 COOH^{-}]} \\ & \therefore K_a=\frac{(0.1) X}{0.05} \\ & x=\frac{1.82 \times 10^{-5} \times 0.05}{0.1} \\ & x=1.82 \times 10^{-4} \times 0.05 M \end{aligned} $
Now,
$ \begin{aligned} \alpha & =\frac{\text{ Amount of acid dissociated }}{\text{ Amount of acid taken }} \\ & =\frac{1.82 \times 10^{-4} \times 0.05}{0.05} \\ & =1.82 \times 10^{-4} \end{aligned} $
7.54 The ionization constant of dimethylamine is $5.4 \times 10^{-4}$. Calculate its degree of ionization in its $0.02 \mathrm{M}$ solution. What percentage of dimethylamine is ionized if the solution is also $0.1 \mathrm{M}$ in $\mathrm{NaOH}$ ?
Show Answer
Answer
$K_b=5.4 \times 10^{-4}$
$c=0.02 M$
Then, $\alpha=\sqrt{\frac{K_b}{c}}$
$ \begin{aligned} & =\sqrt{\frac{5.4 \times 10^{-4}}{0.02}} \\ & =0.1643 \end{aligned} $
Now, if $0.1 M$ of $NaOH$ is added to the solution, then $NaOH$ (being a strong base) undergoes complete ionization.
$ \begin{aligned} & NaOH _{(a q)} \longleftrightarrow Na _{(a q)}^{+}+OH _{(a q)}^{-} \\ & 0.1 M \quad 0.1 M \end{aligned} $
And, $(CH_3)_2 NH$ $H_2 O$ $\longleftrightarrow$ $(0.02-x)$ $; 0.02 M$ $(CH_3)_2 NH_2^{+}+\overline{O} H$ $x$ $x$ $; 0.1 M$
Then, $[(CH_3)_2 NH_2^{+}]=x$
$[OH^{-}]=x+0.1 ; 0.1$
$\Rightarrow K_b=\frac{[(CH_3)_2 NH_2^{+}][OH^{-}]}{[(CH_3)_2 NH]}$
$5.4 \times 10^{-4}=\frac{x \times 0.1}{0.02}$
$x=0.0054$
It means that in the presence of $0.1 M NaOH, 0.54 %$ of dimethylamine will get dissociated.
7.55 Calculate the hydrogen ion concentration in the following biological fluids whose $\mathrm{pH}$ are given below:
(a) Human muscle-fluid, 6.83
(b) Human stomach fluid, 1.2
(c) Human blood, 7.38
(d) Human saliva, 6.4.
Show Answer
Answer
(a) Human muscle fluid 6.83:
$pH=6.83$
$pH=-\log [H^{+}]$
$\therefore 6.83=-\log [H^{+}]$
$[H^{+}]=1.48 \times 10^{-7} M$
(b) Human stomach fluid, 1.2:
$pH=1.2$
$1.2=-\log [H^{+}]$
$\therefore[H^{+}]=0.063$
(c) Human blood, 7.38:
$pH=7.38=-\log [H^{+}]$ $\therefore[H^{+}]=4.17 \times 10^{-8} M$
(d) Human saliva, 6.4:
$pH=6.4$
$6.4=-\log [H^{+}]$
$[H^{+}]=3.98 \times 10^{-7}$
7.56 The pH of milk, black coffee, tomato juice, lemon juice and egg white are 6.8, 5.0, 4.2, 2.2 and 7.8 respectively. Calculate corresponding hydrogen ion concentration in each.
Show Answer
Answer
The hydrogen ion concentration in the given substances can be calculated by using the given relation:
$pH=-\log [H^{+}]$
(i) $pH$ of milk $=6.8$
Since, $pH=a - \log [H^{+}]$
$6.8=- \log [H^{+}]$
$\log [H^{+}]=$- 6.8
$[H^{+}]=anitlog(-8)$
$=1.5 \times 10^{-7} M$
(ii) $pH$ of black coffee $=5.0$
Since, $pH=a - \log [H^{+}]$
$5.0=\hat{a} - \log [H^{+}]$
$\log [H^{+}]=$- 5.0
$[H^{+}]=anitlog(-5.0)$
$=10^{-5} M$
(iii) $pH$ of tomato juice $=4.2$
Since, $pH=- \log [H^{+}]$
$4.2= - \log [H^{+}]$
$\log [H^{+}]=-{4.2}$
$[H^{+}]=anitlog(4.2)$
$=6.31 \times 10^{-5} M$
(iv) $pH$ of lemon juice $=2.2$
Since, $pH=- \log [H^{+}]$ $2.2=- \log [H^{+}]$
$\log [H^{+}]=- 2.2$
$[H^{+}]=anitlog(- 2)$
$=6.31 \times 10^{-3} M$
(v) $pH$ of egg white $=7.8$
Since, $pH=- \log [H^{+}]$
$7.8=- \log [H^{+}]$
$\log [H^{+}]=- 7.8$
$[H^{+}]=anitlog(-7.8)$
$=1.58 \times 10^{-8} M$
7.57 If $0.561 \mathrm{~g}$ of $\mathrm{KOH}$ is dissolved in water to give $200 \mathrm{~mL}$ of solution at $298 \mathrm{~K}$. Calculate the concentrations of potassium, hydrogen and hydroxyl ions. What is its $\mathrm{pH}$ ?
Show Answer
Answer
$[KOH _{a q}]=\frac{0.561}{\frac{1}{5}} g / L$
$=2.805 g / L$
$=2.805 \times \frac{1}{56.11} M$
$=.05 M$
$KOH _{(a q)} \to K _{(a q)}^{+}+OH^{-} _{(a q)}$
$[OH^{-}]=.05 M=[K^{+}]$
$[H^{+}][H^{-}]=K_w$
$[H^{+}] \frac{K_w}{[OH^{-}]}$
$=\frac{10^{-14}}{0.05}=2 \times 10^{-13} M$
$\therefore pH=12.70$
7.58 The solubility of $\mathrm{Sr}(\mathrm{OH})_{2}$ at $298 \mathrm{~K}$ is $19.23 \mathrm{~g} / \mathrm{L}$ of solution. Calculate the concentrations of strontium and hydroxyl ions and the $\mathrm{pH}$ of the solution.
Show Answer
Answer
Solubility of $Sr(OH)_2=19.23 g / L$
Then, concentration of $Sr(OH)_2$
$=\frac{19.23}{121.63} M$
$=0.1581 M$
$Sr(OH) _{2(a q)} \longrightarrow Sr^{2+} _{(a q)}+2(OH^{-}) _{(a q)}$
$\therefore[Sr^{2+}]=0.1581 M$
$[OH^{-}]=2 \times 0.1581 M=0.3126 M$
Now,
$K_w=[OH^{-}][H^{+}]$
$\frac{10^{-14}}{0.3126}=[H^{+}]$
$\Rightarrow[H^{+}]=3.2 \times 10^{-14}$
$\therefore pH=13.495 \approx 13.50$
7.59 The ionization constant of propanoic acid is $1.32 \times 10^{-5}$. Calculate the degree of ionization of the acid in its $0.05 \mathrm{M}$ solution and also its $\mathrm{pH}$. What will be its degree of ionization if the solution is $0.01 \mathrm{M}$ in $\mathrm{HCl}$ also?
Show Answer
Answer
Let the degree of ionization of propanoic acid be $\alpha$.
Then, representing propionic acid as HA, we have:
$ \begin{aligned} & HA+H_2 O \longleftrightarrow H_3 O^{+}+A^{-} \\ & (.05-0.0 \alpha) \approx .05 \end{aligned} $
$ \begin{gathered} .05 \alpha \\ K_a=\frac{[H_3 O^{+}][A^{-}]}{[HA]} \\ \quad=\frac{(.05 \alpha)(.05 \alpha)}{0.05}=.05 \alpha^{2} \\ \alpha=\sqrt{\frac{K_a}{.05}}=1.63 \times 10^{-2} \\ \text{ Then, }[H_3 O^{+}]=.05 \alpha=.05 \times 1.63 \times 10^{-2}=K_b .15 \times 10^{-4} M \\ \therefore pH=3.09 \end{gathered} $
In the presence of $0.1 M$ of $HCl$, let $\alpha^{\prime}$ be the degree of ionization.
$ \begin{aligned} & \text{ Then, }[H_3 O^{+}]=0.01 \\ & {[A^{-}]=005 \alpha^{\prime}} \\ & {[HA]=.05} \\ & K_a=\frac{0.01 \times .05 \alpha^{\prime}}{.05} \\ & 1.32 \times 10^{-5}=.01 \times \alpha^{\prime} \\ & \alpha^{\prime}=1.32 \times 10^{-3} \end{aligned} $
7.60 The $\mathrm{pH}$ of $0.1 \mathrm{M}$ solution of cyanic acid (HCNO) is 2.34. Calculate the ionization constant of the acid and its degree of ionization in the solution.
Show Answer
Answer
$c=0.1 M$
$pH=2.34$ $-\log [H^{+}]=pH$
$-\log [H^{+}]=2.34$
$[H^{+}]=4.5 \times 10^{-3}$
Also,
$[H^{+}]=c \alpha$
$4.5 \times 10^{-3}=0.1 \times \alpha$
$\frac{4.5 \times 10^{-3}}{0.1}=\alpha$
$\alpha=45 \times 10^{-3}=.045$
Then,
$K_a=c \alpha^{2}$
$=0.1 \times(45 \times 10^{-3})^{2}$
$=202.5 \times 10^{-6}$
$=2.02 \times 10^{-4}$
7.61 The ionization constant of nitrous acid is $4.5 \times 10^{-4}$. Calculate the $\mathrm{pH}$ of $0.04 \mathrm{M}$ sodium nitrite solution and also its degree of hydrolysis.
Show Answer
Answer
$NaNO_2$ is the salt of a strong base $(NaOH)$ and a weak acid $(HNO_2)$.
$NO_2^{-}+H_2 O \longleftrightarrow HNO_2+OH^{-}$
$K_h=\frac{[HNO_2][OH^{-}]}{[NO_2^{-}]}$
$\Rightarrow \frac{K_w}{K_a}=\frac{10^{-14}}{4.5 \times 10^{-4}}=.22 \times 10^{-10}$
Now, If $x$ moles of the salt undergo hydrolysis, then the concentration of various species present in the solution will be: $[NO_2^{-}]=.04-x ; 0.04$
$[HNO_2]=x$
$[OH^{-}]=x$
$K_h=\frac{x^{2}}{0.04}=0.22 \times 10^{-10}$
$x^{2}=.0088 \times 10^{-10}$
$x=.093 \times 10^{-5}$
$\therefore[OH^{-}]=0.093 \times 10^{-5} M$
$[H_3 O^{+}]=\frac{10^{-14}}{.093 \times 10^{-5}}=10.75 \times 10^{-9} M$
$\Rightarrow pH=-\log (10.75 \times 10^{-9})$
$=7.96$
Therefore, degree of hydrolysis
$=\frac{x}{0.04}=\frac{.093 \times 10^{-5}}{.04}=2.325 \times 10^{- 5}$
7.62 A $0.02 \mathrm{M}$ solution of pyridinium hydrochloride has $\mathrm{pH}=3.44$. Calculate the ionization constant of pyridine.
Show Answer
Answer
$pH=3.44$
We know that,
$pH=- \log [H^{+}]$
$\therefore[H^{+}]=3.63 \times 10^{-4}$
Then, $K_h=\frac{(3.63 \times 10^{-4})^{2}}{0.02} \quad(\because$ concentration $=0.02 M)$
$\Rightarrow K_h=6.6 \times 10^{-6}$
Now, $K_h=\frac{K_w}{K_a}$
$\Rightarrow K_a=\frac{K_w}{K_h}=\frac{10^{-14}}{6.6 \times 10^{-6}}$
$=1.51 \times 10^{-9}$
7.63 Predict if the solutions of the following salts are neutral, acidic or basic:
$\mathrm{NaCl}, \mathrm{KBr}, \mathrm{NaCN}, \mathrm{NH_4} \mathrm{NO_3}, \mathrm{NaNO_2}$ and $\mathrm{KF}$
Show Answer
Answer
(i) $NaCl$ :
$ NaCl+H_2 O \longleftrightarrow \underset{\text{ Strong base }}{NaOH}+\underset{\text{ Strong acid }}{HCl} $
Therefore, it is a neutral solution.
(ii) $KBr$ :
$ KBr + H _2O \longleftrightarrow \underset{\text{ Strong base }}{KOH} + \underset{\text{ Strong acid }}{HBr} $
Therefore, it is a neutral solution.
(iii) $NaCN$ :
$ \begin{aligned} & NaCN+H_2 O \longleftrightarrow HCN+NaOH \\ & \text{ Weak acid Strong base } \end{aligned} $
Therefore, it is a basic solution.
(iv) $NH_4 NO_3$
$ NH_4 NO_3+H_2 O \longleftrightarrow \underset{\text{ Weak base }}{NH_4 OH+\underset{\text{ Strong acid }}{HNO_3}} $
Therefore, it is an acidic solution.
(v) $NaNO_2$
$ NaNO_2+H_2 O \longleftrightarrow \underset{\text{ Strong base }}{NaOH}+\underset{\text{ Weak acid }}{HNO_2} $
Therefore, it is a basic solution.
(vi) $KF$
$ \begin{aligned} & KF+H_2 O \longleftrightarrow KOH+HF \\ & \text{ Strong base Weak acid } \end{aligned} $
Therefore, it is a basic solution.
7.64 The ionization constant of chloroacetic acid is $1.35 \times 10^{-3}$. What will be the pH of $0.1 \mathrm{M}$ acid and its $0.1 \mathrm{M}$ sodium salt solution?
Show Answer
Answer
It is given that $K_a$ for $ClCH_2 COOH$ is $1.35 \times 10^{-33}$.
$ \begin{aligned} & \Rightarrow K_a=c \alpha^{2} \\ & \therefore \alpha=\sqrt{\frac{K_a}{c}} \\ & \quad=\sqrt{\frac{1.35 \times 10^{-3}}{0.1}} \quad(\therefore \text{ concentration of acid }=0.1 m) \\ & \begin{aligned} \alpha & =\sqrt{1.35 \times 10^{-2}} \\ & =0.116 \\ \therefore[H^{+}] & =c \alpha=0.1 \times 0.116 \\ \quad & =.0116 \\ \Rightarrow pH & =-\log [H^{+}]=1.94 \end{aligned} \end{aligned} $
$ClCH_2 COONa$ is the salt of a weak acid i.e., $ClCH_2 COOH$ and a strong base i.e., $NaOH$.
$ \begin{aligned} & ClCH_2 COO^{-}+H_2 O \longleftrightarrow ClCH_2 COOH+OH^{-} \\ & K_h=\frac{[ClCH_2 COOH][OH^{-}]}{[ClCH_2 COO^{-}]} \\ & K_h=\frac{K_w}{K_a} \\ & K_h=\frac{10^{-14}}{1.35 \times 10^{-3}} \\ & =0.740 \times 10^{-11} \\ & \text{ Also, } .K_h=\frac{x^{2}}{0.1} \quad (\text{ where } x \text{ is the concentration of } OH^{-} \text{and } ClCH_2 COOH) \\ & 0.740 \times 10^{-11}=\frac{x^{2}}{0.1} \\ & 0.074 \times 10^{-11}=x^{2} \\ & \Rightarrow x^{2}=0.74 \times 10^{-12} \\ & \begin{matrix} x=0.86 \times 10^{-6} \\ {[OH^{-}]=0.86 \times 10^{-6}} \\ \therefore[H^{+}]=\frac{K_w}{0.86 \times 10^{-6}} \\ \quad=\frac{10^{-14}}{0.86 \times 10^{-6}} \\ {[H^{+}]=1.162 \times 10^{-8}} \\ pH=-\log [H^{+}] \\ =7.94 \end{matrix} \end{aligned} $
7.65 Ionic product of water at $310 \mathrm{~K}$ is $2.7 \times 10^{-14}$. What is the $\mathrm{pH}$ of neutral water at this temperature?
Show Answer
Answer
Ionic product, $K_w=[H^{+}][OH^{-}]$
Let $[H^{+}]=x$.
Since $[H^{+}]=[OH^{-}], K_w=x^{2}$.
$\Rightarrow K_w$ at $310 K$ is $2.7 \times 10^{-14}$.
$\therefore 2.7 \times 10^{-14}=x^{2}$
$\Rightarrow x=1.64 \times 10^{-7}$
$\Rightarrow[H^{+}]=1.64 \times 10^{-7}$
$\Rightarrow pH=-\log [H^{+}]$
$=-\log [1.64 \times 10^{-7}]$
$=6.78$
Hence, the $pH$ of neutral water is 6.78 .
7.66 Calculate the $\mathrm{pH}$ of the resultant mixtures:
a) $10 \mathrm{~mL}$ of $0.2 \mathrm{M} \mathrm{Ca}(\mathrm{OH})_{2}+25 \mathrm{~mL}$ of $0.1 \mathrm{M} \mathrm{HCl}$
b) $10 \mathrm{~mL}$ of $0.01 \mathrm{M} \mathrm{H_2} \mathrm{SO_4}+10 \mathrm{~mL}$ of $0.01 \mathrm{M} \mathrm{Ca}(\mathrm{OH})_{2}$
c) $10 \mathrm{~mL}$ of $0.1 \mathrm{M} \mathrm{H_2} \mathrm{SO_4}+10 \mathrm{~mL}$ of $0.1 \mathrm{M} \mathrm{KOH}$
Show Answer
Answer
(a)
Moles of $H_3 O^{+}=\frac{25 \times 0.1}{1000}=.0025 mol$
Moles of $OH^{-}=\frac{10 \times 0.2 \times 2}{1000}=.0040 mol$
Thus, excess of $OH^{-}=.0015 mol$
$ \begin{aligned} & {[OH^{-}]=\frac{.0015}{35 \times 10^{-3}} mol / L=.0428} \\ & \begin{aligned} pOH & =-\log [OH] \\ & =1.36 \\ pH & =14-1.36 \\ & =12.63 \quad \text{ (not matched) } \end{aligned} \end{aligned} $
Moles of $H_3 O^{+}=\frac{2 \times 10 \times 0.01}{1000}=.0002 mol$
Moles of $OH^{-}=\frac{2 \times 10 \times .01}{1000}=.0002 mol$
Since there is neither an excess of $H_3 O^{+}$or $OH^{-}$, the solution is neutral. Hence, $pH=7$.
(c)
$ \text{Moles of } H _3O^+ = \frac{2\times 10\times 0.1}{1000} =.002 mol $
Moles of $OH^{-}=\frac{10 \times 0.1}{1000}=0.001 mol$
Excess of $H_3 O^{+}=.001 mol$
Thus, $[H_3 O^{+}]=\frac{.001}{20 \times 10^{-3}}=\frac{10^{-3}}{20 \times 10^{-3}}=.05$
$\therefore pH=-\log (0.05)$
$=1.30$
7.67 Determine the solubilities of silver chromate, barium chromate, ferric hydroxide, lead chloride and mercurous iodide at $298 \mathrm{~K}$ from their solubility product constants given in Table 6.9. Determine also the molarities of individual ions.
Show Answer
Answer
(1) Silver chromate:
$ Ag_2 CrO_4 \longrightarrow 2 Ag^{+}+CrO_4{ }^{2-} $
Then,
$K _{s p}=[Ag^{+}]^{2}[CrO_4{ }^{2-}]$
Let the solubility of $Ag_2 CrO_4$ be s.
$\Rightarrow[Ag^{+}] 2 s$ and $[CrO_4{ }^{2-}]=s$
Then,
$K _{s p}=(2 s)^{2} . s=4 s^{3}$
$\Rightarrow 1.1 \times 10^{-12}=4 s^{3}$
$.275 \times 10^{-12}=s^{3}$
$s=0.65 \times 10^{-4} M$
Molarity of $Ag^{+}=2 s=2 \times 0.65 \times 10^{{-4}}=1.30 \times 10^{{-4}} M$
Molarity of $CrO_4{ }^{2-}=s=0.65 \times 10^{- 4} M$
(2) Barium chromate:
$BaCrO_4 \longrightarrow Ba^{2+}+CrO_4{ }^{2-}$
Then, $K _{s p}=[Ba^{2+}][CrO_4{ }^{2-}]$
Let $s$ be the solubility of $BaCrO_4$.
Thus, $[Ba^{2+}]=s$ and $[CrO_4^{2-}]=s$
$\Rightarrow K _{S P}=s^{2}$
$\Rightarrow 1.2 \times 10^{-10}=s^{2}$
$\Rightarrow s=1.09 \times 10^{-5} M$
Molarity of $Ba^{2+}=$ Molarity of $CrO_4{ }^{2-}=s=1.09 \times 10^{-5} M$
(3) Ferric hydroxide:
$Fe(OH)_3 \longrightarrow Fe^{2+}+3 OH^{-}$
$K _{s p}=[Fe^{2+}][OH^{-}]^{3}$
Let $s$ be the solubility of $Fe(OH)_3$.
Thus, $[Fe^{3+}]=s$ and $[OH^{-}]=3 s$
$\Rightarrow K _{s p}=s .(3 s)^{3}$
$ =s .27 s^{3} $
$K _{s p}=27 s^{4}$
$1.0 \times 10^{-38}=27 s^{4}$
$.037 \times 10^{-38}=s^{4}$
$.00037 \times 10^{-36}=s^{4} \quad \Rightarrow 1.39 \times 10^{-10} M=S$
Molarity of $Fe^{3+}=s=1.39 \times 10^{-10} M$
Molarity of $OH^{-}=3 s=4.17 \times 10^{-10} M$
(4) Lead chloride:
$PbCl_2 \longrightarrow Pb^{2+}+2 Cl^{-}$
$K _{S P}=[Pb^{2+}][Cl^{-}]^{2}$
Let $K _{S P}$ be the solubility of $PbCl_2$. $[PB^{2+}]=s$ and $[Cl^{-}]=2 s$
Thus, $K _{s p}=s .(2 s)^{2}$
$=4 s^{3}$
$\Rightarrow 1.6 \times 10^{-5}=4 s^{3}$
$\Rightarrow 0.4 \times 10^{-5}=s^{3}$
$4 \times 10^{-6}=s^{3} \Rightarrow 1.58 \times 10^{-2} M=S .1$
Molarity of $PB^{2+}=s=1.58 \times 10^{-2} M$
Molarity of chloride $=2 s=3.16 \times 10^{-2} M$
(5) Mercurous iodid
6.68 The solubility product constant of $\mathrm{Ag_2} \mathrm{CrO_4}$ and $\mathrm{AgBr}$ are $1.1 \times 10^{-12}$ and $5.0 \times 10^{-13}$ respectively. Calculate the ratio of the molarities of their saturated solutions
Show Answer
Answer
Let $s$ be the solubility of $Ag_2 CrO_4$.
Then, $Ag_2 CrO_4 \longleftrightarrow Ag^{2+}+2 CrO_4^{-}$
$K _{s p}=(2 s)^{2} . s=4 s^{3}$
$1.1 \times 10^{-12}=4 s^{3}$
$s=6.5 \times 10^{-5} M$
Let $s^{\prime}$ be the solubility of AgBr.
$AgBr _{(s)} \longleftrightarrow Ag^{+}+Br^{-}$
$K _{s p}=s^{\prime 2}=5.0 \times 10^{-13}$
$\therefore s^{\prime}=7.07 \times 10^{-7} M$
Therefore, the ratio of the molarities of their saturated solution is $\frac{s}{s^{\prime}}=\frac{6.5 \times 10^{-5} M}{7.07 \times 10^{-7} M}=91.9$.
7.69 Equal volumes of $0.002 \mathrm{M}$ solutions of sodium iodate and cupric chlorate are mixed together. Will it lead to precipitation of copper iodate? (For cupric iodate $\left.K_{\text {sp }}=7.4 \times 10^{-8}\right)$.
Show Answer
Answer
When equal volumes of sodium iodate and cupric chlorate solutions are mixed together, then the molar concentrations of both solutions are reduced to half i.e., $0.001 M$.
Then,
$ \begin{aligned} & NalO_3 \to Na^{+}+10_3^{-} \\ & 0.001 M \quad 0.001 M \\ & Cu(ClO_3)_2 \to Cu^{2+}+2 ClO_3^{-} \\ & 0.001 M \quad 0.001 M \end{aligned} $
Now, the solubility equilibrium for copper iodate can be written as:
$ Cu(10_3)_2 \to Cu^{2+} _{(aq)}+210 _{3(aq)}^{-} $
Ionic product of copper iodate:
$ \begin{aligned} & =[Cu^{2+}][10_3^{-}]^{2} \\ & =(0.001)(0.001)^{2} \\ & =1 \times 10^{-9} \end{aligned} $
Since the ionic product $(1 \times 10^{{-9}})$ is less than $K _{s p}(7.4 \times 10^{- 8})$, precipitation will not occur.
7.70 The ionization constant of benzoic acid is $6.46 \times 10^{-5}$ and $K_{\mathrm{sp}}$ for silver benzoate is $2.5 \times 10^{-13}$. How many times is silver benzoate more soluble in a buffer of $\mathrm{pH} 3.19$ compared to its solubility in pure water?
Show Answer
Answer
Since $pH=3.19$,
$ [H_3 O^{+}]=6.46 \times 10^{-4} M $
$C_6 H_5 COOH+H_2 O \longleftrightarrow C_6 H_5 COO^{-}+H_3 O$
$K_a \frac{[C_6 H_5 COO^{-}][H_3 O^{+}]}{[C_6 H_5 COOH]}$
$ \frac{[C_6 H_5 COOH]}{[C_6 H_5 COO^{-}]}=\frac{[H_3 O^{+}]}{K_a}=\frac{6.46 \times 10^{-4}}{6.46 \times 10^{-5}}=10 $
Let the solubility of $C_6 H_5 COOAg$ be $x mol / L$.
Then,
$ \begin{aligned} & {[Ag^{+}]=x} \\ & {[C_6 H_5 COOH]+[C_6 H_5 COO^{-}]=x} \\ & 10[C_6 H_5 COO^{-}]+[C_6 H_5 COO^{-}]=x \\ & {[C_6 H_5 COO^{-}]=\frac{x}{11}} \\ & K _{s p}[Ag^{+}][C_6 H_5 COO^{-}] \\ & 2.5 \times 10^{-13}=x(\frac{x}{11}) \\ & x=1.66 \times 10^{-6} mol / L \end{aligned} $
Thus, the solubility of silver benzoate in a pH 3.19 solution is $ 1.66 \times 10^{-6} molL^{-1} $
Now, let the solubility of $C_6 H_5 COOAg$ be $x^{\prime} mol / L$.
Then, $[Ag^{+}]=x^{\prime} M$ and $[CH_3 COO^{-}]=x^{\prime} M$.
$K _{s p}=[Ag^{+}][CH_3 COO^{-}]$
$K _{s p}=(x^{\prime})^{2}$
$x^{\prime}=\sqrt{K _{s p}}=\sqrt{2.5 \times 10^{-13}}=5 \times 10^{-7} mol / L$
$\therefore \frac{x}{x^{\prime}}=\frac{1.66 \times 10^{-6}}{5 \times 10^{-7}}=3.32$
Hence, $C_6 H_5 COOAg$ is approximately 3.317 times more soluble in a low $pH$ solution.
7.71 What is the maximum concentration of equimolar solutions of ferrous sulphate and sodium sulphide so that when mixed in equal volumes, there is no precipitation of iron sulphide? (For iron sulphide, $K_{\mathrm{sp}}=6.3 \times 10^{-18}$ ).
Show Answer
Answer
Let the maximum concentration of each solution be $x mol / L$. After mixing, the volume of the concentrations of each solution will be reduced to half i.e., $\frac{x}{2}$.
$ \therefore[FeSO_4]=[Na_2 S]=\frac{x}{2} M $
Then, $[Fe^{2+}]=[FeSO_4]=\frac{x}{2} M$
Also, $[S^{2-}]=[Na_2 S]=\frac{x}{2} M$
$FeS _{(s)} \longleftrightarrow Fe^{2+} _{(a q)}+S^{2-} _{(a q)}$
$K _{s p}=[Fe^{2+}][S^{2-}]$
$6.3 \times 10^{-18}=(\frac{x}{2})(\frac{x}{2})$
$\frac{x^{2}}{4}=6.3 \times 10^{-18}$
$\Rightarrow x=5.02 \times 10^{-9}$
If the concentrations of both solutions are equal to or less than $5.02 \times 10^{ae 9} M$, then there will be no precipitation of iron sulphide.
7.72 What is the minimum volume of water required to dissolve $1 \mathrm{~g}$ of calcium sulphate at $298 \mathrm{~K}$ ? (For calcium sulphate, $K_{\mathrm{sp}}$ is $9.1 \times 10^{-6}$ ).
Show Answer
Answer
$CaSO _{4(s)} \longleftrightarrow Ca^{2+} _{(aq)}+ SO _{4(aq)}^{2-} $
$K _{s p}=[Ca^{2+}][SO_4^{2-}]$
Let the solubility of $CaSO_4$ be $s$.
Then, $K _{s p}=s^{2}$
$9.1 \times 10^{-6}=s^{2}$
$s=3.02 \times 10^{-3} mol / L$
Molecular mass of $CaSO_4=136 g / mol$
Solubility of $CaSO_4$ in gram $/ L=3.02 \times 10^{-3} \times 136$
$=0.41 g / L$
This means that we need $1 L$ of water to dissolve $0.41 g$ of $CaSO_4$
Therefore, to dissolve $1 g$ of $CaSO_4$ we require $=\frac{1}{0.41} L=2.44 L$ of water.
7.73 The concentration of sulphide ion in $0.1 \mathrm{M} \mathrm{HCl}$ solution saturated with hydrogen sulphide is $1.0 \times 10^{-19} \mathrm{M}$. If $10 \mathrm{~mL}$ of this is added to $5 \mathrm{~mL}$ of $0.04 \mathrm{M}$ solution of the following: $\mathrm{FeSO_4}, \mathrm{MnCl_2}, \mathrm{ZnCl_2}$ and $\mathrm{CdCl_2}$. in which of these solutions precipitation will take place?
Show Answer
Answer
For precipitation to take place, it is required that the calculated ionic product exceeds the $K _{s p}$ value.
Before mixing:
$ [S^{2-}]=1.0 \times 10^{-19} M[M^{2+}]=0.04 M $
volume $=10 mL \quad$ volume $=5 mL$
After mixing:
$ [S^{2-}]=? \quad[M^{2+}]=? $
volume $=(10+5)=15 mL \quad$ volume $=15 mL$
$ \begin{aligned} & {[S^{2-}]=\frac{1.0 \times 10^{-19} \times 10}{15}=6.67 \times 10^{-20} M} \\ & {[M^{2+}]=\frac{0.04 \times 5}{15}=1.33 \times 10^{-2} M} \end{aligned} $
$ \begin{aligned} \text{ Ionic product } & =[M^{2+}][S^{2-}] \\ & =(1.33 \times 10^{-2})(6.67 \times 10^{-20}) \\ & =8.87 \times 10^{-22} \end{aligned} $
This ionic product exceeds the $K _{s p}$ of $Zns$ and $CdS$. Therefore, precipitation will occur in $CdCl_2$ and $ZnCl_2$ solutions.










