Simple Harmonic Motion 5 Question 5
10. A point mass is subjected to two simultaneous sinusoidal displacements in $x$-direction, $x _1(t)=A \sin \omega t$ and
$x _2(t)=A \sin \omega t+\frac{2 \pi}{3}$. Adding a third sinusoidal displacement $x _3(t)=B \sin (\omega t+\varphi)$ brings the mass to a complete rest. The values of $B$ and $\varphi$ are
(2011)
(a) $\sqrt{2} A, \frac{3 \pi}{4}$
(b) $A, \frac{4 \pi}{3}$
(c) $\sqrt{3} A, \frac{5 \pi}{6}$
(d) $A, \frac{\pi}{3}$
Show Answer
Answer:
Correct Answer: 10. (b)
Solution:
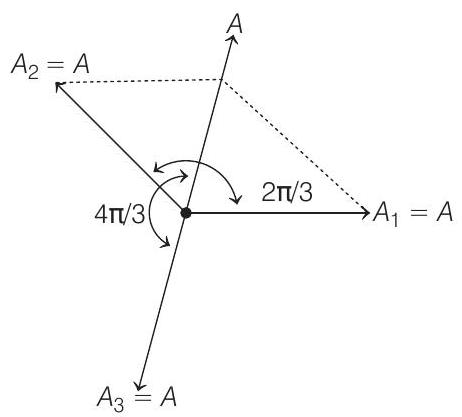
Resultant amplitude of $x _1$ and $x _2$ is $A$ at angle $\frac{\pi}{3}$ from $A _1$. To make resultant of $x _1, x _2$ and $x _3$ to be zero. $A _3$ should be equal to $A$ at angle $\varphi=\frac{4 \pi}{3}$ as shown in figure.
$\therefore$ Correct answer is (b).
Alternate Solution.
It we substitute, $x _1+x _2+x _3=0$
or $A \sin \omega t+A \sin \omega t+\frac{2 \pi}{3}+B \sin (\omega t+\varphi)=0$
Then by applying simple mathematics we can prove that
$$ B=A \quad \text { and } \quad \varphi=\frac{4 \pi}{3} $$






