SATHEE: Simple Harmonic Motion 4 Question 14

Simple Harmonic Motion 4 Question 14

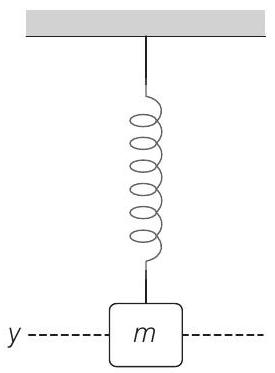14. A mass $m$ is undergoing SHM in the vertical direction about the mean position $y_{0}$ with amplitude $A$ and angular frequency $ω$ . At a distance $y$ from the mean position, the mass detaches from the spring. Assume that the spring contracts and does not obstruct the motion of $m$ . Find the distance $y$ (measured from

the mean position) such that the height $h$ attained by the block is maximum. $(A ω^{2} > g)$ .

(2005)

Show Answer

Answer:

Correct Answer: 14. $\frac{g}{ω^{2}}$

Solution:

At distance $y$ above the mean position velocity of the block.

$v = ω \sqrt{A^{2} - y^{2}}$

After detaching from the spring net downward acceleration of the block will be $g$ .

Therefore, total height attained by the block above the mean position,

$h = y + \frac{v^{2}}{2 g} = y + \frac{ω^{2} (A^{2} - y^{2})}{2 g}$

For $h$ to be maximum $d h / d y = 0$

Putting $\frac{d h}{d y} = 0$ , we get $y = \frac{g}{ω^{2}} = y_{max}$

Play Store

App Store

Ask SATHEE

Welcome to SATHEE !
Select from 'Menu' to explore our services, or ask SATHEE to get started. Let's embark on this journey of growth together! 🌐📚🚀🎓

I'm relatively new and can sometimes make mistakes.
If you notice any error, such as an incorrect solution, please use the thumbs down icon to aid my learning.
To begin your journey now, click on "I understand".

Simple Harmonic Motion 4 Question 14

Answer:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu