Simple Harmonic Motion 3 Question 8
8. A highly rigid cubical block $A$ of small mass $M$ and side $L$ is fixed rigidly on to another cubical block $B$ of the same dimensions and of low modulus of rigidity $\eta$ such that the lower face of $A$ completely covers the upper face of $B$. The lower face of $B$ is rigidly held on a horizontal surface. A small force $F$ is applied perpendicular to one of the side faces of $A$. After the force is withdrawn, block $A$ executes small oscillations, the time period of which is given by
(a) $2 \pi \sqrt{M \eta L}$
(b) $2 \pi \sqrt{\frac{M \eta}{L}}$
(c) $2 \pi \sqrt{\frac{M L}{\eta}}$
(d) $2 \pi \sqrt{\frac{M}{\eta L}}$
$(1992,2 M)$
Show Answer
Answer:
Correct Answer: 8. (d)
Solution:
- Modulus of rigidity, $\eta=F / A \theta$
Here,
$A=L^{2}$
and
$$ \theta=\frac{x}{L} $$
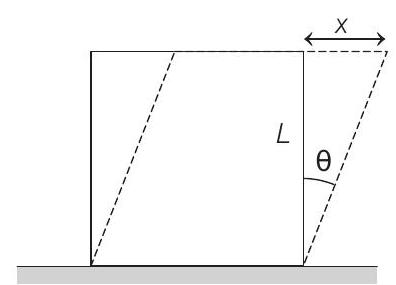
Therefore, restoring force is
$$ F=-\eta A \theta=-\eta L x $$
or acceleration, $a=\frac{F}{M}=-\frac{\eta L}{M} x$
Since, $a \propto-x$, oscillations are simple harmonic in nature, time period of which is given by
$$ \begin{aligned} T & =2 \pi \sqrt{\frac{\text { displacement }}{\text { acceleration }}} \\ & =2 \pi \sqrt{\frac{x}{a}}=2 \pi \sqrt{\frac{M}{\eta L}} \end{aligned} $$






