Rotation 5 Question 24
36. Three particles $A, B$ and $C$, each of mass $m$, are connected to each other by three massless rigid rods to form a rigid, equilateral triangular body of side $l$. This body is placed on a horizontal frictionless table $(x-y$ plane $)$ and is hinged to
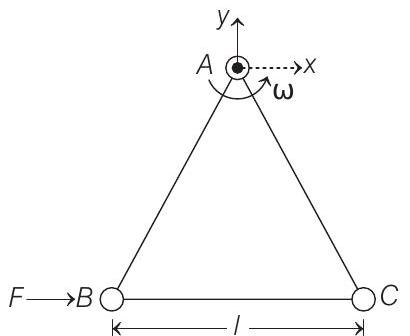it at the point $A$, so that it can move without friction about the vertical axis through $A$ (see figure). The body is set into rotational motion on the table about $A$ with a constant angular velocity $\omega$.
(2002, 5M)
(a) Find the magnitude of the horizontal force exerted by the hinge on the body.
(b) At time $T$, when the side $B C$ is parallel to the $X$-axis, a force $F$ is applied on $B$ along $B C$ (as shown). Obtain the $x$-component and the $y$-component of the force exerted by the hinge on the body, immediately after time $T$.
Show Answer
Answer:
Correct Answer: 36. (a) $\sqrt{3} m l \omega^2$
(b) $\left(F _{\text {net }}\right)_x=\frac{-F}{4},\left(F _{\text {net }}\right)_y=\sqrt{3} m l \omega^2$
Solution:
- (a) The distance of centre of mass (CM) of the system about point $A$ will be $r=\frac{l}{\sqrt{3}}$
Therefore, the magnitude of horizontal force exerted by the hinge on the body is
$F=$ centripetal force or $F=(3 m) r \omega^{2}$
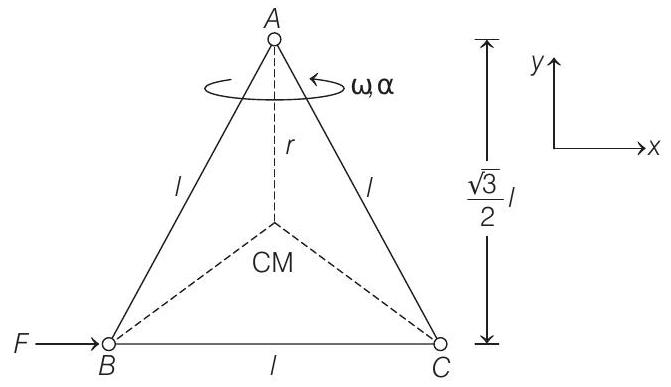
$$ \begin{aligned} & \text { or } \\ & F=(3 m) \frac{l}{\sqrt{3}} \omega^{2} \\ & \text { or } \\ & F=\sqrt{3} m l \omega^{2} \end{aligned} $$
(b) Angular acceleration of system about point $A$ is
$$ \alpha=\frac{\tau _A}{I _A}=\frac{(F) \frac{\sqrt{3}}{2} l}{2 m l^{2}}=\frac{\sqrt{3} F}{4 m l} $$
Now, acceleration of CM along $x$-axis is
$$ a _x=r \alpha=\frac{l}{\sqrt{3}} \quad \frac{\sqrt{3} F}{4 m l} \quad \text { or } \quad a _x=\frac{F}{4 m} $$
Let $F _x$ be the force applied by the hinge along $X$-axis.
$$ \begin{aligned} \text { Then, } & & F _x+F & =(3 m) a _x \\ \text { or } & & F _x+F & =(3 m) \frac{F}{4 m} \\ \text { or } & & F _x+F & =\frac{3}{4} F \\ \text { or } & & F _x & =-\frac{F}{4} \end{aligned} $$
Further if $F _y$ be the force applied by the hinge along $Y$-axis. Then,
$$ \begin{aligned} & F _y=\text { centripetal force } \\ & \text { or } \quad F _y=\sqrt{3} m l \omega^{2} \end{aligned} $$
