Rotation 4 Question 3
4. A cubical block of side $a$ moving with velocity $v$ on a horizontal smooth plane as shown. It hits a ridge at point $O$. The angular speed of the block after it hits $O$ is $\quad(1999,2 M)$
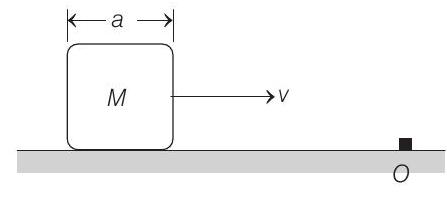
(a) $3 v / 4 a$
(b) $3 v / 2 a$
(c) $\sqrt{3} / \sqrt{2} a$
(d) zero
Objective Questions II (One or more correct option)
Show Answer
Answer:
Correct Answer: 4. (a)
Solution:
- $r=\sqrt{2} \frac{a}{2}$ or $r^{2}=\frac{a^{2}}{2}$
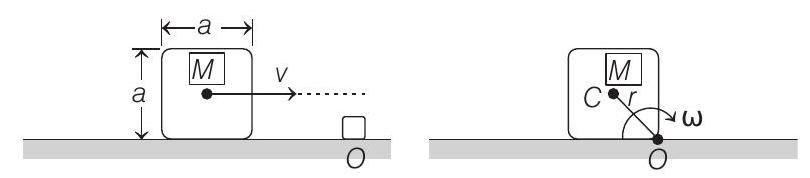
Net torque about $O$ is zero.
Therefore, angular momentum $(L)$ about $O$ will be conserved, or $L _i=L _f$
$$ \begin{aligned} M v \frac{a}{2} & =I _o \omega=\left(I _{CM}+M r^{2}\right) \omega \\ & =\frac{M a^{2}}{6}+M \frac{a^{2}}{2} \omega \\ & =\frac{2}{3} M a^{2} \omega \\ \omega & =\frac{3 v}{4 a} \end{aligned} $$






