Rotation 3 Question 8
14. The net external force acting on the disk when its centre of mass is at displacement $x$ with respect to its equilibrium position is
(a) $-k x$
(b) $-2 k x$
(c) $-\frac{2 k x}{3}$
(d) $-\frac{4 k x}{3}$
(2008, 4M)
Show Answer
Answer:
Correct Answer: 14. (d)
Solution:
$$ \begin{aligned} a & =R \alpha \\ \therefore \quad \frac{2 k x-f}{M} & =R \frac{f R}{\frac{1}{2} M R^{2}} \end{aligned} $$
Solving this equation, we get
$$ \begin{aligned} f & =\frac{2 k x}{3} \\ \therefore \quad\left|F _{\text {net }}\right| & =2 k x-f=2 k x-\frac{2 k x}{3}=\frac{4 k x}{3} \end{aligned} $$
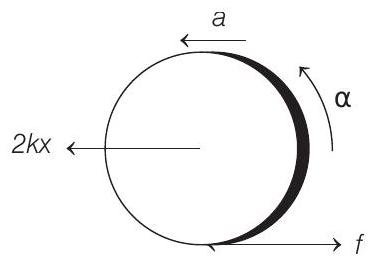
This is opposite to displacement.
$$ \therefore \quad F _{\text {net }}=-\frac{4 k x}{3} $$
