Rotation 2 Question 15
15. The torque $\tau$ on a body about a given point is found to be equal to $\mathbf{A} \times \mathbf{L}$, where $\mathbf{A}$ is a constant vector and $\mathbf{L}$ is the angular momentum of the body about that point. From this it follows that
$(1998,2$ M)
(a) $\frac{d \mathbf{L}}{d t}$ is perpendicular to $\mathbf{L}$ at all instants of time
(b) the component of $\mathbf{L}$ in the direction of $\mathbf{A}$ does not change with time
(c) the magnitude of $\mathbf{L}$ does not change with time
(d) $\mathbf{L}$ does not change with time
Passage Based Questions
Passage 1
A frame of the reference that is accelerated with respect to an inertial frame of reference is called a non-inertial frame of reference. A coordinate system fixed on a circular disc rotating about a fixed axis with a constant angular velocity $\omega$ is
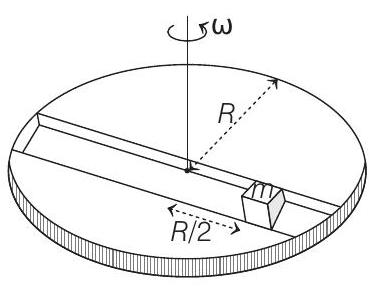an example of a non-inertial frame of reference. The relationship between the force $\mathbf{F} _{\text {rot }}$ experienced by a particle of mass $m$ moving on the rotating disc and the force $\mathbf{F} _{\text {in }}$ experienced by the particle in an inertial frame of reference is, $\mathbf{F} _{\text {rot }}=\mathbf{F} _{\text {in }}+2 m\left(\mathbf{v} _{\text {rot }} \times \vec{\omega}\right)+m(\vec{\omega} \times \mathbf{r}) \times \vec{\omega}$, where, $\mathbf{v} _{\text {rot }}$ is the velocity of the particle in the rotating frame of reference and $\mathbf{r}$ is the position vector of the particle with respect to the centre of the disc. Now, consider a smooth slot along a diameter of a disc of radius $R$ rotating counter-clockwise with a constant angular speed $\omega$ about its vertical axis through its centre. We assign a coordinate system with the origin at the centre of the disc, the $X$-axis along the slot, the $Y$-axis perpendicular to the slot and the $z$-axis along th rotation axis $(\omega=\omega \mathbf{k})$. A small block of mass $m$ is gently placed in the slot at $\mathbf{r}=(R / 2) \hat{\mathbf{i}}$ at $t=0$ and is constrained to move only along the slot.
(2016 Adv.)
Show Answer
Answer:
Correct Answer: 15. $(a, b, c)$
Solution:
- (a) $\tau=\mathbf{A} \times \mathbf{L}$
i.e. $\quad \frac{d \mathbf{L}}{d t}=\mathbf{A} \times \mathbf{L}$
This relation implies that $\frac{d \mathbf{L}}{d t}$ is perpendicular to both $\mathbf{A}$ and $\mathbf{L}$.
(c) Here, $\mathbf{L} \cdot \mathbf{L}=L^{2}$
Differentiating w.r.t. time, we get
$$ \begin{aligned} \Rightarrow & \mathbf{L} \cdot \frac{d \mathbf{L}}{d t}+\frac{d \mathbf{L}}{d t} \cdot \mathbf{L} & =2 L \frac{d L}{d t} \\ & 2 \mathbf{L} \cdot \frac{d \mathbf{L}}{d t} & =2 L \frac{d L}{d t} \end{aligned} $$
But since,
$$ \mathbf{L} \perp \frac{d \mathbf{L}}{d t} $$
$$ \therefore \quad \mathbf{L} \cdot \frac{d \mathbf{L}}{d t}=0 $$
Therefore, from Eq. (i) $\quad \frac{d L}{d t}=0$
or magnitude of $\mathbf{L}$ i.e. $L$ does not change with time.
(b) So far we are confirm about two points
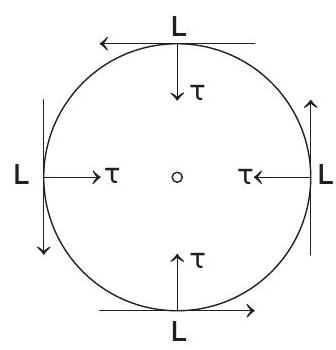
(i) $\operatorname{Tor} \frac{d \mathbf{L}}{d t} \perp \mathbf{L}$ and
(ii) $|\mathbf{L}|=L$ is not changing with time, therefore, it is a case when direction of $\mathbf{L}$ is changing but its magnitude is constant and $\tau$ is perpendicular to $\mathbf{L}$ at all points.
This can be written as
If $\quad \mathbf{L}=(a \cos \theta) \hat{\mathbf{i}}+(a \sin \theta) \hat{\mathbf{j}}$
Here, $a=$ positive constant
Then $\tau=(a \sin \theta) \hat{\mathbf{i}}-(a \cos \theta) \hat{\mathbf{j}}$
So, that $\mathbf{L} \cdot \tau=0$ and $\mathbf{L} \perp \tau$
Now, $\mathbf{A}$ is constant vector and it is always perpendicular to $\tau$. Thus, $\mathbf{A}$ can be written as $\mathbf{A}=A \hat{\mathbf{k}}$ we can see that $\mathbf{L} \cdot \mathbf{A}=0$ i.e. $\mathbf{L} \perp \mathbf{A}$ also.
Thus, we can say that component of $\mathbf{L}$ along $\mathbf{A}$ is zero or component of $\mathbf{L}$ along $\mathbf{A}$ is always constant.
Finally, we conclude that $\tau \mathbf{A}$ and $\mathbf{L}$ are always mutually perpendicular.
