Optics 6 Question 7
8. An astronomical telescope has an angular magnification of magnitude 5 for far objects. The separation between the objective and the eyepiece is $36 cm$ and the final image is formed at infinity. The focal length $f _o$ of the objective and the focal length $f _e$ of the eyepiece are
(1989, 2M)
(a) $f _o=45 cm$ and $f _e=-9 cm$
(b) $f _o=50 cm$ and $f _e=10 cm$
(c) $f _o=7.2 cm$ and $f _e=5 cm$
(d) $f _o=30 cm$ and $f _e=6 cm$
Show Answer
Answer:
Correct Answer: 8. (c)
Solution:
- In the given case, figure for first minima will be as shown below
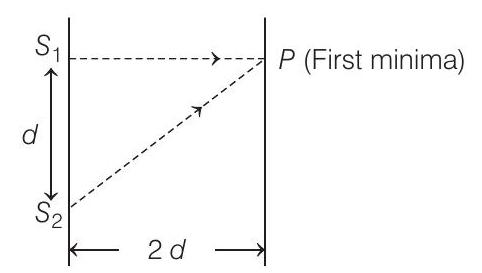
We know that condition for minima in Young’s double slit experiment is path difference,
$$ \begin{aligned} & \text { For first minima, } n=1 \\ & \Rightarrow \quad \Delta x=\lambda / 2 \cdots(i) \end{aligned} $$
Path difference between the rays coming from virtual sources $S _1$ and $S _2$ at point ’ $P$ ’ will be
$$ \Delta x=S _2 P-S _1 P \cdots(ii) $$
From triangle $S _1 S _2 P$,
$$ S _1 P=2 d \cdots(iii) $$
and $\quad\left(S _2 P^{2}\right)=\left(S _1 S _2\right)^{2}+\left(S _1 P\right)^{2}=d^{2}+(2 d)^{2}$
$\Rightarrow \quad\left(S _2 P^{2}\right)=5 d^{2}$ or $S _2 P=\sqrt{5} d \cdots(iv)$
Substituting the values from Eqs. (iii) and (iv) in Eq. (ii), we get
$$ \Delta x=\sqrt{5} d-2 d \cdots(v) $$
From Eqs. (i) and (v), we get
$$ \sqrt{5} d-2 d=\lambda / 2 \Rightarrow d=\frac{\lambda}{2(\sqrt{5}-2)} $$






