Optics 6 Question 4
5. In an interference experiment, the ratio of amplitudes of coherent waves is
(a) 2
(b) 18
(c) 4
(d) 9
(2019 Main, 8 April I)
Show Answer
Answer:
Correct Answer: 5. (c)
Solution:
- In Young’s double slit experiment, ratio of maxima and minima intensity is given by
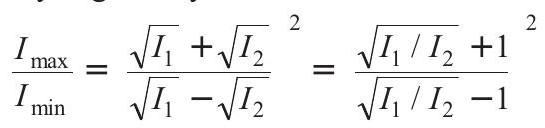
As, intensity
So,






