Optics 4 Question 5
5. Monochromatic light is incident on a glass prism of angle $A$. If the refractive index of the material of the prism is $\mu$, a ray incident at an angle $\theta$, on the face $A B$ would get transmitted through the face $A C$ of the prism provided
(2015 Main)
(a) $\theta<\cos ^{-1} \Big[\mu \sin \Big(A+\sin ^{-1} \Big(\frac{1}{\mu}\Big) \Big)\Big]$
(b) $\theta<\sin ^{-1} \Big[\mu \sin \Big(A-\sin ^{-1} \Big(\frac{1}{\mu}\Big) \Big)\Big]$
(c) $\theta>\cos ^{-1} \Big[\mu \sin \Big(A+\sin ^{-1} \Big(\frac{1}{\mu}\Big) \Big)\Big]$
(d) $\theta>\sin ^{-1} \Big[\mu \sin \Big(A-\sin ^{-1} \Big(\frac{1}{\mu}\Big) \Big)\Big]$
Show Answer
Answer:
Correct Answer: 5. (d)
Solution:
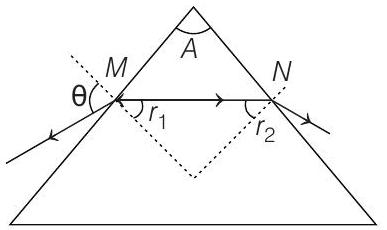
Applying Snell’s law at $M$,
$$ \begin{gathered} \mu=\frac{\sin \theta}{\sin r _1} \\ \therefore \quad r _1=\sin ^{-1} \Big(\frac{\sin \theta}{\mu} \text { or } \sin r _1=\frac{\sin \theta}{\mu}\Big) \end{gathered} $$
Now, $\quad r _2=A-r _1=A-\sin ^{-1} \Big(\frac{\sin \theta}{\mu}\Big)$
Ray of light would get transmitted form face $A C$ if
$$ r _2<\theta _c \quad \text { or } \quad A-\sin ^{-1} \Big(\frac{\sin \theta}{\mu}\Big)<\theta _c $$
where,
$$ \theta _c=\sin ^{-1} \Big(\frac{1}{\mu}\Big) $$
$\therefore \quad \sin ^{-1} \Big(\frac{\sin \theta}{\mu}>A-\theta _c\Big)$
$$ \begin{array}{rlrl} \text { or } & & \Big(\frac{\sin \theta}{\mu}\Big)>\sin \left(A-\theta _c\right) \\ & \therefore & \theta>\sin ^{-1}\left[\mu \sin \left(A-\theta _c\right)\right] \\ \text { or } & \theta>\sin ^{-1} \Big[\mu \sin \Big(A-\sin ^{-1} \Big(\frac{1}{\mu}\Big) \Big)\Big] \end{array} $$
