Optics 4 Question 1
1. A monochromatic light is incident at a certain angle on an equilateral triangular prism and suffers minimum deviation. If the refractive index of the material of the prism is $\sqrt{3}$, then the angle of incidence is
(2019 Main, 11 Jan II)
(a) $45^{\circ}$
(b) $90^{\circ}$
(c) $60^{\circ}$
(d) $30^{\circ}$
Show Answer
Answer:
Correct Answer: 1. (c)
Solution:
- Given, refractive index of material of prism $n=\sqrt{3}$, prism angle $A=60^{\circ}$
Method 1
Using prism formula,
$$ \begin{array}{rlrl} n & =\frac{\sin \frac{A+\delta}{2}}{\sin \frac{A}{2}} \\ \Rightarrow \quad & \sin \frac{60+\delta}{2} & =\frac{\sqrt{3}}{2} \\ \Rightarrow \quad & \sin \frac{60+\delta}{2} & =\sin 60^{\circ} \\ \Rightarrow \quad & \frac{60+\delta}{2} & =60 \end{array} $$
or angle of minimum deviation $\delta=60^{\circ}$
Incident angle, $\quad i=\frac{60+\delta}{2}=60^{\circ}$
Method 2 For minimum deviation, ray should pass symmetrically (i.e. parallel to the base of the equilateral prism) $\Rightarrow$ From geometry of given figure, we have, $r=30^{\circ}$
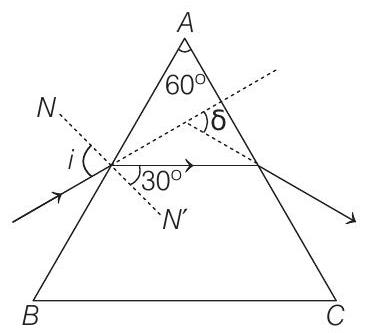
Using Snell’s law,
$$ \begin{aligned} n & =\frac{\sin i}{\sin r} \\ \sin i & =n \sin r=\sqrt{3} \sin 30^{\circ} \\ \Rightarrow \quad \sin i & =\frac{\sqrt{3}}{2} \text { or } i=60^{\circ} \end{aligned} $$






