Optics 3 Question 14
14. A bi-convex lens is formed with two thin plano-convex lenses as shown in the figure. Refractive index $n$ of the first lens is 1.5 and that of the second lens is 1.2. Both the curved surfaces are of the same radius of curvature $R=14 cm$.
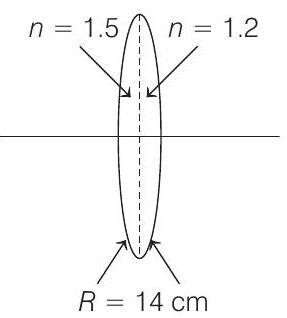
For this bi-convex lens, for an object distance of $40 cm$, the image distance will be
(2012)
(a) $-280.0 cm$
(b) $40.0 cm$
(c) $21.5 cm$
(d) $13.3 cm$
Show Answer
Answer:
Correct Answer: 14. (b)
Solution:
- Using the lens formula,
$$ \begin{aligned} \frac{1}{v}-\frac{1}{u} & =\frac{1}{F}=\frac{1}{f _1}+\frac{1}{f _2} \text { or } \frac{1}{v}=\frac{1}{u}+\frac{1}{f _1}+\frac{1}{f _2} \\ & =\frac{1}{u}+\left(n _1-1\right) \frac{1}{R _1}-\frac{1}{R _2}+\left(n _2-1\right) \frac{1}{R _1^{\prime}}-\frac{1}{R _2^{\prime}} \end{aligned} $$
Substituting the values, we get
$$ \frac{1}{v}=\frac{1}{-40}+(1.5-1) \frac{1}{14}-\frac{1}{\infty}+(1.2-1) \frac{1}{\infty}-\frac{1}{-14} $$
Solving this equations, we get
$$ v=+40 cm $$






