Optics 2 Question 2
2. A ray of light $A O$ in vacuum is incident on a glass slab at angle $60^{\circ}$ and refracted at angle $30^{\circ}$ along $O B$ as shown in the figure. The optical path length of light ray from $A$ to $B$ is
(2019 Main, 11 April I)
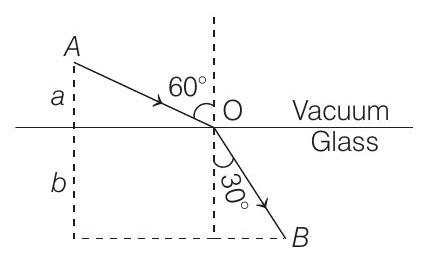
(a) $\frac{2 \sqrt{3}}{a}+2 b$
(b) $2 a+\frac{2 b}{3}$
(c) $2 a+2 b$
(d) $2 a+\frac{2 b}{\sqrt{3}}$
Show Answer
Answer:
Correct Answer: 2. (c)
Solution:
- From the figure,
$$ \begin{array}{rlrl} & & \cos 60^{\circ} & =\frac{a}{A O} \\ \Rightarrow & A O & =\frac{a}{\cos 60^{\circ}}=2 a \\ \text { and } & & \cos 30^{\circ} & =\frac{b}{B O} \\ \text { or } & B O & =\frac{b}{\cos 30^{\circ}}=\frac{2}{\sqrt{3}} b \end{array} $$
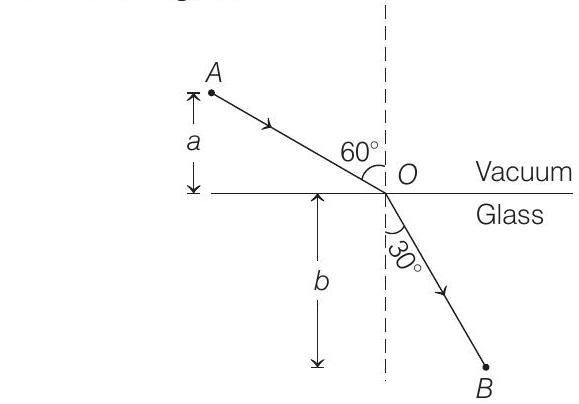
Optical path length of light ray
$$ =A O+\mu(B O) $$
Here, $\mu$ can be determined using Snell’s law, i.e.
$$ \mu=\frac{\sin 60^{\circ}}{\sin 30^{\circ}}=\frac{\sqrt{3} / 2}{1 / 2}=\sqrt{3} $$
Substituting the values from Eqs. (i), (ii) and (iv) in Eq. (iii), we get
$\therefore \quad$ Optical path $=2 a+\left(\sqrt{3} \times \frac{2}{\sqrt{3}} b\right)$
$$ =2 a+2 b $$
