Modern Physics 7 Question 44
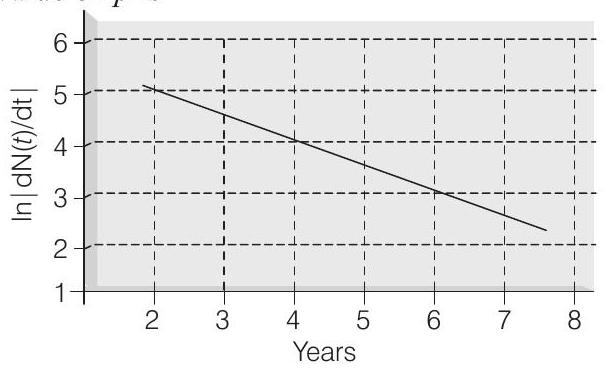
49. To determine the half-life of a radioactive element, a student plots a graph of $\ln \left|\frac{d N(t)}{d t}\right|$ versus $t$. Here $\frac{d N(t)}{d t}$ is the rate of radioactive decay at time $t$. If the number of radioactive nuclei of this element decreases by a factor of $p$ after $4.16 yr$, the value of $p$ is
(2010)
Fill in the Blank
Show Answer
Answer:
Correct Answer: 49. $8 \quad 50$. Atomic number, mass number
Solution:
- $\frac{d N}{d t}=\mid$ Activity of radioactive substance $\mid$
$$ =\lambda N=\lambda N _0 e^{-\lambda t} $$
Taking $\log$ both sides
$\ln \frac{d N}{d t}=\ln \left(\lambda N _0\right)-\lambda t$
Hence, $\ln \frac{d N}{d t}$ versus $t$ graph is a straight line with slope $-\lambda$.
From the graph we can see that, $\lambda=\frac{1}{2}=0.5 yr^{-1}$
Now applying the equation,
$$ \begin{aligned} N & =N _0 e^{-\lambda t}=N _0 e^{-0.5 \times 4.16} \\ & =N _0 e^{-2.08}=0.125 N _0=\frac{N _0}{8} \end{aligned} $$
i.e, nuclei decreases by a factor of 8 .
Hence, the answer is 8.






