Magnetics 4 Question 11
12. When $d \approx a$ but wires are not touching the loop, it is found that the net magnetic field on the axis of the loop is zero at a height $h$ above the loop. In that case
(a) current in wire 1 and wire 2 is the direction $P Q$ and $R S$, respectively and $h \approx a$ (b) current in wire 1 and wire 2 is the direction $P Q$ and $S R$, respectively and $h \approx a$
(c) current in wire 1 and wire 2 is the direction $P Q$ and $S R$, respectively and $h \approx 1.2 a$
(d) current in wire 1 and wire 2 is the direction $P Q$ and $R S$, respectively and $h \approx 1.2 a$
Show Answer
Answer:
Correct Answer: 12. (c)
Solution:
- $\mathbf{B} _R=\mathbf{B}$ due to ring
$\mathbf{B} _1=\mathbf{B}$ due to wire-1
$\Rightarrow \quad \mathbf{B} _2=\mathbf{B}$ due to wire-2
In magnitudes,
$$ \mathbf{B} _1=\mathbf{B} _2=\frac{\mu _0 I}{2 \pi r} $$
Resultant of $\mathbf{B} _1$ and $\mathbf{B} _2$
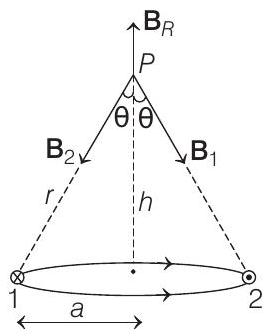
$=2 \mathbf{B} _1 \cos \theta=2 \frac{\mu _0 I}{2 \pi r} \quad \frac{h}{r}=\frac{\mu _0 I h}{\pi r^{2}}$
$$ \begin{aligned} \mathbf{B} _R & =\frac{\mu _0 I R^{2}}{2\left(R^{2}+x^{2}\right)^{3 / 2}}=\frac{2 \mu _0 I \pi a^{2}}{4 \pi r^{3}} \\ \text { As, } \quad R & =a, x=h \quad \text { and } \quad a^{2}+h^{2}=r^{2} \end{aligned} $$
For zero magnetic field at $P, \frac{\mu _0 I h}{\pi r^{2}}=\frac{2 \mu _0 I \pi a^{2}}{4 \pi r^{3}}$
$$ \Rightarrow \quad \pi a^{2}=2 r h \quad \Rightarrow h \approx 1.2 a $$






