Magnetics 3 Question 13
13. Two long straight parallel wires are $2 m$ apart, perpendicular to the plane of the paper.
The wire $A$ carries a current of $9.6 A$, directed into the plane of the paper. The wire $B$ carries a current such that the magnetic field of induction at the point $P$, at a distance of $10 / 11 m$ from the wire $B$, is zero.
$(1987,7 M)$ Find
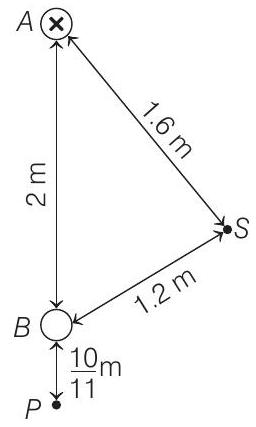
(a) the magnitude and direction of the current in $B$.
(b) the magnitude of the magnetic field of induction at the point $S$.
(c) the force per unit length on the wire $B$.
Show Answer
Solution:
- (a) Direction of current at $B$ should be perpendicular to paper outwards. Let current in this wire be $i _B$. Then,
$$ \frac{\mu _0}{2 \pi} \frac{i _A}{2+\frac{10}{11}}=\frac{\mu _0}{2 \pi} \frac{i _B}{(10 / 11)} $$
or $\quad \frac{i _B}{i _A}=\frac{10}{32}$
or $\quad i _B=\frac{10}{32} \times i _A=\frac{10}{32} \times 9.6=3 A$
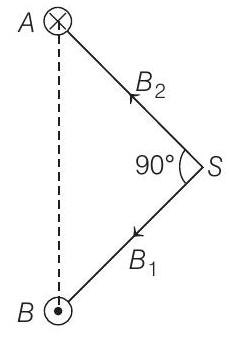
(b) Since, $A S^{2}+B S^{2}=A B^{2}$
$$ \therefore \quad \angle A S B=90^{\circ} $$
At $S: B _1=$ Magnetic field due to $i _A$
$$ \begin{aligned} & =\frac{\mu _0}{2 \pi} \frac{i _A}{1.6} \\ & =\frac{\left(2 \times 10^{-7}\right)(9.6)}{1.6}=12 \times 10^{-7} T \end{aligned} $$
$B _2=$ Magnetic field due to $i _B$
$$ =\frac{\mu _0}{2 \pi} \frac{i _B}{1.2}=\frac{\left(2 \times 10^{-7}\right)(3)}{1.2}=5 \times 10^{-7} T $$
Since, $B _1$ and $B _2$ are mutually perpendicular. Net magnetic field at $S$ would be
$$ \begin{aligned} B & =\sqrt{B _1^{2}+B _2^{2}} \\ & =\sqrt{\left(12 \times 10^{-7}\right)^{2}+\left(5 \times 10^{-7}\right)^{2}} \\ & =13 \times 10^{-7} T \end{aligned} $$
(c) Force per unit length on wire $B$
$$ \begin{aligned} \frac{F}{l} & =\frac{\mu _0}{2 \pi} \frac{i _A i _B}{r} \quad \quad \quad \quad \quad(r=A B=2 m) \\ & =\frac{\left(2 \times 10^{-7}\right)(9.6 \times 3)}{2}=2.88 \times 10^{-6} N / m \end{aligned} $$






