Magnetics 2 Question 13
13. A non-planar loop of conducting wire carrying a current $I$ is placed as shown in the figure. Each of the straight sections of the loop is of length $2 a$. The magnetic field due to this loop at the point $P(a, 0, a)$ points in the direction
(2001, 2M)
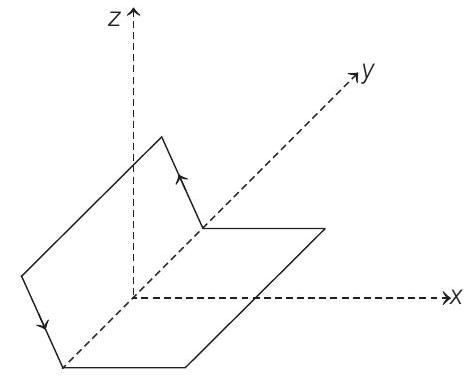
(a) $\frac{1}{\sqrt{2}}(-\hat{\mathbf{j}}+\hat{\mathbf{k}})$
(b) $\frac{1}{\sqrt{3}}(-\hat{\mathbf{j}}+\hat{\mathbf{k}}+\hat{\mathbf{i}})$
(c) $\frac{1}{\sqrt{3}}(\hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}})$
(d) $\frac{1}{\sqrt{2}}(\hat{\mathbf{i}}+\hat{\mathbf{k}})$
Show Answer
Answer:
Correct Answer: 13. (d)
Solution:
- The magnetic field at $P(a, 0, a)$ due to the loop is equal to the vector sum of the magnetic fields produced by loops $A B C D A$ and $A F E B A$ as shown in the figure.
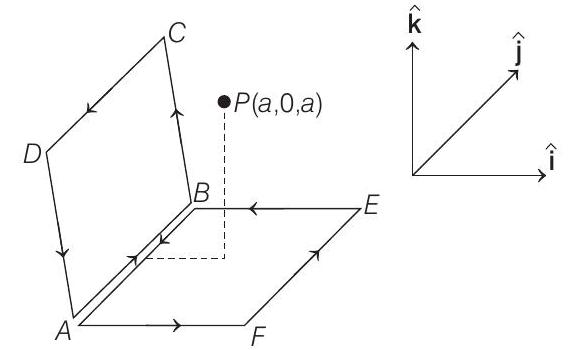
Magnetic field due to loop $A B C D A$ will be along $\hat{\mathbf{i}}$ and due to loop $A F E B A$, along $\hat{\mathbf{k}}$. Magnitude of magnetic field due to both the loops will be equal. Therefore, direction of resultant magnetic field at $P$ will be $\frac{1}{\sqrt{2}}(\hat{\mathbf{i}}+\hat{\mathbf{k}})$.
NOTE This is a common practice, when by assuming equal currents in opposite directions in an imaginary wire (here AB) loops are completed and solution becomes easy.






