SATHEE: Magnetics 2 Question 1

Magnetics 2 Question 1

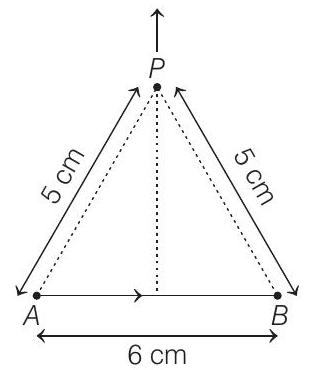
1. Find the magnetic field at point $P$ due to a straight line segment $A B$ of length $6 c m$ carrying a current of $5 A$ (See figure). (Take, $μ_{0} = 4 π \times 10^{- 7} N - A^{- 2}$ )

(2019 Main, 12 April II)

(a) $2.0 \times 10^{- 5} T$

(b) $1.5 \times 10^{- 5} T$

(d) $2.5 \times 10^{- 5} T$

Show Answer

Answer:

Correct Answer: 1. (b)

Solution:

The given figure can be drawn as shown below

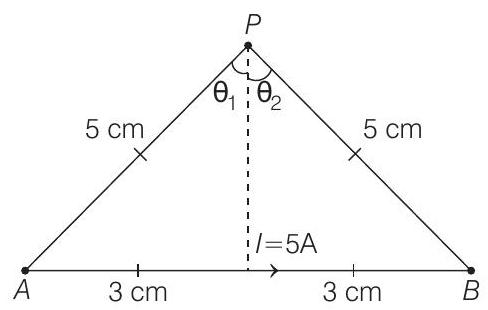
By Biot-Savart’s law, magnetic field due to a wire segment at point $P$ is

$B = \frac{μ_{0} I}{4 π d} (\sin θ_{1} + \sin θ_{2})$

Here,

$θ_{1} = θ_{2} = θ$

Then,

$B = \frac{μ_{0} I}{4 π d} \times 2 \sin θ$

From given data,

$\begin{aligned} I & = 5 A, \\ μ & = 4 π \times 10^{- 7} N A^{- 2} \\ d & = \sqrt{5^{2} - 3^{2}} = \sqrt{16} = 4 c m \\ \sin θ & = \frac{3}{5} \end{aligned}$

On substituting these values in Eq. (i), we get

$\begin{aligned} B = \frac{μ_{0} I}{4 π d} \times 2 \sin θ & = \frac{4 π \times 10^{- 7} \times 5}{4 π \times 4 \times 10^{- 2}} \times 2 \times \frac{3}{5} \\ = \frac{5 \times 2 \times 3}{4 \times 5} \times 10^{- 5} = 1.5 \times 10^{- 5} T \end{aligned}$

Magnetics 2 Question 1

1. Find the magnetic field at point $P$ due to a straight line segment $A B$ of length $6 c m$ carrying a current of $5 A$ (See figure). (Take, $μ_{0} = 4 π \times 10^{- 7} N - A^{- 2}$ )

Answer:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Magnetics 2 Question 1

1. Find the magnetic field at point P due to a straight line segment AB of length 6cm carrying a current of 5A (See figure). (Take, μ0=4π×10−7N−A−2 )

Answer:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu

1. Find the magnetic field at point $P$ due to a straight line segment $A B$ of length $6 c m$ carrying a current of $5 A$ (See figure). (Take, $μ_{0} = 4 π \times 10^{- 7} N - A^{- 2}$ )