Laws of Motion 3 Question 25
27. A block is moving on an inclined plane making an angle $45^{\circ}$ with the horizontal and the coefficient of friction is $\mu$.
The force required to just push it up the inclined plane is 3 times the force required to just prevent it from sliding down.If we define $N=10 \mu$, then $N$ is
(2011)
Show Answer
Answer:
Correct Answer: 27. 5
Solution:
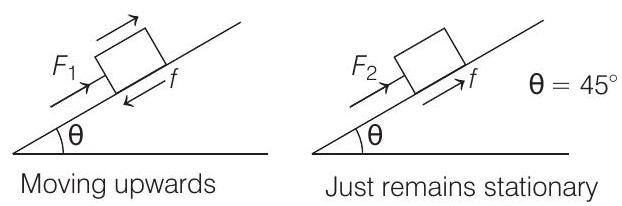
$F _1=m g \sin \theta+\mu m g \cos \theta$
$$ \begin{aligned} F _2 & =m g \sin \theta-\mu m g \cos \theta \\ \text { Given that } F _1 & =3 F _2 \text { or }\left(\sin 45^{\circ}+\mu \cos 45^{\circ}\right) \\ & =3\left(\sin 45^{\circ}-\mu \cos 45^{\circ}\right) \end{aligned} $$
On solving, we get $\mu=0.5 \therefore \quad N=10 \mu=5$






