Laws of Motion 3 Question 18
19. Two blocks $A$ and $B$ of equal masses are released from an inclined plane of inclination $45^{\circ}$ at $t=0$. Both the blocks are initially at rest. The coefficient of kinetic
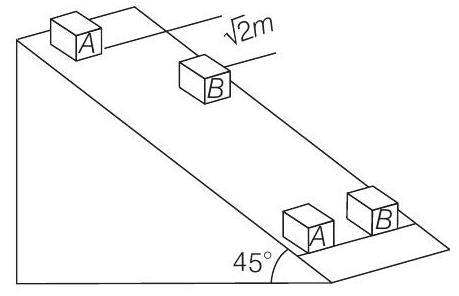friction between the block $A$ and the inclined plane is 0.2 while it is 0.3 for block $B$. Initially the block $A$ is $\sqrt{2} m$ behind the block $B$. When and where their front faces will come in a line?
(Take $g=10 m / s^{2}$ )
(2004, 5M)
Show Answer
Answer:
Correct Answer: 19. $s _A=8 \sqrt{2} m, 2 s$
Solution:
- Acceleration of $A$ down the plane,
$$ \begin{aligned} a _A & =g \sin 45^{\circ}-\mu _A g \cos 45^{\circ} \\ & =(10) \frac{1}{\sqrt{2}}-(0.2)(10) \frac{1}{\sqrt{2}}=4 \sqrt{2} m / s^{2} \end{aligned} $$
Similarly acceleration of $B$ down the plane,
$$ \begin{aligned} a _B & =g \sin 45^{\circ}-\mu _B g \cos 45^{\circ} \\ & =(10) \frac{1}{\sqrt{2}}-(0.3)(10) \frac{1}{\sqrt{2}}=3.5 \sqrt{2} m / s^{2} \end{aligned} $$
The front face of $A$ and $B$ will come in a line when,
$$ \text { or } \begin{aligned} s _A & =s _B+\sqrt{2} \\ \frac{1}{2} a _A t^{2} & =\frac{1}{2} a _B t^{2}+\sqrt{2} \\ \frac{1}{2} \times 4 \sqrt{2} \times t^{2} & =\frac{1}{2} \times 3.5 \sqrt{2} \times t^{2}+\sqrt{2} \end{aligned} $$
Solving this equation, we get $t=2 s$
Further, $\quad s _A=\frac{1}{2} a _A t^{2}=\frac{1}{2} \times 4 \sqrt{2} \times(2)^{2}=8 \sqrt{2} m$
Hence, both the blocks will come in a line after $A$ has travelled a distance $8 \sqrt{2} m$ down the plane.
