Kinematics 5 Question 20
19. Particles $P$ and $Q$ of mass $20 g$ and $40 g$ respectively are simultaneously projected from points $A$ and $B$ on the ground. The initial velocities of $P$ and $Q$ make $45^{\circ}$ and $135^{\circ}$ angles respectively with the horizontal $A B$ as shown in the figure. Each particle has an initial speed of $49 m / s$. The separation $A B$ is $245 m$.
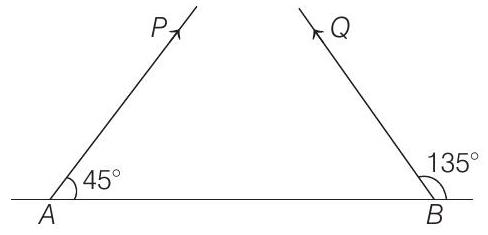
Both particles travel in the same vertical plane and undergo a collision. After the collision, $P$ retraces its path. (a) Determine the position $Q$ where it hits the ground. (b) How much time after the collision does the particle $Q$ take to reach the ground? (Take $g=9.8 m / s^{2}$ ).
$(1982,8 M$
Show Answer
Answer:
Correct Answer: 19. Just midway between $A$ and $B, 3.53 s$
Solution:
- (a) Range of both the particles is
$$ R=\frac{u^{2} \sin 2 \theta}{g}=\frac{(49)^{2} \sin 90^{\circ}}{9.8} $$
By symmetry we can say that they will collide at highest point.

Just before collision Just after collision
Let $v$ be the velocity of $Q$ just after collision. Then, from conservation of linear momentum, we have
$$ \begin{aligned} 20\left(u \cos 45^{\circ}\right)-40\left(u \cos 45^{\circ}\right)= & 40(v) \\ & -20\left(u \cos 45^{\circ}\right) \\ \therefore \quad v= & 0 \end{aligned} $$
i.e. particle $Q$ comes to rest. So, particle $Q$ will fall vertically downwards and will strike just midway between $A$ and $B$.
(b) Maximum height,
$$ H=\frac{u^{2} \sin ^{2} \theta}{2 g}=\frac{(49)^{2} \sin ^{2} 45^{\circ}}{2 \times 9.8}=61.25 m $$
Therefore, time taken by $Q$ to reach the ground,
$$ t=\sqrt{\frac{2 H}{g}}=\sqrt{\frac{2 \times 61.25}{9.8}}=3.53 s $$






