Kinematics 5 Question 1
3. The trajectory of a projectile near the surface of the earth is given as $y=2 x-9 x^{2}$.
If it were launched at an angle $\theta _0$ with speed $v _0$, then (Take, $g=10 ms^{-2}$ )
(2019 Main, 12 April I)
(a) $\theta _0=\sin ^{-1} \frac{1}{\sqrt{5}}$ and $v _0=\frac{5}{3} ms^{-1}$
(b) $\theta _0=\cos ^{-1} \frac{2}{\sqrt{5}}$ and $v _0=\frac{3}{5} ms^{-1}$
(c) $\theta _0=\cos ^{-1} \frac{1}{\sqrt{5}}$ and $v _0=\frac{5}{3} ms^{-1}$
(d) $\theta _0=\sin ^{-1} \frac{2}{\sqrt{5}}$ and $v _0=\frac{3}{5} ms^{-1}$
Show Answer
Answer:
Correct Answer: 3. (c)
Solution:
- Given, $g=10 m / s^{2}$
Equation of trajectory of the projectile,
$$ y=2 x-9 x^{2} $$
In projectile motion, equation of trajectory is given by
$$ y=x \tan \theta _0-\frac{g x^{2}}{2 v _0^{2} \cos ^{2} \theta _0} $$
By comparison of Eqs. (i) and (ii), we get
$$ \begin{aligned} \tan \theta _0 & =2 \\ \text { and } \frac{g}{2 v _0^{2} \cos ^{2} \theta _0} & =9 \text { or } v _0^{2}=\frac{g}{9 \times 2 \cos ^{2} \theta _0} \end{aligned} $$
From Eq. (iii), we can get value of $\cos \theta$ and $\sin \theta$
$\cos \theta _0=\frac{1}{\sqrt{5}}$ and $\sin \theta _0=\frac{2}{\sqrt{5}}$
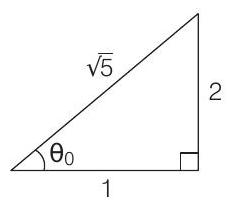
Using value of $\cos \theta _0$ from Eq. (v) to Eq. (iv), we get
$$ \begin{aligned} & v _0^{2}=\frac{10 \times(\sqrt{5})^{2}}{2 \times(1)^{2} \times 9}=\frac{10 \times 5}{2 \times 9} \\ & \Rightarrow \quad v _0^{2}=\frac{25}{9} \text { or } v _0=\frac{5}{3} m / s \end{aligned} $$
From Eq. (v), we get
$$ \theta _0=\cos ^{-1} \frac{1}{\sqrt{5}} $$






