Kinematics 4 Question 5
5. Two stones are thrown up simultaneously from the edge of a cliff $240 m$ high with initial speed of $10 m / s$ and $40 m / s$, respectively. Which of the following graph best represents the time variation of relative position of the second stone with respect to the first? (Assume stones do not rebound after hitting the ground and neglect air resistance, take $g=10 m / s^{2}$ )
(2015 Main)
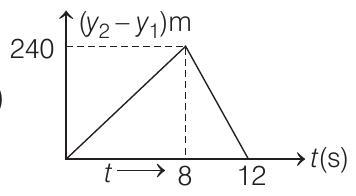
(a)
(b)
(c)
(d)
Show Answer
Answer:
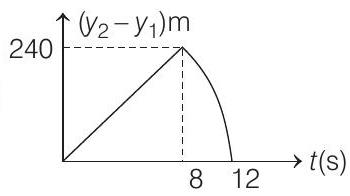
Correct Answer: 5. (b)
Solution:
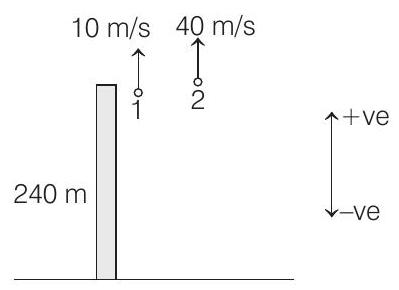
Let us first find, time of collision of two particles with ground in putting proper values in the equation
$$ \begin{aligned} S & =u t+\frac{1}{2} a t^{2} \\ -240 & =10 t _1-\frac{1}{2} \times 10 \times t _1^{2} \end{aligned} $$
Solving, we get the position value of $t _1=8 sec$
Therefore, the first particle will strike the ground at $8 sec$.
Similarly,
$$ -240=40 t _2-\frac{1}{2} \times 10 \times t _2^{2} $$
Solving this equation, we get positive value of $t _2=12 sec$ Therefore, second particle strikes the ground at $12 sec$.
If $y$ is measured from ground. Then,
from 0 to $8 sec$
or
$$ \begin{aligned} y _1 & =240+S _1 \\ & =240+u _1 t+\frac{1}{2} a _1 t^{2} \end{aligned} $$
Similarly, $\quad y _2=240+40 t-\frac{1}{2} \times 10 \times t^{2}$
$\Rightarrow \quad t _2-y _1=30 t$
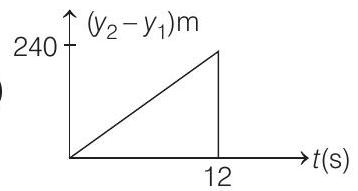
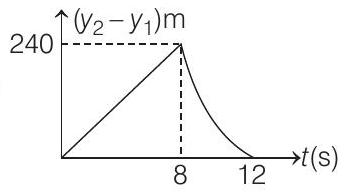
$\therefore\left(y _2-y _1\right)$ versus $t$ graph is a straight line passing through origin
At $t=8 sec, y _2-y _1=240 m$
From $8 sec$ to $12 sec$
$$ \begin{gathered} y _1=0 \\ \Rightarrow \quad y _2=240+40 t-\frac{1}{2} \times 10 \times t^{2} \\ =240+40 t-5 t \\ \therefore \quad\left(y _2-y _1\right)=240+40 t-5 t^{2} \end{gathered} $$
Therefore, $\left(y _2-y _1\right)$ versus $t$ graph is parabolic, substituting the values we can check that at $t=8 sec$, $y _2-y _1$ is $240 m$ and at $t=12 sec, y _2-y _1$ is zero.






