Kinematics 2 Question 3
5. In a car race on a straight path, car $A$ takes a time $t$ less than car $B$ at the finish and passes finishing point with a speed ’ $v$ ’ more than that of car $B$. Both the cars start from rest and travel with constant acceleration $a _1$ and $a _2$ respectively. Then ’ $v$ ’ is equal to
(2019 Main, 09 Jan II )
(a) $\frac{2 a _1 a _2}{a _1+a _2} t$
(b) $\sqrt{2 a _1 a _2} t$
(c) $\sqrt{a _1 a _2} t$
(d) $\frac{a _1+a _2}{2} t$
Show Answer
Answer:
Correct Answer: 5. (c)
Solution:
- Let car $B$ takes time $\left(t _0+t\right)$ and car $A$ takes time $t _0$ to finish the race.
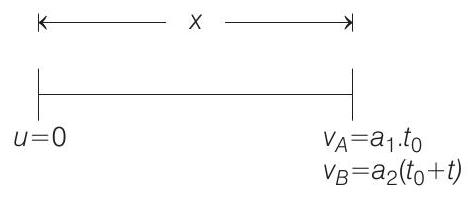
Then,
$$ \begin{aligned} & \text { Given, } \quad v _A-v _B=v=\left(a _1-a _2\right) t _0-a _2 t \\ & s _B=s _A=\frac{1}{2} a _1 t _0^{2}=\frac{1}{2} a _2\left(t _0+t\right)^{2} \\ & \text { or } \quad \sqrt{a _1} t _0=\sqrt{a _2}\left(t _0+t\right) \\ & \text { or } \quad \sqrt{a _1} t _0=\sqrt{a _2} t _0+\sqrt{a _2} t \\ & \text { or } \quad\left(\sqrt{a _1}-\sqrt{a _2}\right) t _0=\sqrt{a _2} t \\ & \text { or } \quad t _0=\frac{\sqrt{a _2} \cdot t}{\left(\sqrt{a _1}-\sqrt{a _2}\right)} \end{aligned} $$
Substituting the value of $t _0$ from Eq. (ii) into Eq. (i), we get
$$ \begin{aligned} v & =\left(a _1-a _2\right) \frac{\sqrt{a _2} t}{\sqrt{a _1}-\sqrt{a _2}}-a _2 t \\ & =\left(\sqrt{a _1}-\sqrt{a _2}\right)\left(\sqrt{a _1}+\sqrt{a _2}\right) \cdot \frac{\sqrt{a _2} t}{\left(\sqrt{a _1}-\sqrt{a _2}\right)}-a _2 t \\ \text { or } \quad v & =\left(\sqrt{a _1}+\sqrt{a _2}\right) \cdot \sqrt{a _2} t-a _2 t \\ & =\sqrt{a _1 a _2} \cdot t+a _2 t-a _2 t \\ \text { or } \quad v & =\sqrt{a _1 \cdot a _2} t \end{aligned} $$






