Gravitation 3 Question 10
13. Two satellites $S_{1}$ and $S_{2}$ revolve round a planet in coplanar circular orbits in the same sense. Their periods of revolution are $1 \mathrm{~h}$ and $8 \mathrm{~h}$, respectively. The radius of the orbit of $S_{1}$ is $10^{4} \mathrm{~km}$ when $S_{2}$ is closest to $S_{1}$. Find
$(1986,6 \mathrm{M})$
(a) the speed of $S_{2}$ relative to $S_{1}$,
(b) the angular speed of $S_{2}$ as actually observed by an astronaut in $S_{1}$.
Show Answer
Answer:
Correct Answer: 13. (a) $-\pi \times 10^4 \mathrm{~km} / \mathrm{h}$
(b) $3 \times 10^{-4} \mathrm{rad} / \mathrm{s}$
Solution:
- $T \propto r^{3 / 2}$ or $r \propto T^{2 / 3} \Rightarrow \frac{r_{2}}{r_{1}}={\frac{T_{2}}{T_{1}}}^{2 / 3}$
$$ \begin{aligned} r_{2} & ={\frac{T_{2}}{T_{1}}}^{2 / 3} \quad r_{1}=\frac{8}{1}^{2 / 3}\left(10^{4}\right) \\ & =4 \times 10^{4} \mathrm{~km} \end{aligned} $$
Now, $\quad v_{1}=\frac{2 \pi r_{1}}{T_{1}}=\frac{(2 \pi)\left(10^{4}\right)}{1}=2 \pi \times 10^{4} \mathrm{~km} / \mathrm{h}$
$v_{2}=\frac{2 \pi r_{2}}{T_{2}}=\frac{(2 \pi)\left(4 \times 10^{4}\right)}{8}=\left(\pi \times 10^{4}\right) \mathrm{km} / \mathrm{h}$
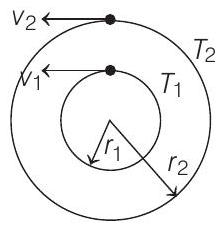
(a) Speed of $S_{2}$ relative to $S_{1}=v_{2}-v_{1}=-\pi \times 10^{4} \mathrm{~km} / \mathrm{h}$
(b) Angular speed of $S_{2}$ as observed by $S_{1}$
$$ \begin{aligned} \omega_{r} & =\frac{\left|v_{2}-v_{1}\right|}{\left|r_{2}-r_{1}\right|}=\frac{\pi \times 10^{4} \times \frac{5}{18} \mathrm{~m} / \mathrm{s}}{\left(3 \times 10^{7} \mathrm{~m}\right)} \\ & =0.3 \times 10^{-3} \mathrm{rad} / \mathrm{s} \\ & =3.0 \times 10^{-4} \mathrm{rad} / \mathrm{s} \end{aligned} $$






