Gravitation 2 Question 1
4. A rocket is launched normal to the surface of the Earth, away from the Sun, along the line joining the Sun and the Earth. The Sun is $3 \times 10^{5}$ times heavier than the Earth and is at a distance $2.5 \times 10^{4}$ times larger than the radius of Earth. The escape velocity from Earth’s gravitational field is $v _e=11.2 km s^{-1}$. The minimum initial velocity $\left(v _s\right)$ required for the rocket to be able to leave the Sun-Earth system is closest to (Ignore the rotation and revolution of the Earth and the presence of any other planet)
(a) $v _s=72 km s^{-1}$
(b) $v _s=22 km s^{-1}$
(c) $v _s=42 km s^{-1}$
(d) $v _s=62 km s^{-1}$
(2017 Adv.)
Show Answer
Answer:
Correct Answer: 4. (c)
Solution:
- Given, $v _e=11.2 km / s=\sqrt{\frac{2 G M _e}{R _e}}$
From energy conservation,
$$ \begin{array}{r} K _i+U _i=K _f+U _f \\ \frac{1}{2} m v _s^{2}-\frac{G M _s m}{r}-\frac{G M _e m}{R _e}=0+0 \end{array} $$
Here, $r=$ distane of rocket from sun
$$ \Rightarrow \quad v _s=\sqrt{\frac{2 G M _e}{R _e}+\frac{2 G M _s}{r}} $$
Given, $M _s=3 \times 10^{5} M _e$ and $r=2.5 \times 10^{4} R _e$
$$ \begin{aligned} & \Rightarrow \quad v _s=\sqrt{\frac{2 G M _e}{R _e}+\frac{2 G 3 \times 10^{5} M _e}{2.5 \times 10^{4} R _e}} \end{aligned} $$
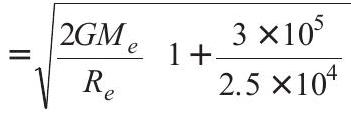
$$ \begin{aligned} & =\sqrt{\frac{2 G M _e}{R _e} \times 13} \\ & \Rightarrow \quad v _s \simeq 42 km / s \end{aligned} $$






