Electrostatics 6 Question 11
11. In the given circuit,
$(1988,5 M)$
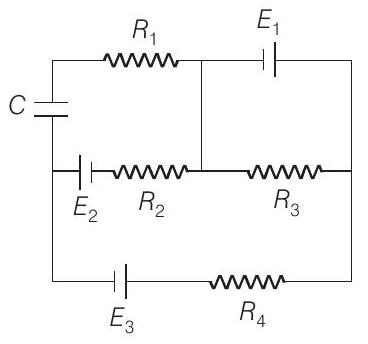
$E_{1}=3 E_{2}=2 E_{3}=6 \mathrm{~V}$ and $R_{1}=2 R_{4}=6 \Omega$,
$R_{3}=2 R_{2}=4 \Omega, C=5 \mu \mathrm{F}$.
Find the current in $R_{3}$ and the energy stored in the capacitor.
Show Answer
Answer:
Correct Answer: 11. 1.5 A from right to left$, 1.44 \times {10}^{-15} J$
Solution:
- In steady state no current will flow through $R_{1}=6 \Omega$.
Potential difference across $R_{3}$ or $4 \Omega$ is $E_{1}$ or $6 \mathrm{~V}$
$\therefore$ Current through it will be $\frac{6}{4}=1.5 \mathrm{~A}$ from right to left.
Because left hand side of this resistance is at higher potential.
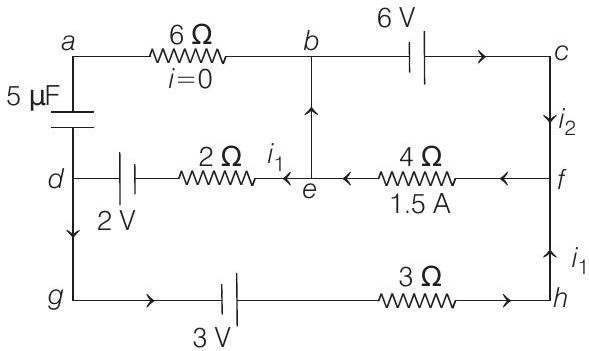
Now, suppose this $1.5 \mathrm{~A}$ distributes in $i_{1}$ and $i_{2}$ as shown.
Applying Kirchhoff’s second law in loop dghfed
$$ \begin{array}{cc} 3-3 i_{1}-4 \times 1.5-2 i_{1}+2=0 \\ \therefore & i_{1}=-\frac{1}{5} \mathrm{~A}=-0.2 \mathrm{~A} \end{array} $$
To find energy stored in capacitor we will have to find potential difference across it. Or $V_{a d}$.
Now,
$$ V_{a}-2 i_{1}+2=V_{d} $$
$$ \begin{array}{ll} \text { or } & V_{a}-V_{d}=2 i_{1}-2=-2.4 \mathrm{~V} \\ \text { or } & V_{d}-V_{a}=2.4 \mathrm{~V}=V_{d a} \end{array} $$
Energy stored in capacitor:
$$ \begin{aligned} U & =\frac{1}{2} C V_{d a}^{2} \\ & =\frac{1}{2}\left(5 \times 10^{-6}\right)(2.4)^{2} \\ & =1.44 \times 10^{-5} \mathrm{~J} \end{aligned} $$






