Electrostatics 5 Question 4
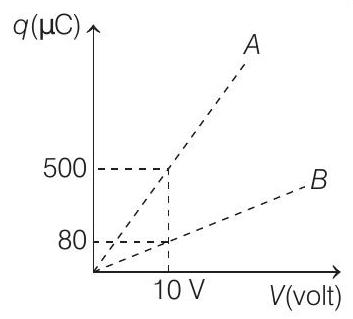
4. Figure shows charge $(q)$ versus voltage $(V)$ graph for series and parallel combination of two given capacitors. The capacitances are
(Main 2019, 10 April I)
(a) $60 \mu \mathrm{F}$ and $40 \mu \mathrm{F}$
(b) $50 \mu \mathrm{F}$ and $30 \mu \mathrm{F}$
(c) $20 \mu \mathrm{F}$ and $30 \mu \mathrm{F}$
(d) $40 \mu \mathrm{F}$ and $10 \mu \mathrm{F}$
Show Answer
Answer:
Correct Answer: 4. (d)
Solution:
- In the given figure, Slope of $O A>$ Slope of $O B$
Since, we know that, net capacitance of parallel combination $>$ net capacitance of series combination
$\therefore$ Parallel combination’s capacitance,
$ C_{P}=C_{1}+C_{2}=\frac{500 \mu \mathrm{C}}{10 \mathrm{~V}}=50 \mu \mathrm{F} \cdots(i) $
Series combination’s capacitance,
$ C_{S}=\frac{C_{1} C_{2}}{C_{1}+C_{2}}=\frac{80 \mu \mathrm{C}}{10 \mathrm{~V}}=8 \mu \mathrm{F} \cdots(ii) $
or
$ \begin{aligned} C_{1} C_{2} & =8 \times\left(C_{1}+C_{2}\right)=8 \times 50 \mu \mathrm{F} \cdots(iii)\\ & =400 \mu \mathrm{F} \quad \text { [using Eq. (i)] } \end{aligned} $
From Eqs. (i) and (iii), we get
$ \begin{aligned} & C_{1}=50-C_{2} \\ & \text { and } \quad C_{1} C_{2}=400 \\ & \Rightarrow \quad C_{2}\left(50-C_{2}\right)=400 \\ & \Rightarrow \quad 50 C_{2}-C_{2}^{2}=400 \\ & \text { or } C_{2}^{2}-50 C_{2}+400=0 \\ & \Rightarrow C_{2}=\frac{+50 \pm \sqrt{2500-1600}}{2}=\frac{+50 \pm 30}{2} \end{aligned} $
$\Rightarrow \quad C_{2}=+40 \mu \mathrm{F}$ or $+10 \mu \mathrm{F}$
Also, $C_{1}=50-C_{2} \Rightarrow C_{1}=+10 \mu \mathrm{F}$ or $+40 \mu \mathrm{F}$
Hence, capacitance of two given capacitors is $10 \mu \mathrm{F}$ and $40 \mu \mathrm{F}$.
