Electrostatics 3 Question 6
6. Consider a uniform spherical charge distribution of radius $R_{1}$ centred at the origin $O$. In this distribution, a spherical cavity of radius $R_{2}$, centred at $P$ with distance $O P=a=R_{1}-R_{2}$ (see figure) is made. If the electric field inside the cavity at position $\mathbf{r}$ is $\mathbf{E}(\mathbf{r})$, then the correct statements is/are
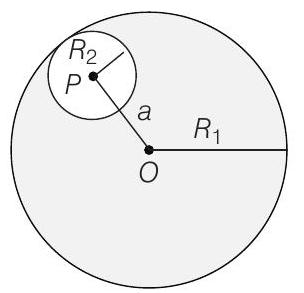
(a) $\mathbf{E}$ is uniform, its magnitude is independent of $R_{2}$ but its direction depends on $\mathbf{r}$
(b) $\mathbf{E}$ is uniform, its magnitude depends on $R_{2}$ and its direction depends on $\mathbf{r}$
(c) $\mathbf{E}$ is uniform, its magnitude is independent of ’ $a$ ’ but its direction depends on a
(d) $\mathbf{E}$ is uniform and both its magnitude and direction depend on a
Correct Answer: 6. (d) Solution: $\mathrm{E}_{+} \rightarrow \mathbf{E}$ due to total positive charge $\mathrm{E}_{-} \rightarrow \mathbf{E}$ due to total negative charge. $
\mathrm{E}=\mathrm{E}{+}+\mathrm{E}{-}
$ If we calculate it at $P$, then $\mathrm{E}_{-}$comes out to be zero. $\therefore \quad \mathrm{E}=\mathrm{E}_{+}$ and $\mathrm{E}{+}=\frac{1}{4 \pi \varepsilon{0}} \frac{q}{R_{1}^{3}}(O P)$, in the direction of $O P$. Here, $q$ is total positive charge on whole sphere. It is in the direction of $O P$ or $\mathbf{a}$. Now, inside the cavity electric field comes out to be uniform at any point. This is a standard result.Show Answer
Answer:






