Electrostatics 1 Question 17
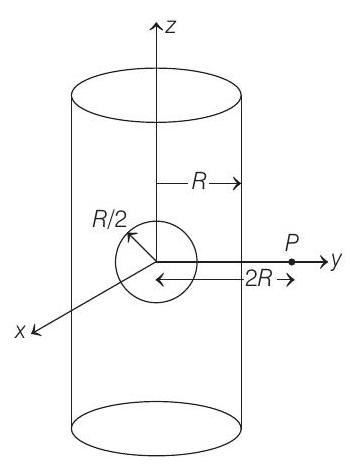
18. An infinitely long solid cylinder of radius $R$ has a uniform volume charge density $\rho$. It has a spherical cavity of radius $R / 2$ with its centre on the axis of the cylinder, as shown in the figure. The magnitude of the electric field at the point $P$, which is at a distance $2 R$ from the axis of the cylinder, is given by the expression $\frac{23 \rho R}{16 k \varepsilon_{0}}$.
The value of $k$ is
(2012)
Show Answer
Answer:
Correct Answer: 18. 6
Solution:
- Volume of cylinder per unit length $(l=1)$ is
$$ V=\pi R^{2} l=\left(\pi R^{2}\right) $$
$\therefore$ Charge per unit length,
$\lambda=($ Volume per unit length $) \times($ Volume charge density $)$
$=\left(\pi R^{2} \rho\right)$
Now at $P$
$R=$ Remaining portion
$$ E_{R}=E_{T}-E_{C} $$
$T=$ Total portion and
$C=$ cavity
$$ \begin{aligned} \therefore \quad E_{R} & =\frac{\lambda}{2 \pi \varepsilon_{0}(2 R)}-\frac{1}{4 \pi \varepsilon_{0}} \frac{Q}{(2 R)^{2}} \\ Q & =\text { charge on sphere } \\ & =\frac{4}{3} \pi \quad \frac{R^{3}}{2} \quad \rho=\frac{\pi R^{3} \rho}{6} \end{aligned} $$
Substituting the values, we have
$$ \begin{aligned} E_{R} & =\frac{\left(\pi R^{2} \rho\right)}{4 \pi \varepsilon_{0} R}-\frac{1}{4 \pi \varepsilon_{0}} \cdot \frac{\left(\pi R^{3} \rho / 6\right)}{4 R^{2}} \\ & =\frac{23 \rho R}{96 \varepsilon_{0}}=\frac{23 \rho R}{(16)(6) \varepsilon_{0}} \\ \therefore \quad k & =6 \end{aligned} $$






