Electromagnetic Induction and Alternating Current 7 Question 28
31. An infinitesimally small bar magnet of dipole moment $\mathbf{M}$ is pointing and moving with the speed $v$ in the positive $x$-direction. A small closed circular conducting loop of radius $a$ and negligible self-inductance lies in the $y$-z plane with its centre at $x=0$, and its axis coinciding with the $X$-axis. Find the force opposing the motion of the magnet, if the resistance of the loop is $R$. Assume that the distance $x$ of the magnet from the centre of the loop is much greater than $a$.
(1997C, 5M)
Show Answer
Answer:
Correct Answer: 31. $F=\frac{21}{4} \frac{\mu _0^{2} M^{2} a^{4} v}{R x^{8}}$ (repulsion)
Solution:
- Given that $x»a$.
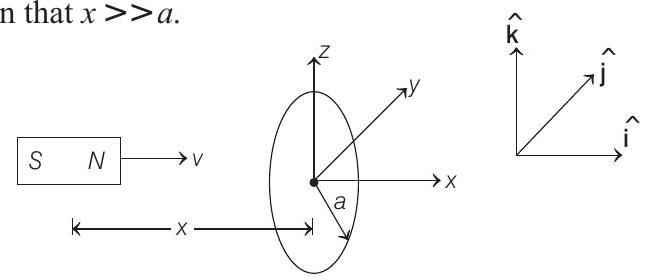
Magnetic field at the centre of the coil due to the bar magnet is
$$ B=\frac{\mu _0}{4 \pi} \frac{2 M}{x^{3}}=\frac{\mu _0}{2 \pi} \frac{M}{x^{3}} $$
Due to this, magnetic flux linked with the coil will be,
$$ \varphi=B S=\frac{\mu _0}{2 \pi} \frac{M}{x^{3}}\left(\pi a^{2}\right)=\frac{\mu _0 M a^{2}}{2 x^{3}} $$
$\therefore$ Induced emf in the coil, due to motion of the magnet is
$$ \begin{aligned} e & =\frac{-d \varphi}{d t}=-\frac{\mu _0 M a^{2}}{2} \frac{d}{d t} \frac{1}{x^{3}} \\ & =\frac{\mu _0 M a^{2}}{2} \frac{3}{x^{4}} \frac{d x}{d t}=\frac{3}{2} \frac{\mu _0 M a^{2}}{x^{4}} v \quad \because \frac{d x}{d t}=v \end{aligned} $$
Therefore, induced current in the coil is
$$ i=\frac{e}{R}=\frac{3}{2} \frac{\mu _0 M a^{2}}{R x^{4}} v $$
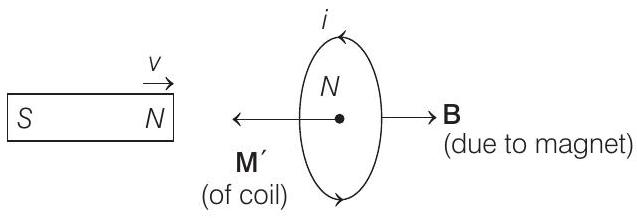
Magnetic moment of the coil due to this induced current will be,
$$ M^{\prime}=i S=\frac{3}{2} \frac{\mu _0 M a^{2}}{R x^{4}} v\left(\pi a^{2}\right) \Rightarrow M^{\prime}=\frac{3}{2} \frac{\mu _0 \pi M a^{4} v}{R x^{4}} $$
Potential energy of $\mathbf{M}^{\prime}$ in $\mathbf{B}$ will be
$$ \begin{aligned} U & =-M^{\prime} B \cos 180^{\circ} \\ U & =M^{\prime} B=\frac{3}{2} \frac{\mu _0 \pi M a^{4} v}{R x^{4}} \frac{\mu _0}{2 \pi} \cdot \frac{M}{x^{3}} \end{aligned} $$
$$ U=\frac{3}{4} \frac{\mu _0^{2} M^{2} a^{4} v}{R} \frac{1}{x^{7}} \Rightarrow F=-\frac{d U}{d x}=\frac{21}{4} \frac{\mu _0^{2} M^{2} a^{4} v}{R x^{8}} $$
Positive sign of $F$ implies that there will be a repulsion between the magnet and the coil.






