Electromagnetic Induction and Alternating Current 2 Question 11
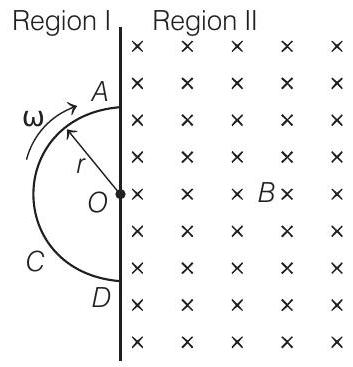
12. Space is divided by the line $A D$ into two regions. Region $I$ is field free and the region II has a uniform magnetic field $B$ directed into the plane of the paper. $A C D$ is a semicircular conducting loop of radius $r$ with centre at $O$, the plane of the loop being in the plane of the paper.
The loop is now made to rotate with a constant angular velocity $\omega$ about an axis passing through $O$ and perpendicular to the plane of the paper. The effective resistance of the loop is $R$.
$(1985,6 M)$
(a) Obtain an expression for the magnitude of the induced current in the loop.
(b) Show the direction of the current when the loop is entering into the region II.
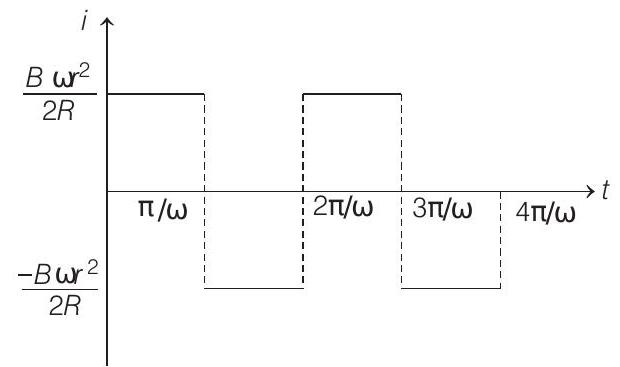
(c) Plot a graph between the induced current and the time of rotation for two periods of rotation.
Show Answer
Answer:
Correct Answer: 12. (a) $\frac{1}{2} \frac{B r^{2} \omega}{R}$ (b) anti-clockwise (c) see the solution
Solution:
- (a) At time $t: \theta=\omega t$
$\therefore$ Flux passing through $\operatorname{coil} \varphi=B S \cos 0^{\circ}$
$$ \begin{aligned} \text { or } & \varphi & =B \frac{\theta}{2 \pi}\left(\pi r^{2}\right) \\ \text { or } & \varphi & =\frac{B r^{2}}{2} \quad \theta=\frac{B r^{2}}{2} \omega t \end{aligned} $$
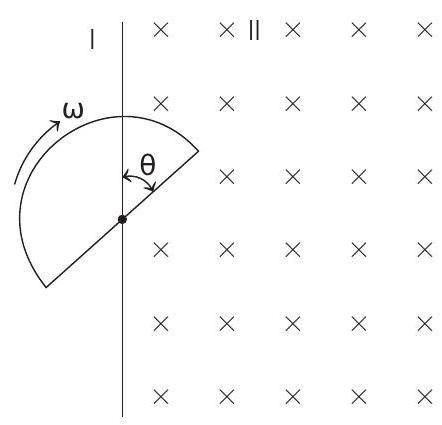
Magnitude of induced emf
$$ e=\frac{d \varphi}{d t}=\frac{B \omega r^{2}}{2} $$
$\therefore \quad$ Magnitude of induced current
$$ i=\frac{e}{R}=\frac{B \omega r^{2}}{2 R} $$
(b) When the loop enters in region II, magnetic field in cross direction passing through the loop is increasing. Hence, from the Lenz’s law, induced current will produce magnetic field in dot direction or the current will be anti-clockwise.
(c) For half rotation $t=\frac{T}{2}=\frac{\pi}{\omega}$, current in the loop will be of constant magnitude $i=\frac{B \omega r^{2}}{2 R}$ and anti-clockwise. In the next half rotation when loop comes out of region II current will be clockwise, but again magnitude is constant. So, taking anti-clockwise current as the positive, $i-t$ graph for two rotations will be as under.
