SATHEE: Centre of Mass 4 Question 3

Centre of Mass 4 Question 3

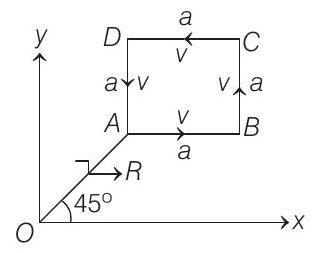
4. A particle of mass $m$ is moving along the side of a square of side $a$ , with a uniform speed $v$ in the $x y$ -plane as shown in the figure.

(2016 Main)

Which of the following statements is false for the angular momentum $L$ about the origin?

(a) $L = \frac{- m v}{\sqrt{2}} R \hat{k}$ when the particle is moving from $A$ to $B$

(b) $L = m v \frac{R}{\sqrt{2}} - a \hat{k}$ when the particle is moving from

$C$ to $D$

to $C$

(d) $L = \frac{m v}{\sqrt{2}} R \hat{k}$ when the particle is moving from $D$ to $A$

Numerical Value

Show Answer

Answer:

Correct Answer: 4. (b, d)

Solution:

We can apply $L = m (r \times v)$ for different parts.

For example :

In part (a), coordinates of $A$ are $\frac{R}{\sqrt{2}}, \frac{R}{\sqrt{2}}$ Therefore, $r = \frac{R}{\sqrt{2}} \hat{i} + \frac{R}{\sqrt{2}} \hat{j}$ and $v = v \hat{i}$

So, substituting in $L = m (r \times v)$ we get,

$L = - \frac{m v R}{\sqrt{2}} \hat{k}$

Hence, option (a) is correct. Similarly, we can check other options also.

Centre of Mass 4 Question 3

4. A particle of mass $m$ is moving along the side of a square of side $a$ , with a uniform speed $v$ in the $x y$ -plane as shown in the figure.

Numerical Value

Answer:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Centre of Mass 4 Question 3

4. A particle of mass m is moving along the side of a square of side a, with a uniform speed v in the xy-plane as shown in the figure.

Numerical Value

Answer:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu

4. A particle of mass $m$ is moving along the side of a square of side $a$ , with a uniform speed $v$ in the $x y$ -plane as shown in the figure.