Centre of Mass 1 Question 14
14. A small sphere of radius $R$ is held against the inner surface of a larger sphere of radius $6 R$. The masses of large and small spheres are $4 M$ and $M$ respectively. This arrangement is placed on a horizontal
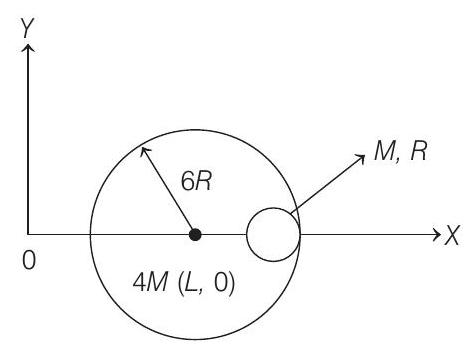
friction between any surfaces of contact. The small sphere is now released. Find the coordinates of the centre of the larger sphere when the smaller sphere reaches the other extreme position.
(1996, 3 M)
Show Answer
Answer:
Correct Answer: 14. $(L+2 R, 0)$
Solution:
- Since, all the surfaces are smooth, no external force is acting on the system in horizontal direction. Therefore, the centre of mass of the system in horizontal direction remains stationary.
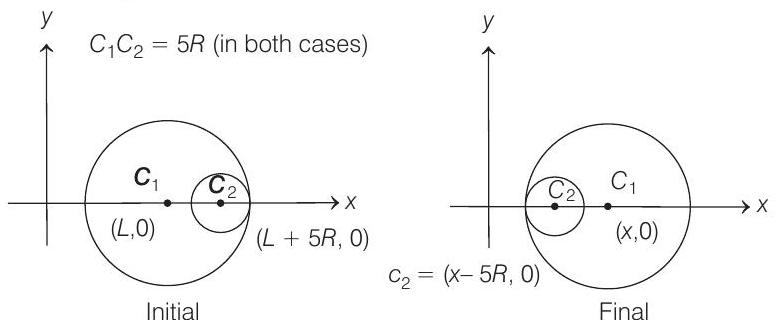
$x$-coordinate of CM initially will be given by
$$ \begin{aligned} & x _i=\frac{m _1 x _1+m _2 x _2}{m _1+m _2} \\ = & \frac{(4 M)(L)+M(L+5 R)}{4 M+M}=(L+R) \end{aligned} $$
Let $(x, 0)$ be the coordinates of the centre of large sphere in final position. Then, $x$-coordinate of CM finally will be
$$ x _f=\frac{(4 M)(x)+M(x-5 R)}{4 M+M}=(x-R) $$
Equating Eqs. (i) and (ii), we have $x=L+2 R$
Therefore, coordinates of large sphere, when the smaller sphere reaches the other extreme position, are $(L+2 R, 0)$.






