Straight Line and Pair of Straight Lines 1 Question 58
58. Straight lines
Show Answer
Solution:
- Let
Then,
Clearly,
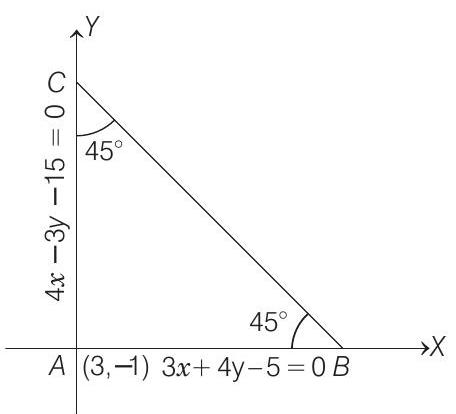
Hence, the line
where,






