Straight Line and Pair of Straight Lines 1 Question 45
45. The orthocentre of the triangle formed by the lines
Show Answer
Solution:
- Let
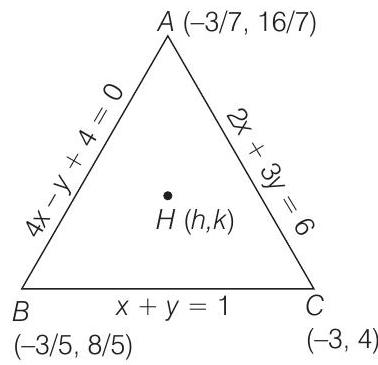
Also,
On solving Eqs. (i) and (ii), we get
Hence, this coordinate lies in the first quadrant.






