Straight Line and Pair of Straight Lines 1 Question 19
19.
Let $k$ be an integer such that the triangle with vertices $(k,-3 k),(5, k)$ and $(-k, 2)$ has area $28$ sq units. Then, the orthocentre of this triangle is at the point
(2017 Main)
(a) $(2,-\frac{1}{2})$
(b) $(1, \frac{3}{4})$
(c) $(1,-\frac{3}{4})$
(d) $(2, \frac{1}{2})$
Show Answer
Answer:
Correct Answer: 19. (d)
Solution:
- Given, vertices of triangle are $(k,-3 k),(5, k)$ and $(-k, 2)$.
$ \begin{array}{lr} \therefore & \frac{1}{2}\left|\begin{array}{ccc} k & -3 k & 1 \\ 5 & k & 1 \\ -k & 2 & 1 \end{array}\right|= \pm 28 \\ \Rightarrow & \left|\begin{array}{ccc} k & -3 k & 1 \\ 5 & k & 1 \\ -k & 2 & 1 \end{array}\right|= \pm 56 \\ \Rightarrow & k(k-2)+3 k(5+k)+1\left(10+k^{2}\right)= \pm 56 \\ \Rightarrow & k^{2}-2 k+15 k+3 k^{2}+10+k^{2}= \pm 56 \\ \Rightarrow & 5 k^{2}+13 k+10= \pm 56 \\ \Rightarrow & 5 k^{2}+13 k-66=0 \\ \text { or } & 5 k^{2}+13 k-46=0 \\ \Rightarrow & k=2 \end{array} $
Thus, the coordinates of vertices of triangle are $A(2,-6), B(5,2)$ and $C(-2,2)$.
Now, equation of altitude from vertex $A$ is
$ y-(-6)=\frac{-1}{(\frac{2-2}{-2-5})}(x-2) \Rightarrow x=2 $ $\quad$ …….(i)
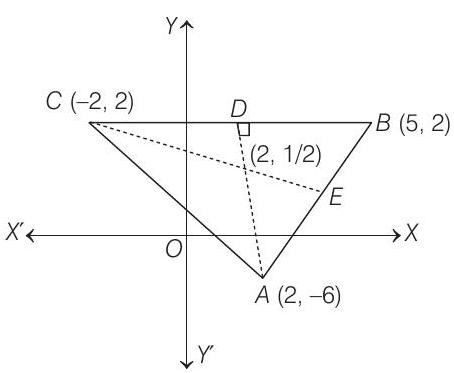
Equation of altitude from vertex $C$ is
$ y-2=\frac{-1}{[\frac{2-(-6)}{5-2}]}[x-(-2)] $
$\Rightarrow \quad 3 x+8 y-10=0$ $\quad$ …….(ii)
On solving Eqs. (i) and (ii), we get $x=2$ and $y=\frac{1}{2}$.
$\therefore$ Orthocentre $=(2, \frac{1}{2})$
