Properties of Triangles 3 Question 15
15. Circle with radii 3,4 and 5 touch each other externally, if $P$ is the point of intersection of tangents to these circles at their points of contact. Find the distance of $P$ from the point of contact.
(2005, 2M)
Show Answer
Answer:
Correct Answer: 15. $\sqrt{5}$
Solution:
- Since, the circles with radii 3,4 and 5 touch each other externally and $P$ is the point of intersection of tangents.
$\Rightarrow P$ is incentre of $\Delta C _1 C _2 C _3$.
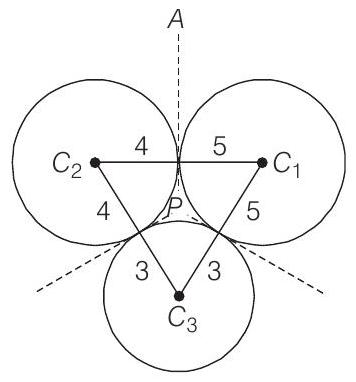
Thus, distance of point $P$ from the points of contact
$$ =\text { inradius }(r) \text { of } \Delta C _1 C _2 C _3 $$
i.e. $\quad r=\frac{\Delta}{s}=\sqrt{\frac{s(s-a)(s-b)(s-c)}{s}}$
where, $2 s=7+8+9 \Rightarrow s=12$
Hence, $r=\sqrt{\frac{(12-7)(12-8)(12-9)}{12}}=\sqrt{\frac{5 \cdot 4 \cdot 3}{12}}=\sqrt{5}$
