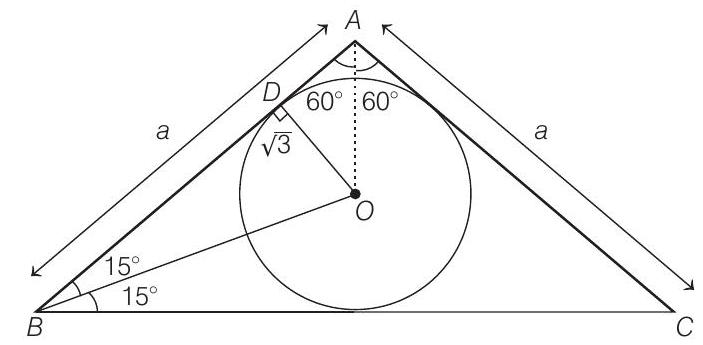
Properties of Triangles 2 Question 3
3. In radius of a circle which is inscribed in a isosceles triangle one of whose angle is $2 \pi / 3$, is $\sqrt{3}$, then area of triangle (in sq units) is
(2006, 2M)
(a) $4 \sqrt{3}$
(b) $12-7 \sqrt{3}$
(c) $12+7 \sqrt{3}$
(d) None of these
Show Answer
Answer:
Correct Answer: 3. (c)
Solution:
- Let $A B=A C=a$ and $\angle A=120^{\circ}$.
$\therefore \quad$ Area of triangle $=\frac{1}{2} a^{2} \sin 120^{\circ}$
where, $\quad a=A D+B D=\sqrt{3} \tan 30^{\circ}+\sqrt{3} \cot 15^{\circ}$
$$ =1+\frac{\sqrt{3}}{\tan \left(45^{\circ}-15^{\circ}\right)} $$
$$ \begin{aligned} \Rightarrow \quad a & =1+\sqrt{3} \frac{1+\tan 45^{\circ} \tan 30^{\circ}}{\tan 45^{\circ}-\tan 30^{\circ}} \\ & =1+\sqrt{3} \frac{\sqrt{3}+1}{\sqrt{3}-1}=4+2 \sqrt{3} \end{aligned} $$
$\therefore$ Area of a triangle
$$ =\frac{1}{2}(4+2 \sqrt{3})^{2} \frac{\sqrt{3}}{2}=(12+7 \sqrt{3}) \text { sq units } $$
