Properties of Triangles 1 Question 3
3. In a triangle, the sum of lengths of two sides is
(2019 Main, 11 Jan I)
(a)
(b)
(c)
(d)
Show Answer
Answer:
Correct Answer: 3. (b)
Solution:
- We know that,
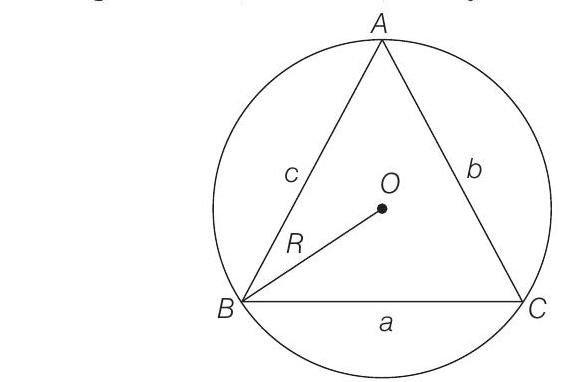
[using cosine rule,
Now,






