SATHEE: Parabola 3 Question 11

Parabola 3 Question 11

11. Find the equation of the normal to the curve $x^{2} = 4 y$ which passes through the point $(1, 2)$ .

(1984, 4M)

Show Answer

Answer:

Correct Answer: 11. $x + y = 3$

Solution:

Let the equation of chord $O P$ be $y = m x$ .

Then, equation of chord will be $y = - \frac{1}{m} x$ and

$P$ is point of intersection of $y = m x$ and $y^{2} = 4 x$ is $\frac{4}{m^{2}}, \frac{4}{m}$ and $Q$ is point intersection of $y = - \frac{1}{m} x$ and $y^{2} = 4 x$ is $(4 m^{2}, - 4 m)$ .

Now, equation of $P Q$ is

$y + 4 m = \frac{\frac{4}{m} + 4 m}{\frac{4}{m^{2}} - 4 m^{2}} (x - 4 m^{2})$

$\begin{array}{lc} \Rightarrow & y + 4 m = \frac{m}{1 - m^{2}} (x - 4 m^{2}) \\ \Rightarrow & (1 - m^{2}) y + 4 m - 4 m^{3} = m x - 4 m^{3} \\ \Rightarrow & m x - (1 - m^{2}) y - 4 m = 0 \end{array}$

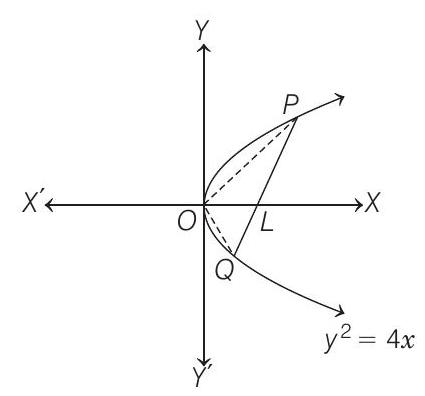
This line meets $X$ -axis, where $y = 0$

i.e. $x = 4 \Rightarrow O L = 4$ which is constant as independent of $m$ .

Again, let $(h, k)$ be the mid-point of $P Q$ . Then,

$\begin{aligned} h = \frac{4 m^{2} + \frac{4}{m^{2}}}{2} \\ and & k & = \frac{\frac{4}{m} - 4 m}{2} \\ \Rightarrow & h = 2 & m^{2} + \frac{1}{m^{2}} \\ and & k & = 2 \frac{1}{m} - m \\ \Rightarrow & h & = 2 & m - {\frac{1}{m}}^{2} + 2 \\ and & k & = 2 \frac{1}{m} - m \end{aligned}$

Eliminating $m$ , we get

$2 h = k^{2} + 8$

or $y^{2} = 2 (x - 4)$ is required equation of locus.

Parabola 3 Question 11

11. Find the equation of the normal to the curve $x^{2} = 4 y$ which passes through the point $(1, 2)$ .

Answer:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Parabola 3 Question 11

11. Find the equation of the normal to the curve x2=4y which passes through the point (1,2).

Answer:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu

11. Find the equation of the normal to the curve $x^{2} = 4 y$ which passes through the point $(1, 2)$ .